A circular disc of radius $\frac{R}{4}$ is cut from a uniform circular disc of radius R.
The centre of cut portion is at a distance of $\frac{R}{2}$ from the centre of the disc from which it is removed. Calculate the centre of mass of remaining portion of the disc.

Video Solution

Text Solution

Generated By DoubtnutGPT

The correct Answer is: $\frac{R}{60}$

To find the center of mass of the remaining portion of the disc after a smaller disc has been cut out, we can follow these steps:
Step 1: Define the System
We have a larger disc of radius $R$ and a smaller disc of radius $\frac{R}{4}$ that is cut out from the larger disc. The center of the smaller disc is at a distance of $\frac{R}{2}$ from the center of the larger disc.
Step 2: Calculate the Masses
Assuming the mass per unit area of the larger disc is uniform, we can denote:
- The mass of the larger disc $M$ .
- The area of the larger disc $A_{1} = π R^{2}$ .
- The area of the smaller disc $A_{2} = π {(\frac{R}{4})}^{2} = \frac{π R^{2}}{16}$ .
The mass of the smaller disc $M_{1}$ can be calculated as:
$M_{1} = (mass per unit area) \times A_{2} = \frac{M}{A_{1}} \times A_{2} = \frac{M}{π R^{2}} \times \frac{π R^{2}}{16} = \frac{M}{16}$
The mass of the remaining portion $M_{2}$ is:
$M_{2} = M - M_{1} = M - \frac{M}{16} = \frac{15 M}{16}$
Step 3: Determine the Position Vectors
- The center of the larger disc (original disc) is at the origin, $(0, 0)$ .
- The center of the smaller disc, which is cut out, is at $(0, \frac{R}{2})$ .
Step 4: Calculate the Center of Mass of the Remaining Portion
The center of mass $X_{c m}$ of the remaining portion can be calculated using the formula:
$X_{c m} = \frac{M_{2} \cdot X_{2} - M_{1} \cdot X_{1}}{M_{2} - M_{1}}$
Where:
- $X_{1}$ is the position of the center of mass of the smaller disc, which is $\frac{R}{2}$ .
- $X_{2}$ is the position of the center of mass of the larger disc, which is $0$ .
Substituting the values:
$X_{c m} = \frac{(\frac{15 M}{16}) \cdot 0 - (\frac{M}{16}) \cdot (\frac{R}{2})}{\frac{15 M}{16} - \frac{M}{16}}$
$= \frac{- \frac{M R}{32}}{\frac{14 M}{16}}$
$= \frac{- \frac{R}{32}}{\frac{14}{16}} = - \frac{R}{32} \cdot \frac{16}{14} = - \frac{R}{28}$
Step 5: Conclusion
Thus, the center of mass of the remaining portion of the disc is located at:
$Center of Mass = - \frac{R}{28} along the y-axis$

|

Updated on:7/8/2024

Class 11 PHYSICS RIGID BODY DYNAMICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A circular disc of radius $20 c m$ is cut from one edge of a larger circular disc of radius $50 c m$ . The shift of centre of mass is
A $5.7 c m$
B $- 5.7 c m$
C $3.2 c m$
D $- 3.2 c m$
Question 2 - Select One
A uniform circular disc of radius a is taken. A circular portion of radius b has been removed from it as shown in the figure. If the center of hole is at a distance c from the center of the disc, the distance $x_{2}$ of the center of mass of the remaining part from the initial center of mass O is given by

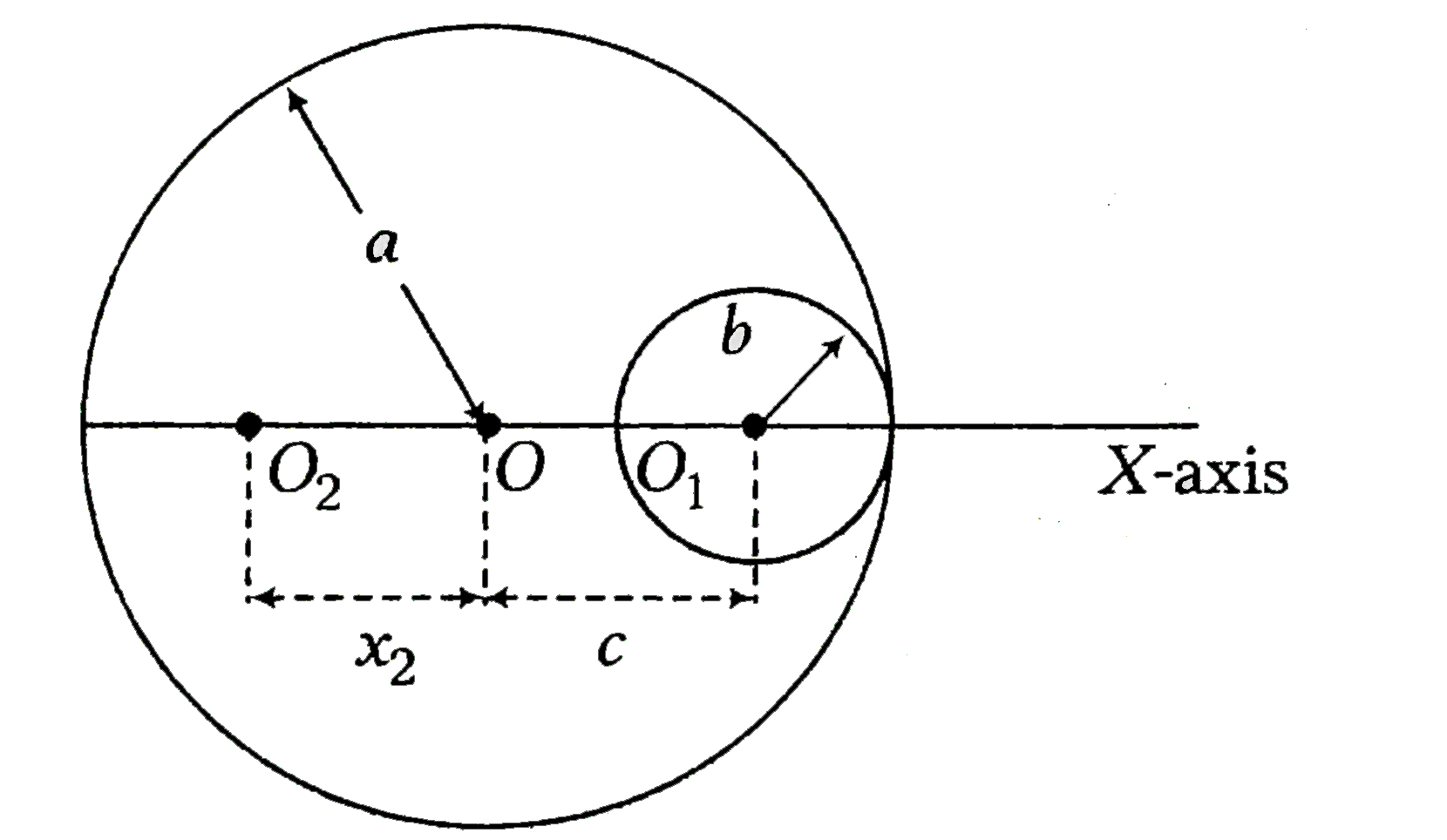A $\frac{π b^{2}}{(a^{2} - b^{2})}$
B $\frac{- c b^{2}}{(a^{2} - b^{2})}$
C $\frac{π c^{2}}{(a^{2} - b^{2})}$
D $\frac{π a^{2}}{(c^{2} - b^{2})}$
Question 3 - Select One
A circular disc of radius R is removed from a bigger circular disc of radius 2R, such that the circumference of the discs coincide. The centre of the bigger disc. The value of $α$ is
A $\frac{1}{3}$
B $\frac{1}{2}$
C $\frac{1}{6}$
D $\frac{1}{4}$

MODERN PUBLICATION-SYSTEMS OF PARTICLES AND ROTATIONAL MOTION-PRACTICE PROBLEMS

Home
Ask Doubt
Profile