Tangents drawn from P(1,8) to the circle x2+y2−6x−4y−11=0 touches the circle at the points A and B, respectively. The radius of the circle which passes through the points of intersection of circles x2+y2−2x−6y+6=0andx2+y2−2x−6y+6=0 the circumcircle of the and interse ΔPAB orthogonally is equal to
√734
√712
3
2
The correct Answer is:A
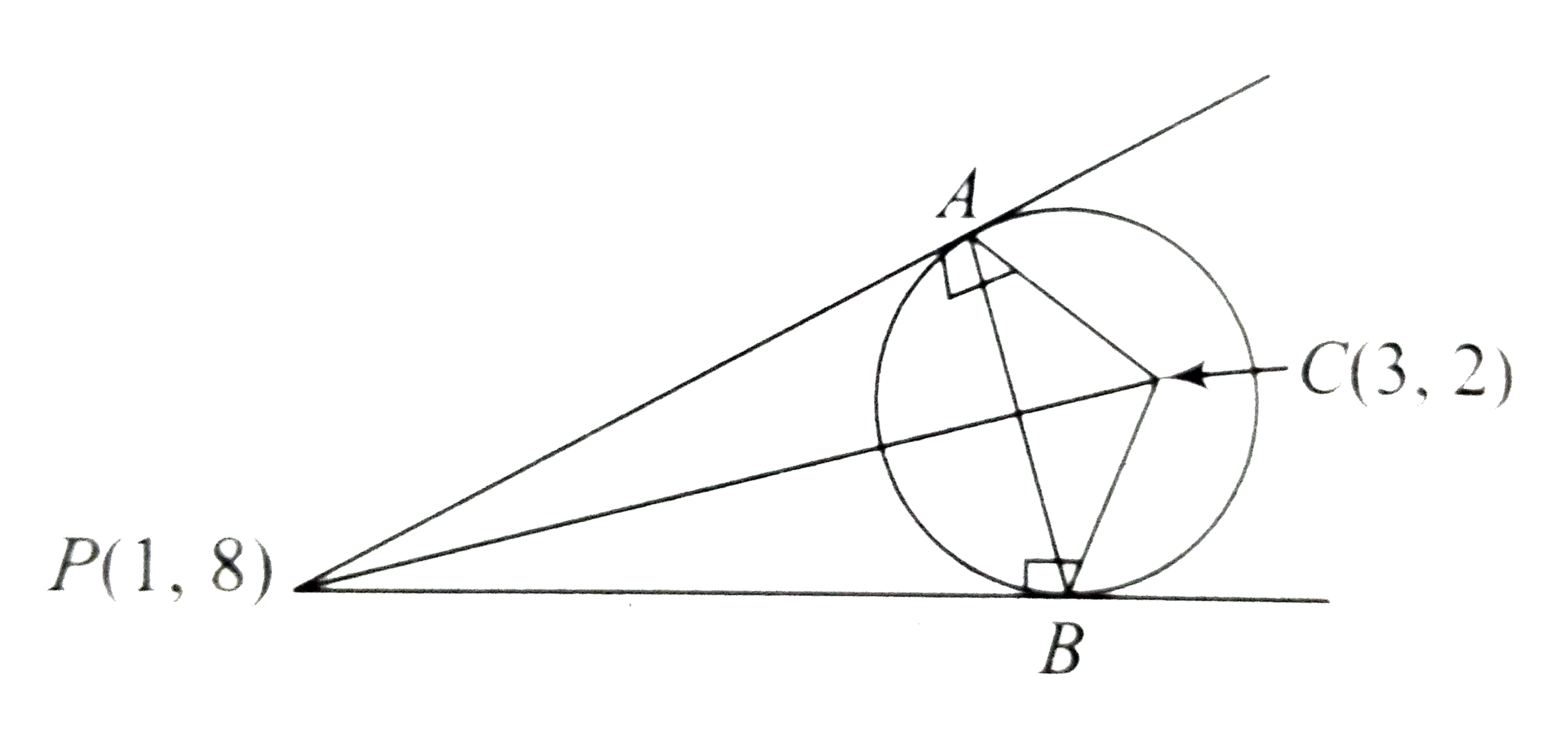
Equation of circle circumscribing ΔPAB is:
(x−1)(x−3)+(y−8)(y−2)=0
⇒x2+y2−4x−10y+19=0 (i)
Equation of circle passing through points of intersection of circles x2+y2−2x−6y+6=0 and x2+y2+2x−6y+6=0 is given by
(x2+y2−2x−6y+6)+λ(x2+y2+2x−6y+6)=0
⇒x2+y2+(2λ−2)λ+1x−6y+6=0 (ii)
As circle (ii) is orthogonal to circle (i), we have
−2(2λ−2λ+1)−5(−6)=19+6
⇒4λ−4=5λ+5
⇒λ=−9
Hence, required equation of circle is:
x2+y2+52x−6y+6=0
∴ Radius of circle =√2516+9−6=√734
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Question Bank32videos
CIRCLES
- BOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Comprehension Type8videos
CIRCLES
- BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - JEE Advanced (Single Correct Answer Type)14videos
CIRCLE
- BOOK - CENGAGECHAPTER - COMPLEX NUMBERSEXERCISE - Question Bank30videos
COMPLEX NUMBERS
Similar Questions
The equation of the circle which passes through the origin and cuts orthogonally each of the circles x2+y2−6x+8=0 and x2+y2−2x−2y−7=0 is
View SolutionFind the equation of the circle passing through the point of intersection of the circles x2+y2−6x+2y+4=0,x2+y2+2x−4y−6=0 and with its centre on the line y=x.
View Solution
Knowledge Check
- Question 1 - Select One
Tangents drawn from the point P(1,8) to the circle x2+y2−6x−4y−11=0 touch the circle at the points A and B the equation of the circumcircle of △PAB is
Ax2+y2÷4x−6y+19=0Bx2+y2−4x−10y+19=0Cx2+y2−2x+6y−29=0Dx2+y2−6x−4y+19=0 - Question 2 - Select One
Tangents drawn from the point P(1,8) to the circle x2+y2−6x−4y−11=0 touch the circle at the points A and B. The equation of the circumcircle of the triangle PAB is:
Ax2+y2+4x−6y+19=0Bx2+y2−4x−10y+19=0Cx2+y2−2x+6y−20Dx2+y2−6x−4y+19=0 - Question 3 - Select One
Tangents drawn from the point P(1,8) to the circle x2+y2−6x−4y−11=0 touch the circle at the points A and B. The equation of the circumcircle of the triangle PAB is:
Ax2+y2+4x−6y+19=0Bx2+y2−4x−10y+19=0Cx2+y2−2x+6y−20Dx2+y2−6x−4y+19=0
The equation of the circle which pass through the origin and cuts orthogonally each of the circles x2+y2−6x+8=0 and x2+y2−2x−2y=7 is
View SolutionTangents drawn from the point P(1,8) to the circle x2+y2−6x−4y−11=0 touch the circle at the points A and B. The equation of the circumcircle of the triangle PAB is:
View SolutionTangents drawn from the point P (1,8) to the circle x2+y2−6x−4y−11=0 touch the circle at the points A and B. The equation of the circumcircle of the triangle PAB is
View SolutionThe equation of the circle which passes through the point (1,1) and through the points of intersection of the circles x2+y2=6 and x2+y2−6y+8=0 is
View SolutionThe equation of the circle which passes through the point (1, 1) and cuts the circles x2+y2−8x−2y+16=0 and x2+y2−4x−4y−1=0 orthogonally is
View Solution

