ABCD is a square Points E(4,3) and F(2,5) lie on AB and CD, respectively,such that EF divides the square in two equal parts. If the coordinates of A are (7,3),then the coordinates of other vertices can be
(7,2)
(7,5)
(−1,3)
(−1,5)
The correct Answer is:D
Since EF divides the squre in two equal parts, it must pass through the center of the square and center is midpoint of EF. So, center of the sqaure is (3,4).
Topper's Solved these Questions
- COORDINATE SYSYEM BOOK - CENGAGECHAPTER - COORDINATE SYSYEM EXERCISE - Exercise (Multiple)
13 Videos
- COORDINATE SYSYEM BOOK - CENGAGECHAPTER - COORDINATE SYSYEM EXERCISE - Exercise (Comprehension)
10 Videos
- COORDINATE SYSYEM BOOK - CENGAGECHAPTER - COORDINATE SYSYEM EXERCISE - Exercise 1.6
9 Videos
- COORDINATE SYSTEMBOOK - CENGAGECHAPTER - COORDINATE SYSTEMEXERCISE - Multiple Correct Answers Type
2 Videos
- CROSS PRODUCTS BOOK - CENGAGECHAPTER - CROSS PRODUCTS EXERCISE - DPP 2.2
13 Videos
Similar Questions
Knowledge Check
ABCD is a trapezium , in which AD∣∣BC, E and F are the mid-point of AB and CD respectively , then EF is :
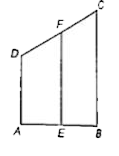 A(AD+BC)2B(AB+CD)2C(DF xx CF)/(AE xx BE)DAD+EF+BC2
A(AD+BC)2B(AB+CD)2C(DF xx CF)/(AE xx BE)DAD+EF+BC2
The two opposite vertices of a square are (−1,2) and (3,2). Find the coordinates of the other two vertices.
View SolutionThe center of a square is at the origin and its one vertex is A(2,1). Find the coordinates of the other vertices of the square.
View SolutionABCD is a trapezium, in which AD||BC, E and F are the mid-points of AB and CD respectively, then EF is:

View SolutionThe opposite vertices of a square are (2, 6) and (0, -2). Find the coordinates of the other vertices.
View SolutionIf A(3, 4) and C (1, -1) are two opposite angular points of square ABCD, find the coordinates of other two vertices.
View SolutionQ.7 The two opposite vertices of a square are (l.-6)and (5,4). Find the coordinates of the other two vertices.
View SolutionQ.7 The two opposite vertices of a square are (l.-6)and (5,4). Find the coordinates of the other two vertices.
View Solution
