A rectangular billiard table has vertices at P(0,0),Q(0,7),R(10,7), and S(10,0). A small billiard ball starts at M(3,4) , moves in a straight line to the top of the table, bounces to the right side of the table, and then comes to rest at N(7,1) . The y− coordinate of the point where it hits the right side is 3.7 (b) 3.8 (c) 3.9 (d) 4
3.7
3.8
3.9
4
The correct Answer is:A
tanθ=a−13=7−a10−b
Also, tanθ=7−4b−3=3b−3
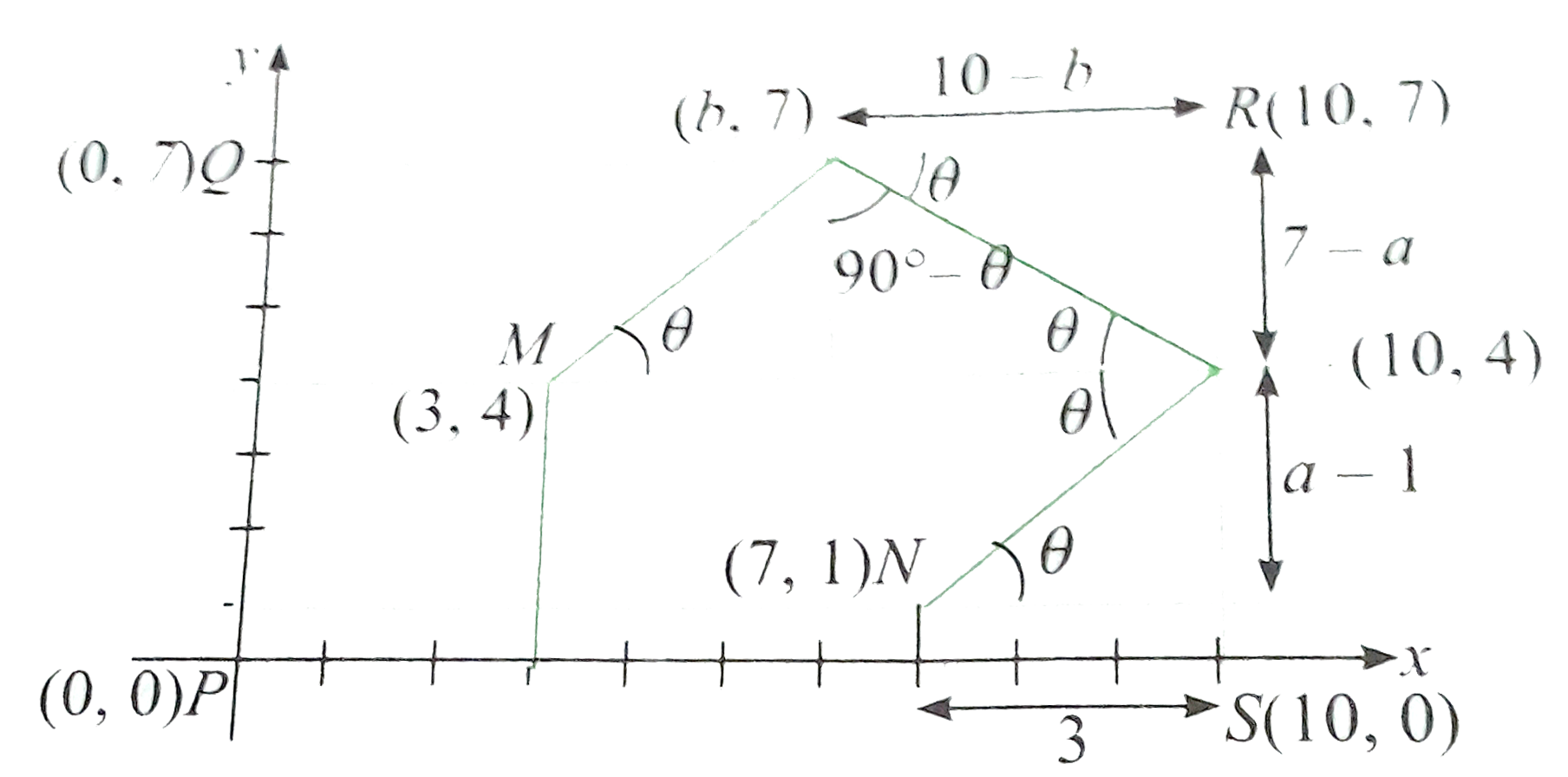
Hence, 3b−3=a−13=7−a10−b
From the first two relations, we have
9=ab−b−3a+3
or 3a+6=ab−b
From the last two relations, we have
10a−ab−10+b=21−3a
or 3a−ab+b=31
or ab−b=13a−31
Hence, from (1) and (2) ,
3a+6=13a−31
or 10a−37
or a=3.7
Topper's Solved these Questions
- COORDINATE SYSYEM Book:CENGAGEChapter:COORDINATE SYSYEM Exercise:Exercise (Multiple)
Explore 13 Videos
- COORDINATE SYSYEM Book:CENGAGEChapter:COORDINATE SYSYEM Exercise:Exercise (Comprehension)
Explore 10 Videos
- COORDINATE SYSYEM Book:CENGAGEChapter:COORDINATE SYSYEM Exercise:Exercise 1.6
Explore 9 Videos
- COORDINATE SYSTEMBook:CENGAGEChapter:COORDINATE SYSTEMExercise:Multiple Correct Answers Type
Explore 2 Videos
- CROSS PRODUCTS Book:CENGAGEChapter:CROSS PRODUCTS Exercise:DPP 2.2
Explore 13 Videos
Similar Questions
A rectangular billiard table has vertices at P(0,0),Q(0,7),R(10,7), and S(10,0). A small billiard ball starts at M(3,4) , moves in a straight line to the top of the table, bounces to the right side of the table, and then comes to rest at N(7,1) . The y− coordinate of the point where it hits the right side is (a)3.7 (b) 3.8 (c)3.9(d) 4
View SolutionThe quadrilateral whose consecutive vertices are A(0,0),B(3,4),C(7,7)&D(4,3) is
View Solution
Knowledge Check
The vertices of a right angled triangle right angle at P are P (3,4) , Q(7,4) and R (3,8). Then, what is the coordinates of orthocentre of ΔPQR ?
A(3,4)B(7,4)C(3,8)D(5,6)
The coordinates of the point of intersection of the lines 3x+4y−18=0 and 4x−5y+7=0 are
View SolutionFind the point where the straight lines 7x−8y−3=0and10y−9x+5=0 intersect.
View SolutionA man starts from the point P(−3,4) and will reach the point Q(0,1) touching the line 2x+y=7 at R. The coordinates R on the line so that he will travel in the shortest distance is
View SolutionIf the image of the point (3,8) in the line px+3y−7=0 is the point (1,−4), then find the value of p.
View SolutionThe quadrilateral whose consecutive vertices are A(0,0), B(3,4),C(7,7) & D(4,3) is a parallelogram only a rectangle a square a rhombus
View SolutionIf a circle having the point (−1,1) as its centre touches the straight line x+2y+9=0, then the coordinates of the point(s) of contact are : (A) (73,−173) (B) (−3,−3) (C) (−3,3) (D) (0,0)
View SolutionA line passes through (−3,4) and the portion of the line intercepted between the coordinate axes is bisected at the point then equation of line is (A) 4x−3y+24=0 (B) x−y−7=0 (C) 3x−4y+25=0 (D) 3x−4y+24=0
View Solution