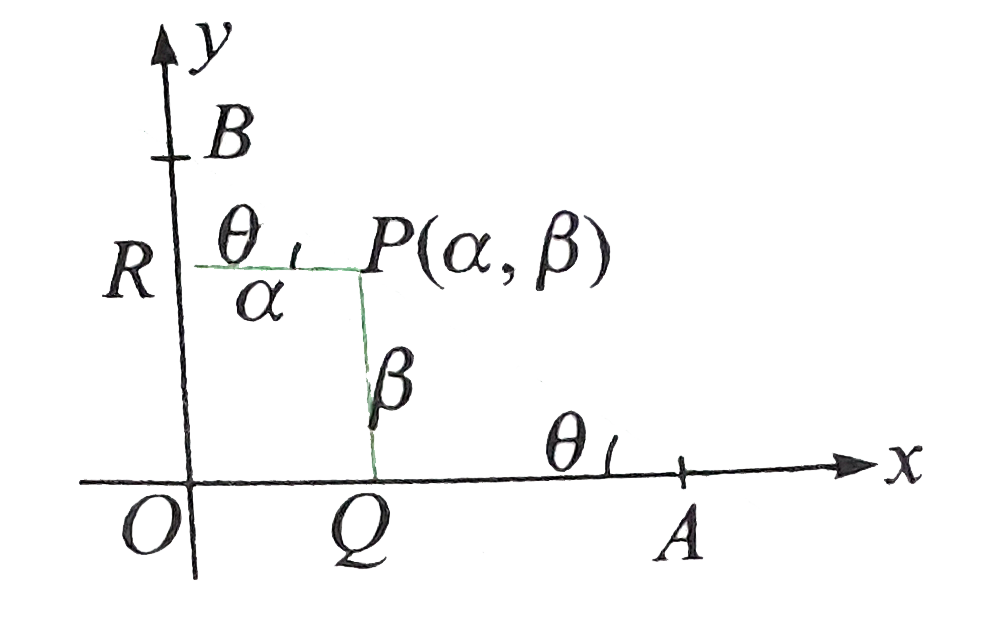
Through the point $P (α, β)$ , where $α β > 0,$ the straight line $\frac{x}{a} + \frac{y}{b} = 1$ is drawn so as to form a triangle of area $S$ with the axes. If $a b > 0,$ then the least value of $S$ is $α β$ (b) $2 α β$ (c) $3 α β$ (d) none

$α β$

$2 α β$

$3 α β$

none

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Updated on:21/07/2023

If $α, β$ be the roots of the quadratic equation $3 x^{2} - 6 x + 4 = 0$ , find the value of
$(\frac{α}{β} + \frac{β}{α}) + 2 (\frac{1}{α} + \frac{1}{β}) + 3 α β$ :
A6
B8
C7
D5
If $α and β$ are the roots of $2 x^{2} - 6 x + 3 = 0$ , then the value of $(\frac{α}{β} + \frac{β}{α}) + 3 (\frac{1}{α} + \frac{1}{β}) + 2 α β$ is
A13
B4
C6
D3
If $α and β$ are the roots of the equation $3 x^{2} + 7 x + 3 = 0$ Find the value of $α β :$
A1
B0
C2
D3