If a straight line through the origin bisects the line passing through the given points (acosα,asinα) and (acosβ,asinβ), then the lines are perpendicular are parallel have an angle between them of π4 none of these
A
are perpendicular
B
are parallel
C
have an angle between them of π/4
D
none of these
Video Solution
Text Solution
The correct Answer is:A
Since OA=OB=a,AOB is an isosceles triangle.
The line through the origin bisecting AB will be perpendicular to AB.
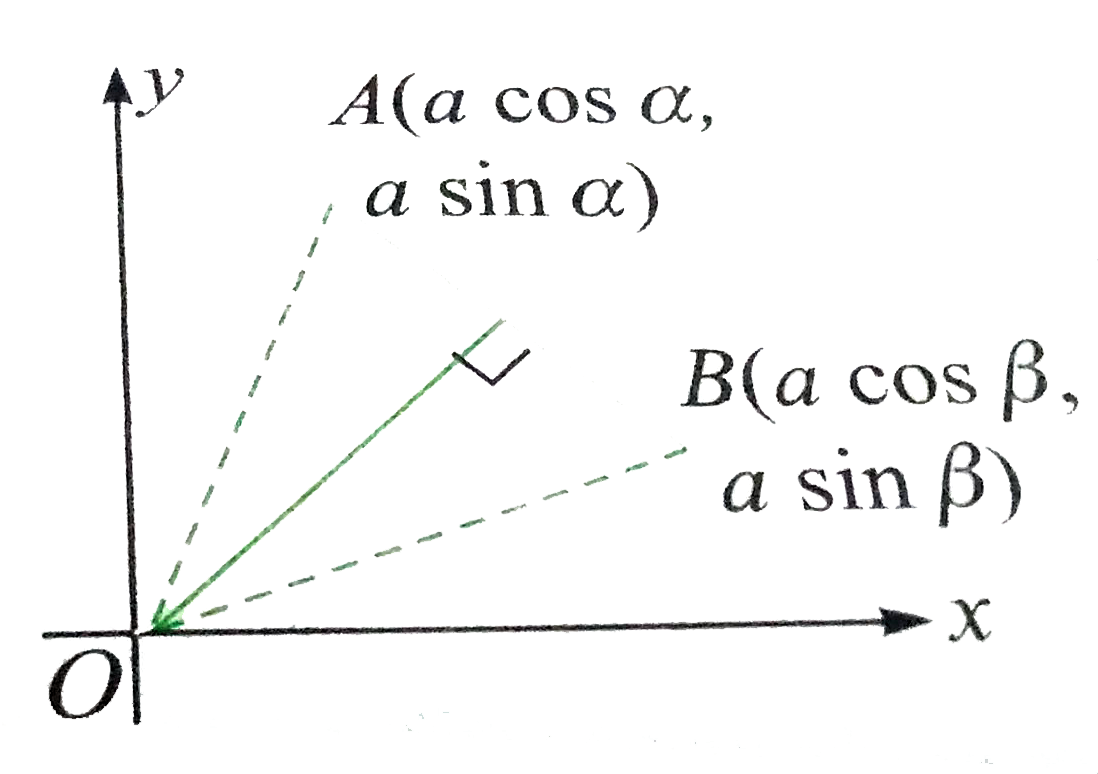
|
Topper's Solved these Questions
- COORDINATE SYSYEM Book:CENGAGEChapter:COORDINATE SYSYEM Exercise:Exercise (Multiple)
Explore 13 Videos
- COORDINATE SYSYEM Book:CENGAGEChapter:COORDINATE SYSYEM Exercise:Exercise (Comprehension)
Explore 10 Videos
- COORDINATE SYSYEM Book:CENGAGEChapter:COORDINATE SYSYEM Exercise:Exercise 1.6
Explore 9 Videos
- COORDINATE SYSTEMBook:CENGAGEChapter:COORDINATE SYSTEMExercise:Multiple Correct Answers Type
Explore 2 Videos
- CROSS PRODUCTS Book:CENGAGEChapter:CROSS PRODUCTS Exercise:DPP 2.2
Explore 13 Videos
Similar Questions
Knowledge Check
Equation of straight line passing through origin
AY=mx,BY=CCX= myDX=CWhich of the following straight lines passes through the origin?
Ax + y = 4Bx2+y2=−6Cx + y =5Dx =4yThe lines parallel to the axes and passing through the point (4, -5) are
Ax=−5,y=4Bx=5,y=−4Cx=4,y=−5Dx=−4,y=5
Equation of line passing through a given point and parallel to given line
View SolutionEquation of line passing through a given point and perpendicular to given line
View SolutionThe slope of a line passing through origin and a point (3,4) is.......
View SolutionLine passing through a point and making a given angle with a line .
View SolutionThe equation of the line passing through the point (-3,1) and bisecting the angle between coordinate axes is
View Solution