Consider the point A=(3,4),B(7,13). If 'P' be a point on the line y=x such that PA+PB is minimum then coordinates of P is (A) (137,13,7) (B) (237,237) (C) (317,317) (D) (337,337)
(12/7,12/7)
(-24/5,17/5)
(31/7,31/7)
(0,0)
The correct Answer is:C
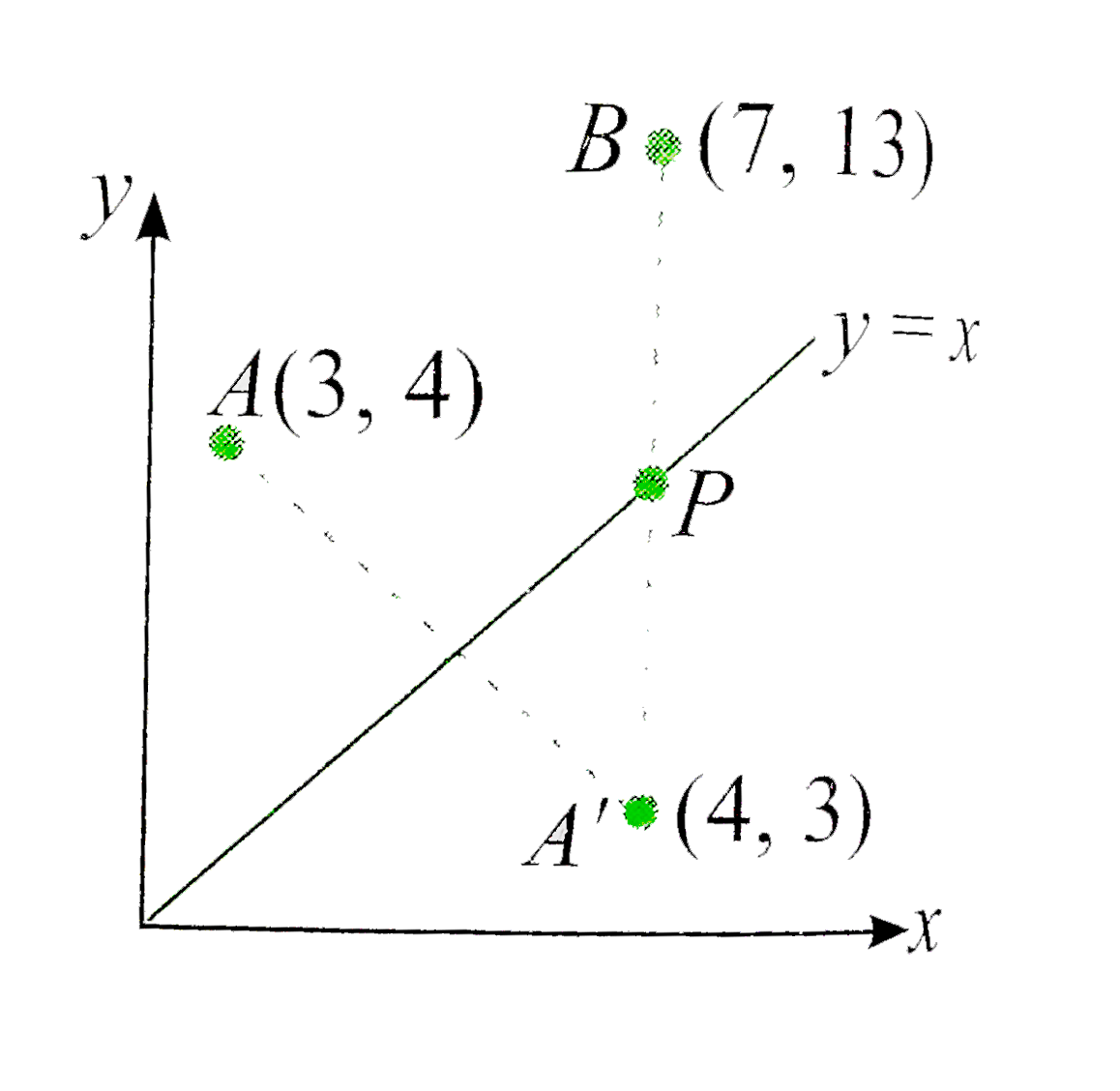
Consider a point A', the image of A in y=x. Therefore, the coordinates of A' are (4,3).
Notice that A and B lie on the same side with respect to y=x. Then PA=PA' . Thus, PA+PB is minimum. If PA'+PB is minimum, i.e., if P, A', B are collinear. Now, the equation of A'B is
y−3=13−37−4(x−4)
or 3y-10x+31=0
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise (Multiple)30videos
STRAIGHT LINES
- BOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise (Comprehension)27videos
STRAIGHT LINES
- BOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise 2.65videos
STRAIGHT LINES
- BOOK - CENGAGECHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type8videos
STRAIGHT LINE
- BOOK - CENGAGECHAPTER - THEORY OF EQUATIONSEXERCISE - JEE Advanced Previous Year9videos
THEORY OF EQUATIONS
Similar Questions
Consider the points A(0,1)andB(2,0),andP be a point on the line 4x+3y+9=0 . The coordinates of P such that |PA−PB| is maximum are (−245,175) (b) (−845,135) (317,317) (d) (0,0)
View SolutionA point on the line x−11=y−22=z+13 at a distance √6 from the origin is (A) (−57,−107,137) (B) (57,107,−137) (C) (1,2,−1) (D) (−1,−2,1)
View Solution
Knowledge Check
- Question 1 - Select One
If A(3,1)andB(−5,7) are any two given points, If P is a point one the line y=x such that PA+PB is minimum then P is
A(1,1)B(11/5,11/5)C(7/13),7/13)Dnone of these - Question 2 - Select One
The points A (2, 3), B(3, 5), C (7, 7) and D(4, 7) are such that
AABCD is a parallelogramBA, B, C and D are collinearCD lies inside the triangle ABCDD lies on the boundary of the triangle ABC - Question 3 - Select One
3, 7, 13, 21, 31, ….
A40B41C42D43
The reflection of the point (2,−1,3) in the plane 3x−2y−z=9 is (A) (287,157,177) (B) (267,−157,177) (C) (157,26,−177) (D) (267,177,−1570)
View SolutionThe coordinates of a point (3,-7,5) in the new system when the origin is shifted to (-4,3,5) is
(A) (-7,10,4) (B) (7,-10,-4) (C) (7,-10,0) (D) (-7,-10,-4)View SolutionIf (2,3) are the coordinates of a point P in the new system when the origin is shifted to (−3,7) then the original coordinates of P are
View SolutionFind the ratio in which the straight line 3x – 4y = 7 divides the join of (2,–7) and (–1,3) .
View SolutionThe position vector of the point, which divides the line segment joining the points (1,3,−9) and (6,−2,−4) in the ratio 2:3 is
A) (3,−1,−7)
B) (−3,1,−7)
C) (3,1,−7)
D) (−3,−1,7)View Solution

