The straight lines $7 x - 2 y + 10 = 0$ and $7 x + 2 y - 10 = 0$ form an isosceles triangle with the line $y = 2 .$ The area of this triangle is equal to

A

15/7 sq. units

B

10/7 sq. units

C

18/7 sq. units

D

none of these

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

We have
$B \equiv (\frac{6}{7}, 2), C \equiv (- \frac{6}{7}, 2)$
$or B C = \frac{12}{7}, A D = 3$

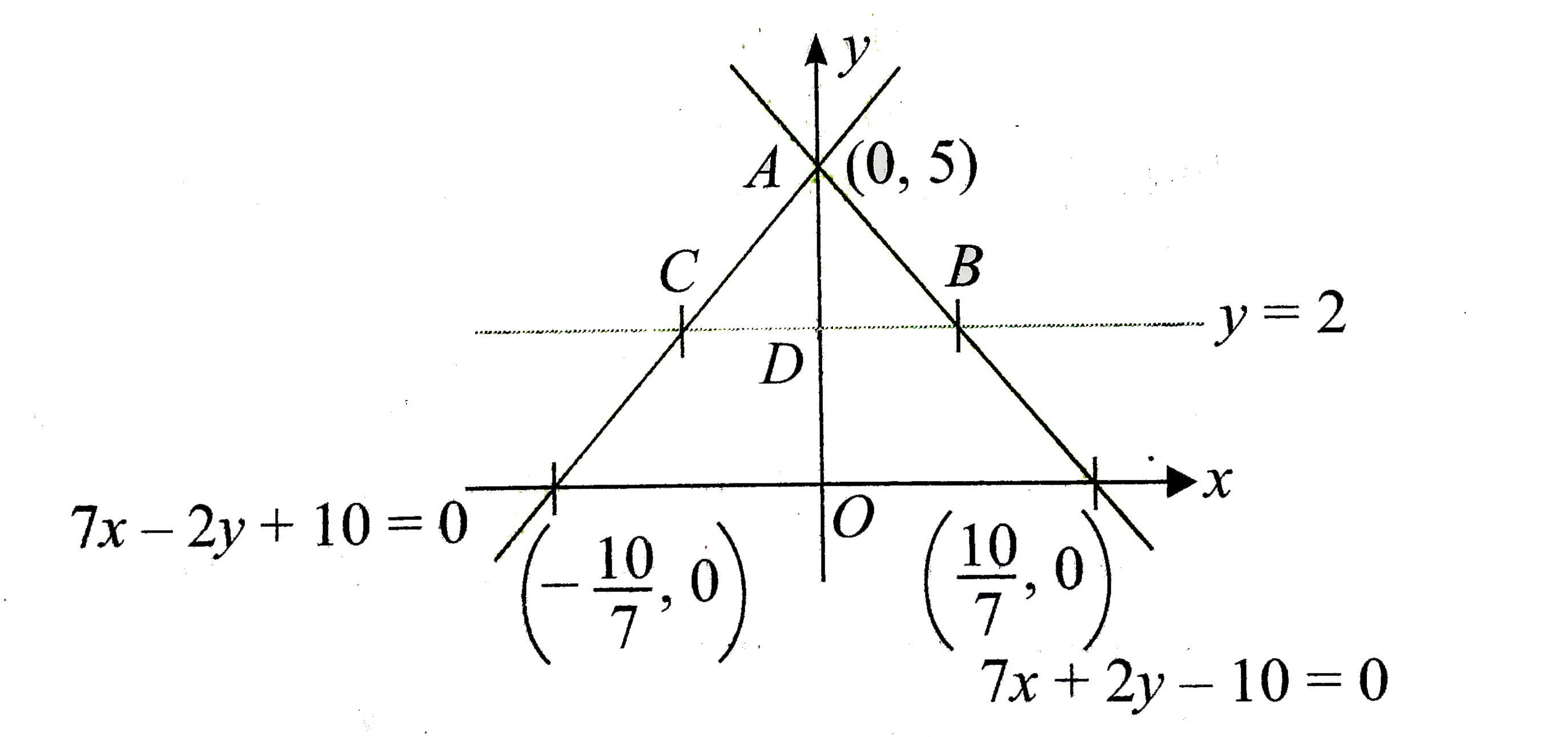
$∴ Δ_{A B C} = \frac{1}{2} \times \frac{12}{7} \times 3 = \frac{18}{7}$ sq. units

|

Updated on:21/07/2023

Class 12 MATHS STRAIGHT LINES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
If the lines $k x^{2} + 6 x y + 2 y^{2} = 0 and x + 3 y = 9$ form an isosceles triangle, then k=
A $- 8$
B $8$
C $6$
D $- 6$
Question 2 - Select One
Drawn from origin are two mutually perpendicular lines forming an isosceles triangle together with the straight line $2 x + y = a$ then the area of this triangle is
A $\frac{a^{2}}{2}$ sq units
B $\frac{a^{2}}{3}$ sq units
C $\frac{a^{2}}{5}$ sq units
DNone of these
Question 3 - Select One
The straight lines $4 x - 3 y - 5 = 0, x - 2 y = 0, 7 x + y - 40 = 0$ and $x + 3 y + 10 = 0$ from
Aa rectangle
Ba parallelogram
Ca cyclic quadrilateral
Dnone of these

CENGAGE-STRAIGHT LINES-Exercise (Single)

Home
Ask Doubt
Profile