The lines y=m1x,y=m2x,andy=m3x make equal in- tercepts on the line x+y=1. then
2(1+m1)(1+m3)=(1+m2)(2+m1+m3)
(1+m1)(1+m3)=(1+m2)(1+m1+m3)
(1+m1)(1+m2)=(1+m3)(2+m1+m3)
2(1+m1)(1+m3)=(1+m2)(1+m1+m3)
The correct Answer is:A
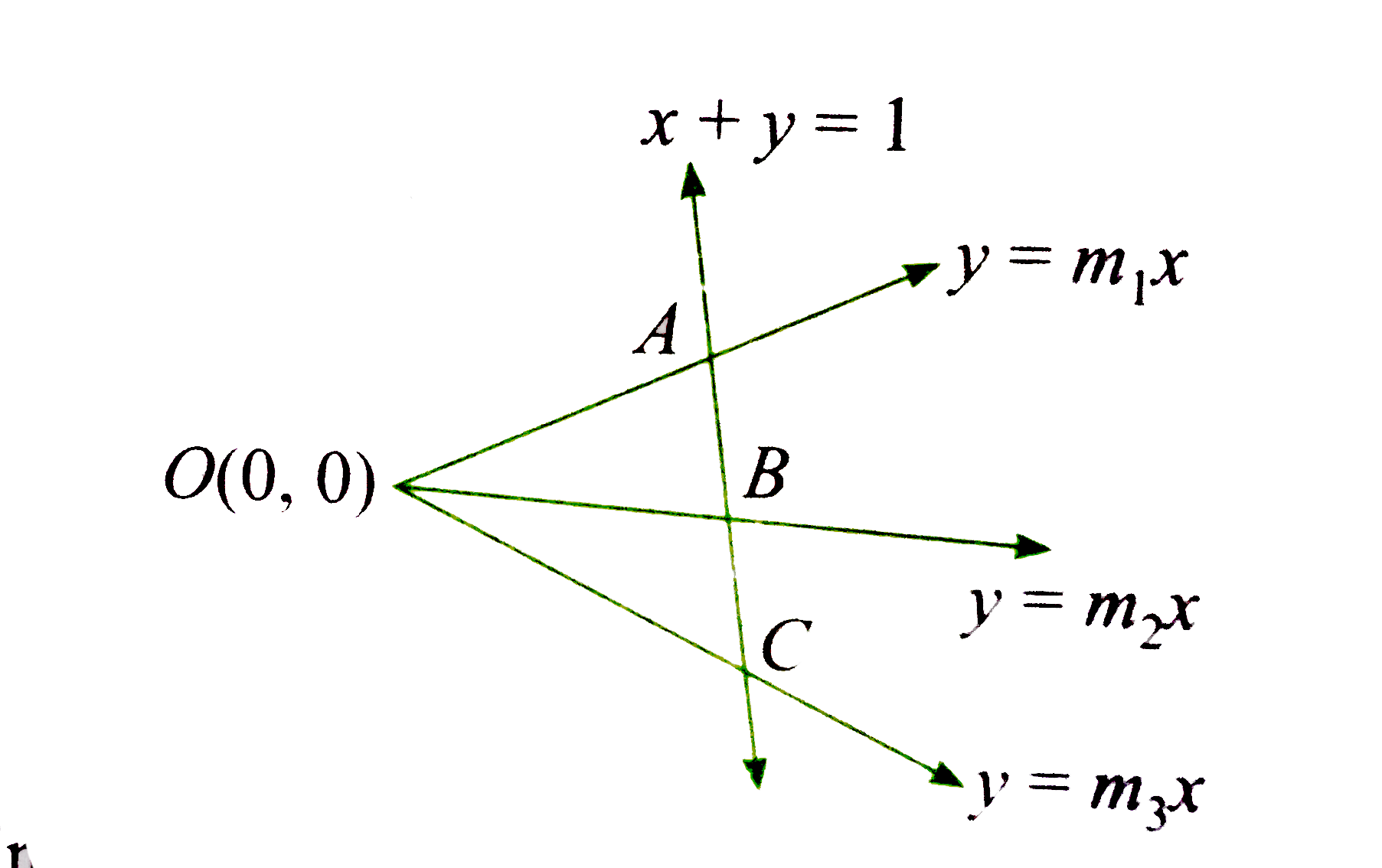
Solving the equations of the lines, we get
A≡(11+m1,m11+m1),C≡(11+m3,m31+m3)
If AB=BC, then the midpoint of AC lies on
y=m2x
or m11+m1+m31+m32=m2⎡⎢⎣11+m1+11+m32⎤⎥⎦
Topper's Solved these Questions
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise (Multiple)
30 Videos
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise (Comprehension)
27 Videos
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise 2.6
5 Videos
- STRAIGHT LINEBOOK - CENGAGECHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type
8 Videos
- THEORY OF EQUATIONSBOOK - CENGAGECHAPTER - THEORY OF EQUATIONSEXERCISE - JEE Advanced Previous Year
9 Videos
Similar Questions
Knowledge Check
If the transversal y=mrx:r=1,2,3 cut off equal intercepts on the transversal x+y=1 then 1+m1,1+m2,1+m3 are in
AA.P.BG.P.CH.P.DNone of theseIf the lines y=x+3andy=3x+1 are equally inclined to the line y=mx+4 then the value of m is
A1+5√22B5√2−17C1−√52DNone of theseIf the lines y=3x+1 and 2y=x+3 are equally inclined to the line y=mx+4, (12<m<3), then the values of m is
A17(1±5√3)B17(1±5√5)C17(1±5√2)D17(1±2√5)
(i) Find the value of 'a' if the lines 3x−2y+8=0, 2x+y+3=0 and ax+3y+11=0 are concurrent.
(ii) If the lines y=m1x+c1, y=m2x+c2 and y=m3x+c3 meet at point then shown that :
c1(m2−m3)+c2(m3−m1)+c3(m1−m2)=0
View SolutionIf the linesy−3x+1and2y−x+3 are equally inclined to the line y−mx+4,(12<m<3),then the values of m is :
View SolutionIf the lines y=3x+1and 2y=x+3are equally inclined to the liney=mx+4, find the value of m.
View SolutionIf the line (x−y+1)+k(y−2x+4)=0 makes equal intercept on the axes then the value of k is
View SolutionIf x1,x2,x3 are the abcissa of the points A1,A2,A3 respectively where the lines y=m1x,y=m2x,y=m3 x meet the line 2x−y+3=0 such that m1,m2,m3 are in A.P., then x1,x2,x3 are in
View Solution