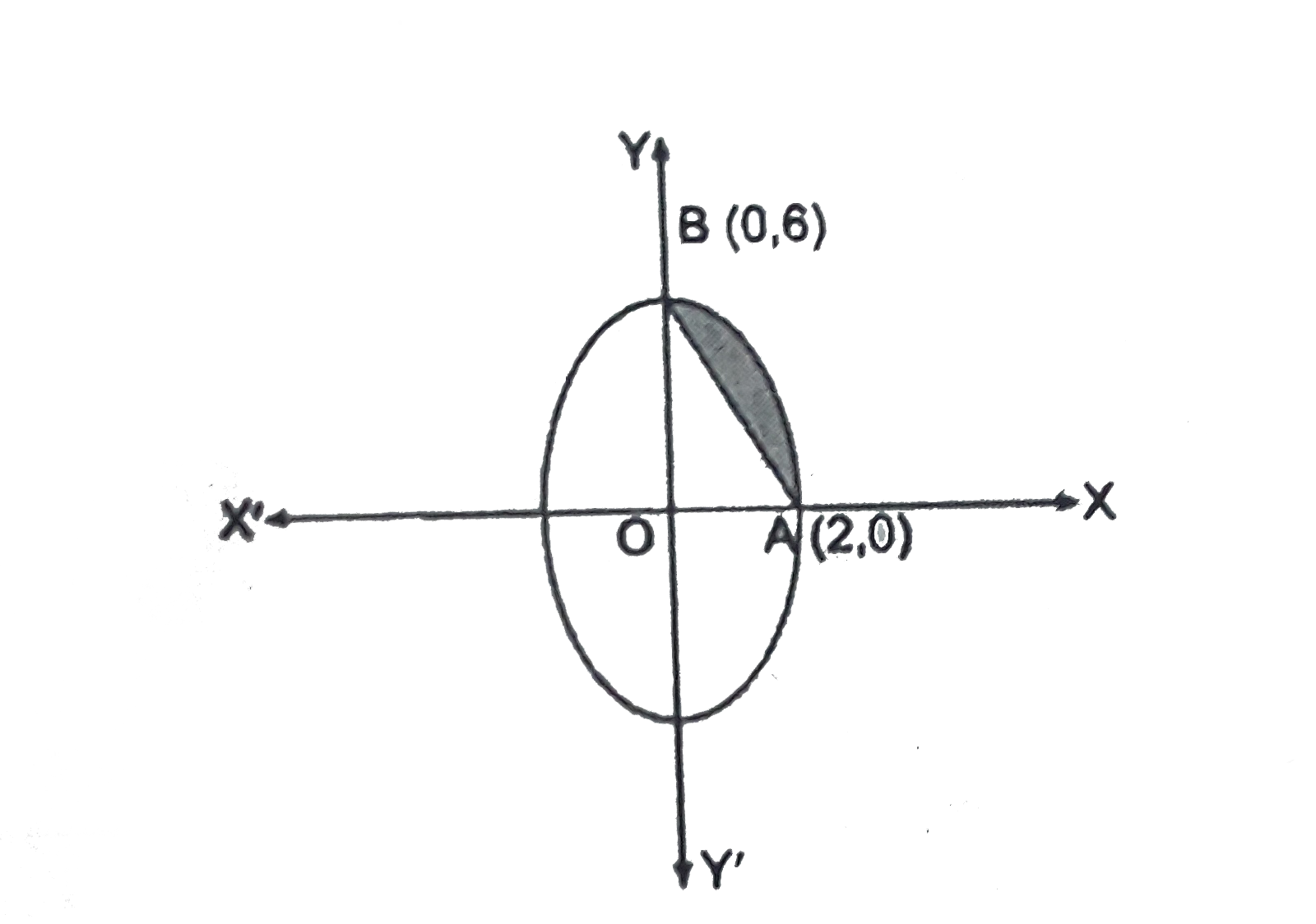
In the adjoining, is the region of the ellipse which lies in first quadrant. If then find the area of the region bounded by chord AB and arc AB.
A
units.
B
units.
C
units.
D
units.
Video Solution
Text Solution
The correct Answer is:C
Equation of AB
Required area
units.
|
Topper's Solved these Questions
- APPLICATIONS OF INTEGRALSBook:NAGEEN PRAKASHANChapter:APPLICATIONS OF INTEGRALSExercise:Exercise 8a
Explore 28 Videos
- APPLICATIONS OF INTEGRALSBook:NAGEEN PRAKASHANChapter:APPLICATIONS OF INTEGRALSExercise:Exercise 8 B Multiple Choice Questions
Explore 10 Videos
- APPLICATIONS OF DERIVATIVESBook:NAGEEN PRAKASHANChapter:APPLICATIONS OF DERIVATIVESExercise:Miscellaneous Exercise
Explore 24 Videos
- Continuity and DifferentiabilityBook:NAGEEN PRAKASHANChapter:Continuity and DifferentiabilityExercise:Miscellaneous Exercise
Explore 23 Videos
Similar Questions
Knowledge Check
In the adjoining, OABO is the region of the ellipse 9x2+y2=36 which lies in first quadrant. If OA=2,OB=6, then find the area of the region bounded by chord AB and arc AB.
 A(3π−8)sq. units.B(3π−7)sq. units.C(3π−6)sq. units.D(3π−9)sq. units.
A(3π−8)sq. units.B(3π−7)sq. units.C(3π−6)sq. units.D(3π−9)sq. units.The area of region bounded by y=|x−1|andy=1 is
A1B2C1/2DNoneThe area of the region bounded by y=x2 and y=−x2+2 is
A53B43C83D1
Find the area of the region bounded by the curve y2=9x and y=3x.
View SolutionFind the area of the region bounded by the curve y2=9x and y=3x.
View SolutionFind the area of the region bounded by the parabola y=x2 and y=|x|.
View SolutionFind the area of the region bounded by the parabola y=x2 and y=|x| .
View SolutionThe area of the region bounded by the curves x=y2−2 and x=y is
View Solution
