The area of the circle x2+y2=16 exterior to the parabola y2=6x is

A

43(4π−√3)

B

43(4π+√3)

C

43(8π−√3)

D

43(8π+√3)

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:C

For the point of intersection of the circle x2+y2=16 and parabola y2=6x
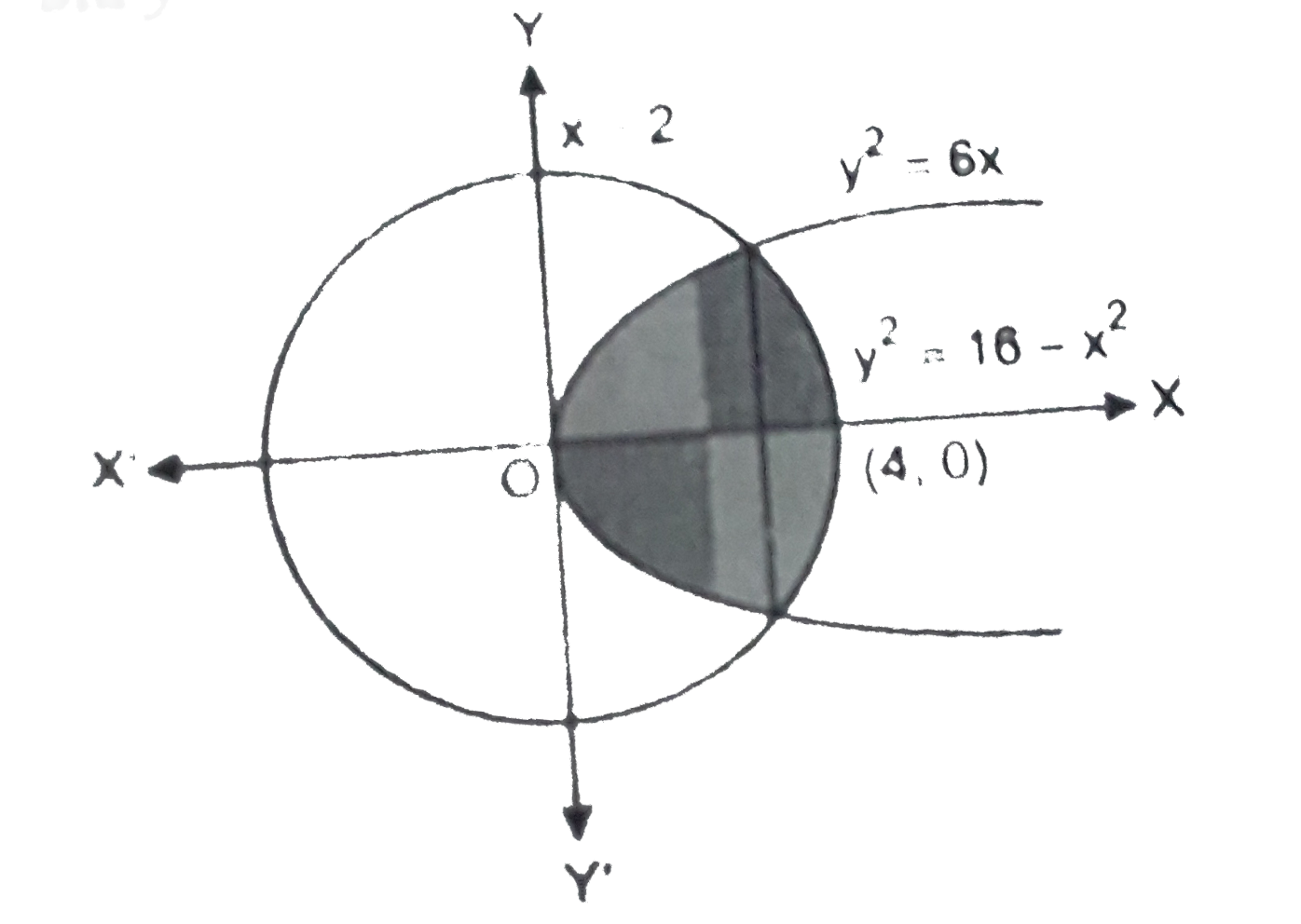
ocr_image
x2+6x=16
⇒x2+6x−16=0
⇒(x+8)(x−2)=0
⇒x=−8,2
∴ Required area
= area of circle −2×(area of shaded region)
=π(4)2−2[∫20√6x+∫42√16−x2dx]
=16π−2√6[x3/23/2]20−2[x2√16−x2+162sin1x4]42
=16π−4√63(23/2−0)−2[0+8sin−1(1)−√16−4−8sin−112]
=16π−4√6×2√23−2[8×π2−√12−8×π2]
=16π−16√33−16π3+4√3
=32π3−4√33
=43(8π−√3) sq. units.

Updated on:21/07/2023
|
Class 12MATHSAPPLICATION OF INTEGRALS

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • The area of the portion of the circle x2+y2=64 which is exterior to the parabola y2=12x is

    A(8π−√3)squnits
    B163)(8−√3)squnits
    C163)(8π−√3)squnits
    Dnone of the above

  • The area of the portion of the circle x2+y2=64 which is exterior to the parabola y2=12x is

    A(8π−√3)squnits
    B163)(8−√3)squnits
    C163)(8π−√3)squnits
    Dnone of the above

  • Area common to the circle x2+y2=64 and the parabola y2=6ax is

    A163(4π+√3)
    B163(8π−√3)
    C163(4π−√3)
    DNone