Question

Using the method of
integration find the area bounded by the curve $\left|x\right|+\left|y\right|=1$[Hint: The required region
is bounded by lines $x+y=1,x-y=1,x+y=1$
and $-x-y=1].$

Explanation

Given curve, `|x| +|y|=1`

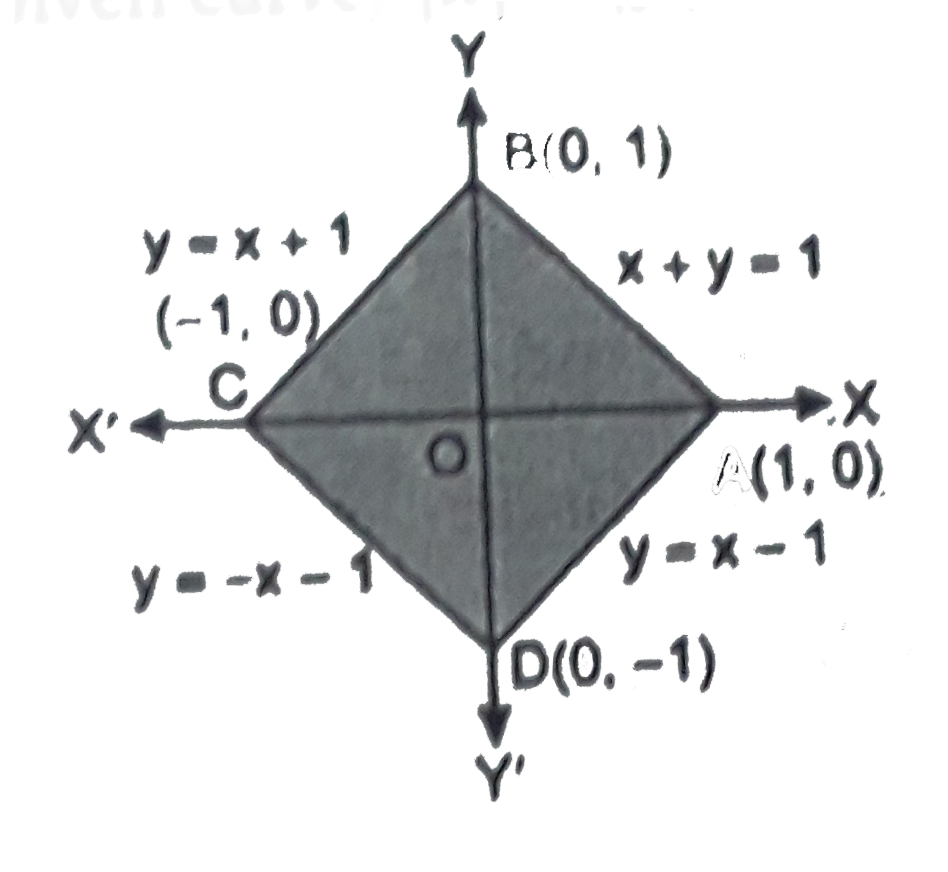
In first quadrant (x gt 0, y gt 0) then line AB is
`x+y=1.`
In second quadrant (x lt 0, y gt 0) then line BC is
`-x+y=1.`
In third quadrant (x lt 0, y lt 0) then line CD is
`-x-y=1.`
In fourth quadrant (x gt 0, y lt 0) then line DA is
`x-y=1`.
Since ABCD is a square
therefore, required area = 4 `xx` (area of shaded region in first quadrant)
`=4int_(0)^(1)(1-x)dx`
`=4[x-(x^(2))/(2)]_(0)^(1)=4[(1-(1)/(2))-0]`
`=2` sq. units.