A unifrom chain of mass M and length L lies on a table with nth part of it hanging off the table. Find the work required to slowly pull the hanging part up to the table.
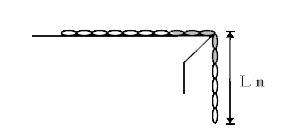
Here work is done against the gravity.
∴W=ΔU=Uf−Uin
Taking table as reference level, i.e. Uf=0
∴W=−Uin=−[−mghC.M.]
=MghC.M.
here mass of hanging part is m = Mn
and its centre of mass is hC.M. hight below the table
where hC.M.=(L/n)2=L2n
∴W=(Mn)g(L2n)=MgL2n2
Topper's Solved these Questions
- WORK, POWER & ENERGY Book:MOTIONChapter:WORK, POWER & ENERGY Exercise:SOLVED EXAMPLES
Explore 5 Videos
- WORK, POWER & ENERGY Book:MOTIONChapter:WORK, POWER & ENERGY Exercise:QUESTIONS FOR PRACTICE
Explore 25 Videos
- WORK, POWER & ENERGY Book:MOTIONChapter:WORK, POWER & ENERGY Exercise:Exercise - 3 Section-B
Explore 22 Videos
- WAVESBook:MOTIONChapter:WAVESExercise:EXERCISE -4 (Level -II) (previous year JEE Advanced)
Explore 10 Videos
Similar Questions
A uniform chain of mass m and length l overhangs a table with its two third part on the table. Fine the work to be done by a person to put the hanging part back on the table.
View SolutionA uniform chain of mass m and length l is kept on a horizontal table with half of its length hanging from the edge of the table.Work done in pulling the chain on to the table so that only 1/5 th of its length now hangs from the edge is K(mgl200) .Find the value of K.
View Solution
Knowledge Check
A chain of mass m and length l is placed on a table with one-sixth of it hanging freely from the table edge. The amount of work. done to pull the chain on the table is
Amgl/4Bmgl/6Cmgl/72Dmgl/36A chain of mass m and length l is placed on a table with one-sixth of it hanging freely from the table edge. The amount of work. done to pull the chain on the table is
Amgl/4Bmgl/6Cmgl/72Dmgl/36A chain is placed on a rough table, partially hanging, as shown in the figure. The coefficient of static friction between the chain and the table is μ = 0.4. If the maximum possible length of the hanging part is x and the length of the chain is L, then what is the value of Lx?
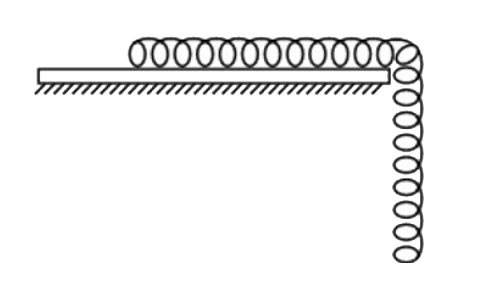 AμL1+μBμL1−μC(1−μ)L1+μDμL
AμL1+μBμL1−μC(1−μ)L1+μDμL
(a) Why can we not associate a potential energy with the frictional force as we did with the gravitational force ? (b) A uniform chain of mass m and length I is lying on a table with (1/4) of its length hanging freely from the edge. Find the amount of work required to be done in dragging the chain on the table completely.
View SolutionA uniform chain of mass m and length l is lying on a horizontal table with one-third of its length hanging over the edge of the table.

What is the speed of the chain, when it just loses contact with the table?
View SolutionA chain is held on a frictionless table with 1/n th of its length hanging over the edge. If the chain has a length L and a mass M, how much work is required to pull the hanging part back on the table?
View SolutionA uniform chain of mass m & length L is kept on a smooth horizontal table such that Lnportion of the chaing hangs from the table. The work dione required to slowly bringsthe chain completely on the table is
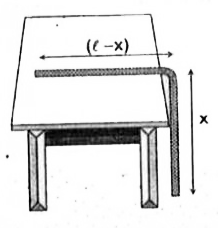
View SolutionA uniform chain of mass m and length I is kept on the table with a part of it overhanging (see figure). If the coefficient of friction between the table and the chain is 1/3 then find the maximum length of the chain that can overhang such that the chain remain in equilibrium.
View Solution