Two particles of mass m each are kept on a horizontal circular platfrom on two mutually perpendicular radii at equal distance r from the center of the table. The particles are connected with a string, which is just taught when the platfrom is not rotating. The coefficient of static friction between the platfrom and block is μ (now if angular speed of platfrom m is slowly increased). Find the maximum angular speed (ω) of platfrom about it center so that the blocks remain stationery relative to platform. (If μ=1√2, r=2.5m and g=10m/s2)
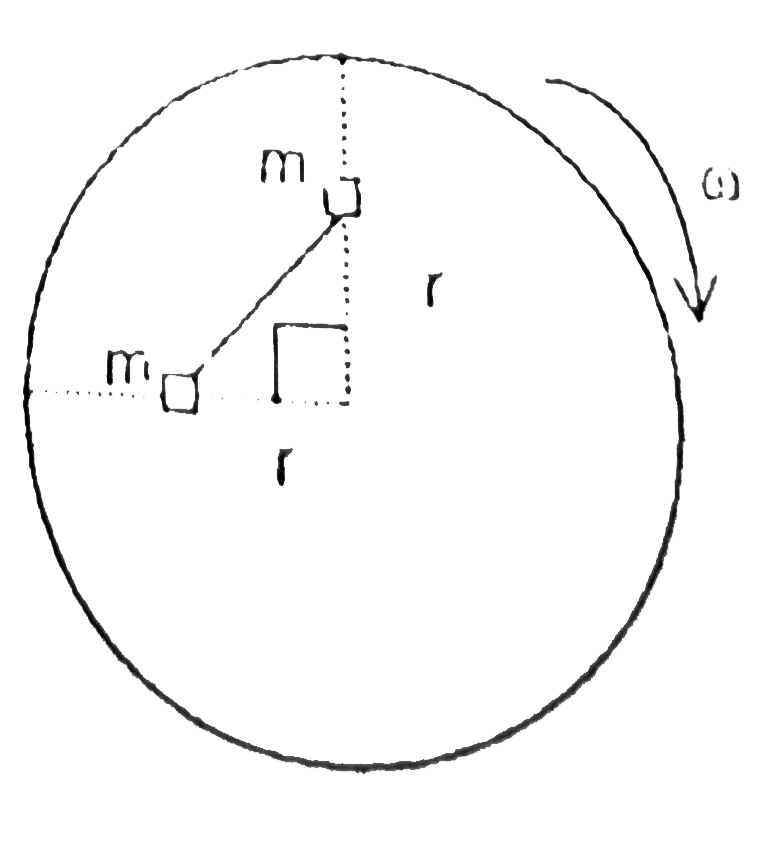
For small values of ω friction will be directed radially inwards as the tension in the spring is zero. The string will develop tension only if the centinfugal force FC exceeds the limiting friction fc. i.e. when
mω2r>μmg (fc=μmg)
or ω=√μgr
in this case direction of friction will be as shown in the figure.
For equilibrium
Fc=Tcos45∘+fccosθ
And Tsin45∘=fcsinθ
Eliminiating T, we get
Fc=fc(sinθ+cosθ)
i.e. mω2r=μmg[√2sin(θ+45∘)]
or ω2=√2μgrsin(θ+45∘)
maximum value of sin(θ+45∘) is 1
∴ Maximum value of ω=√√2μgr=2

Topper's Solved these Questions
Similar Questions
A block on table shown in is just on the edge of slipping Find the coefficient of static friction between the blocks and table
 .
.
View SolutionA particle of mass m connected with a hanging bob of mass M by an inextensible string is stationary relative to the rotating platform . The coefficient of static friction between the particle and platform is μ
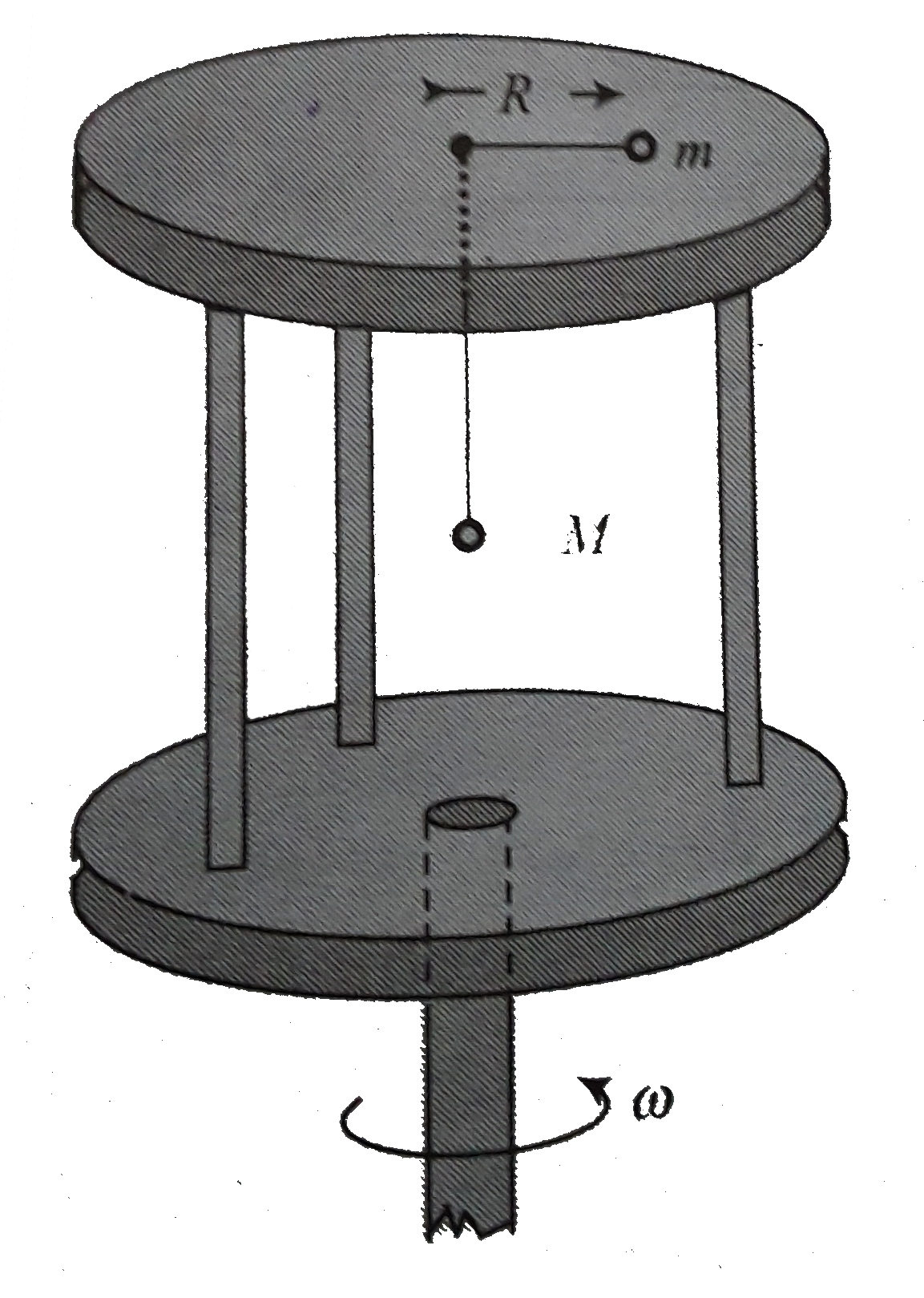
Find the
a. maximum angular speed ω1
b. maximum angular speed ω2
(forM>μm)
View Solution
Knowledge Check
Three particles of equal mass M each are moving on a circular path with radius r under their mutual gravitational attraction. The speed of each particle is
A√GM√2RB√GM√3RC√GM2RD√GM3RA block of mass m is stationary on a horizontal surface. It is connected with a string which has no tension. The coefficient of friction between the block and surface is m . Then , the frictional force between the block and surface is:
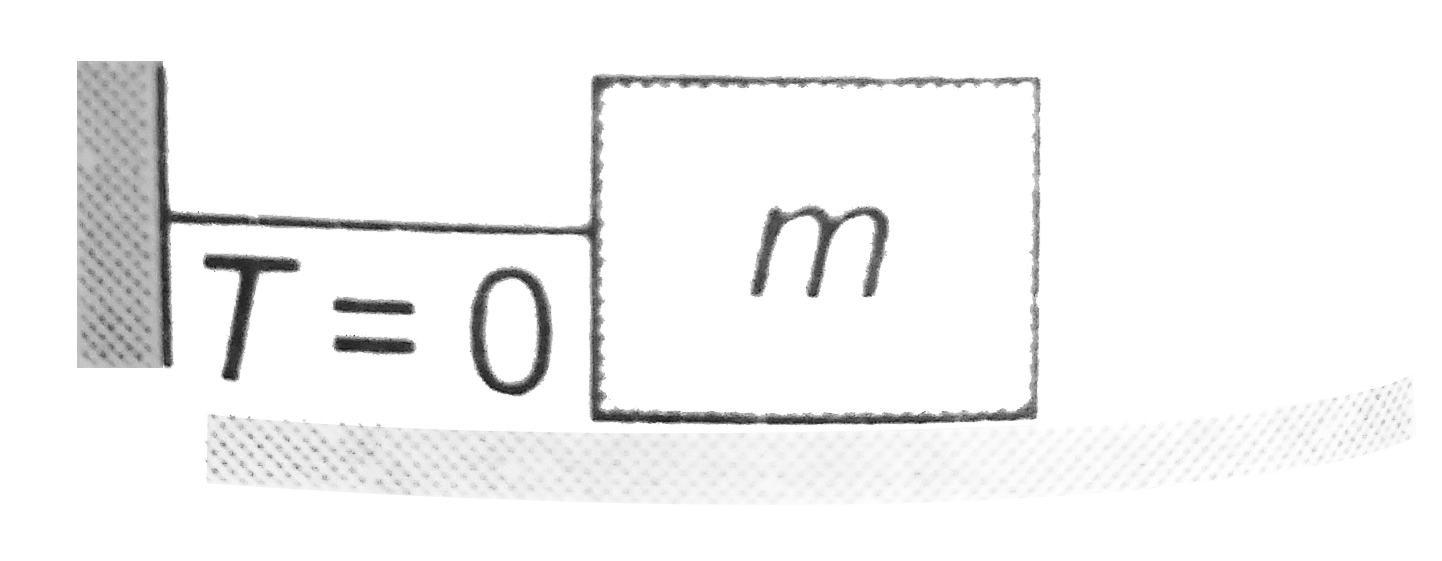 AzeroBμmgCμmgμDNone of these
AzeroBμmgCμmgμDNone of theseBlock B lying on a table weighs w. The coefficient of static friction between the block and the table is mu. Assume that the cord between B and the knot is horizontal. The maximum weight of the block A for which the system will be stationary is
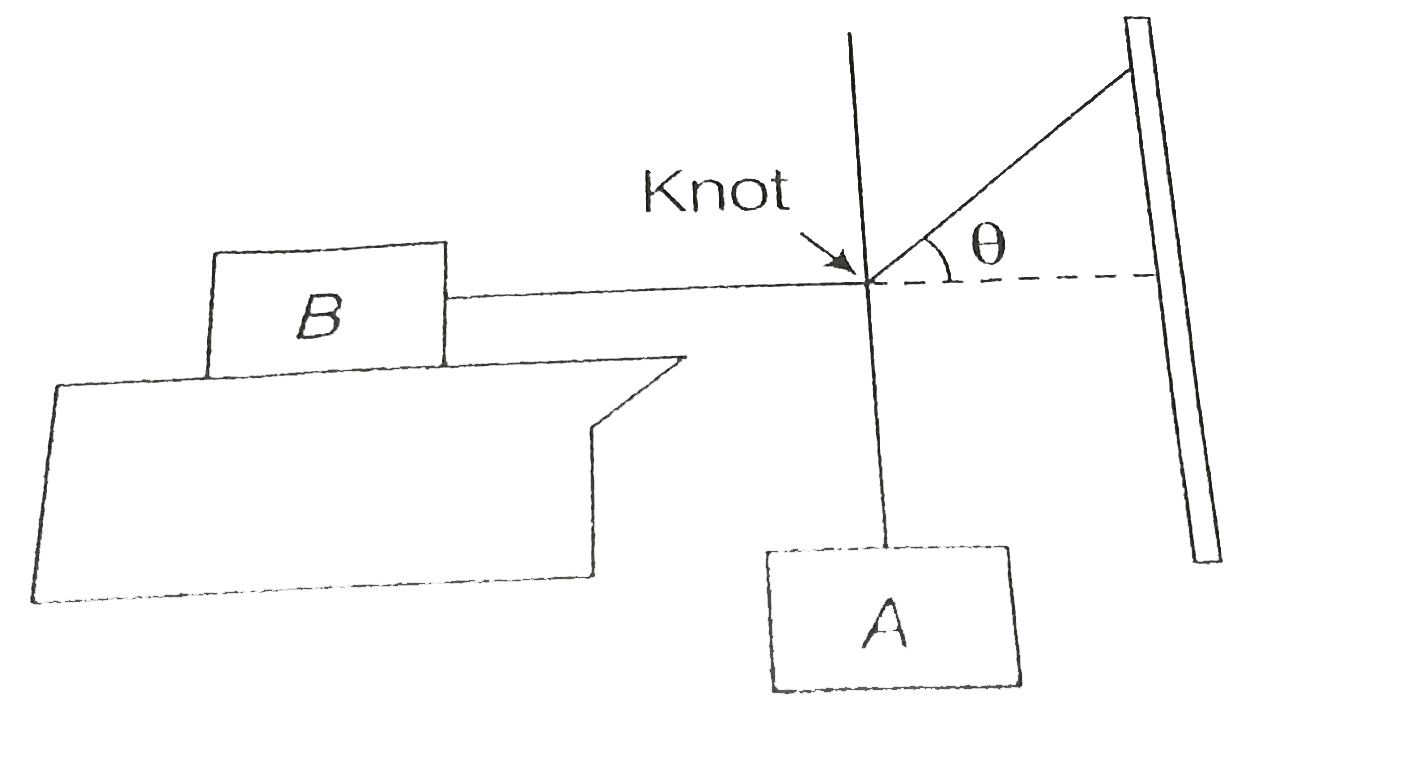 AwtanθμBμwtanθCμw√1+tan2θDμwsinθ
AwtanθμBμwtanθCμw√1+tan2θDμwsinθ
Coefficient of kinetic friction and coefficient of static friction between two blocks is μk and μs respectively. In general
View SolutionA particle of mass m is kept on the axis of a fixed circular ring of mass M and radius R at a distance x from the centre of the ring. Find the maximum gravitational force between the ring and the particle.
View SolutionThe coefficient of static friction between the two blocks shown in figure is μ and the table is smooth. What maximum horizontal forced F can be applied to he block of mass M so that the block move together?

View SolutionFind the acceleration of the block of mass M in the situation of figure. The coefficient of friction between the two blocks is μ1 and that between the bigger block and the ground is μ2.
View SolutionA coin kept at a distance of 5 cm from the centre of a turntable of radius 1.5m just begins to slip when the turntable rotates at a speed of 90r.p.m. Calculate the coefficient of static friction between the coin and the turntable [g=9.8m/s2]
View Solution
