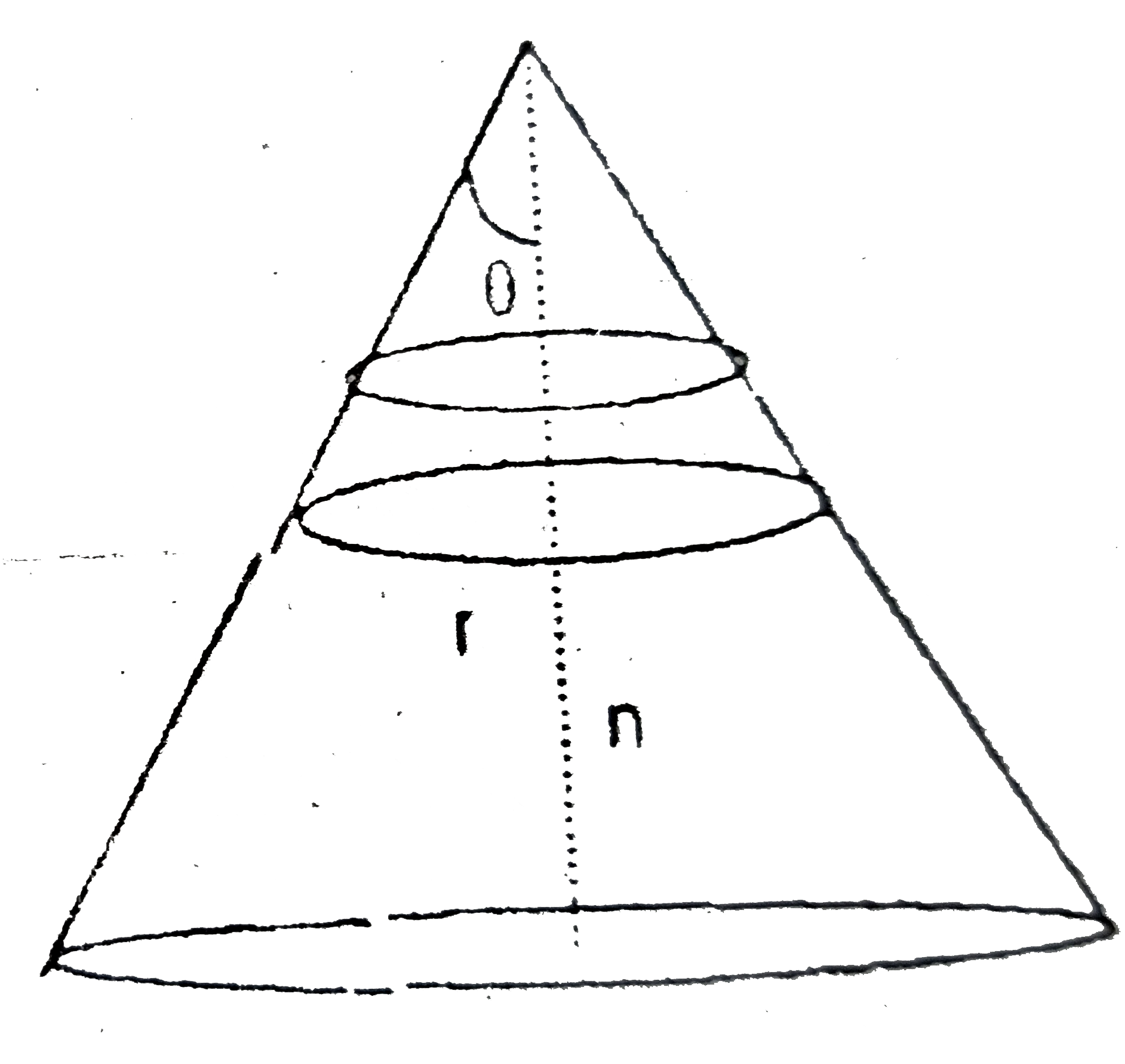
Q charge is uniformaly distributed over the same surface of a right circular cone of semi -vertical angle theta and height h The cone is uniformly rotated about its axis at angular velocity omega Calculated associated magnetic dipole moment
 .
.
A ring of radius r with charge q rotating with angular velocity ω is equilvant to a dipole of dipole moment
=NIA=qfA=qω2πA
dipole moment of differential ring
dp=QπRl2πrdxcosθ⋅ω2ππr2
Rr=hh−x
⇒dp=QcosθπR.hdxcosθωπ(h−xnR)3
⇒P=Qω.R2h4∫h0(h−x)3dx=QωR2h4⋅∣∣
∣∣(h−x)44∣∣
∣∣0h
=ωQR2h4h44=QωR24=Qω4h2tan2θ
Topper's Solved these Questions
Similar Questions
A ring of mass m and radius R is given a charge q. It is then rotated about its axis with angular velocity ω.Find
(iii)Magnetic moment of ring.
View SolutionA uniform charged disc whose total charge has magnitude q and whose radius is r rotates with constant angular velocity of magnitude ω. What is the magnetic dipole moment?
View Solution
Knowledge Check
If the lateral surface of right circular cone is 2 times its base , then the semi-vertical angle of the cone must be :
A15∘B30∘C45∘D60∘If the lateral surface of right circular cone is 2 times its base , then the semi-vertical angle of the cone must be :
A15∘B30∘C45∘D60∘
A uniformly charged thin conducting spherical shell of radius R and charge q is rotated with constant angular velocity ω about a diameter. The magnetic dipole moment of the system is
View SolutionA ring of mass m and radius R is given a charge q. It is then rotated about its axis with angular velocity ω.Find
(ii) Magnetic field produced at the centre of ring.
View SolutionA thin uniformring of radius R carrying uniform charge Q and mass M rotates about its axis with angular velocity ω. The ratio of its magnetic moment and angular momentum is:
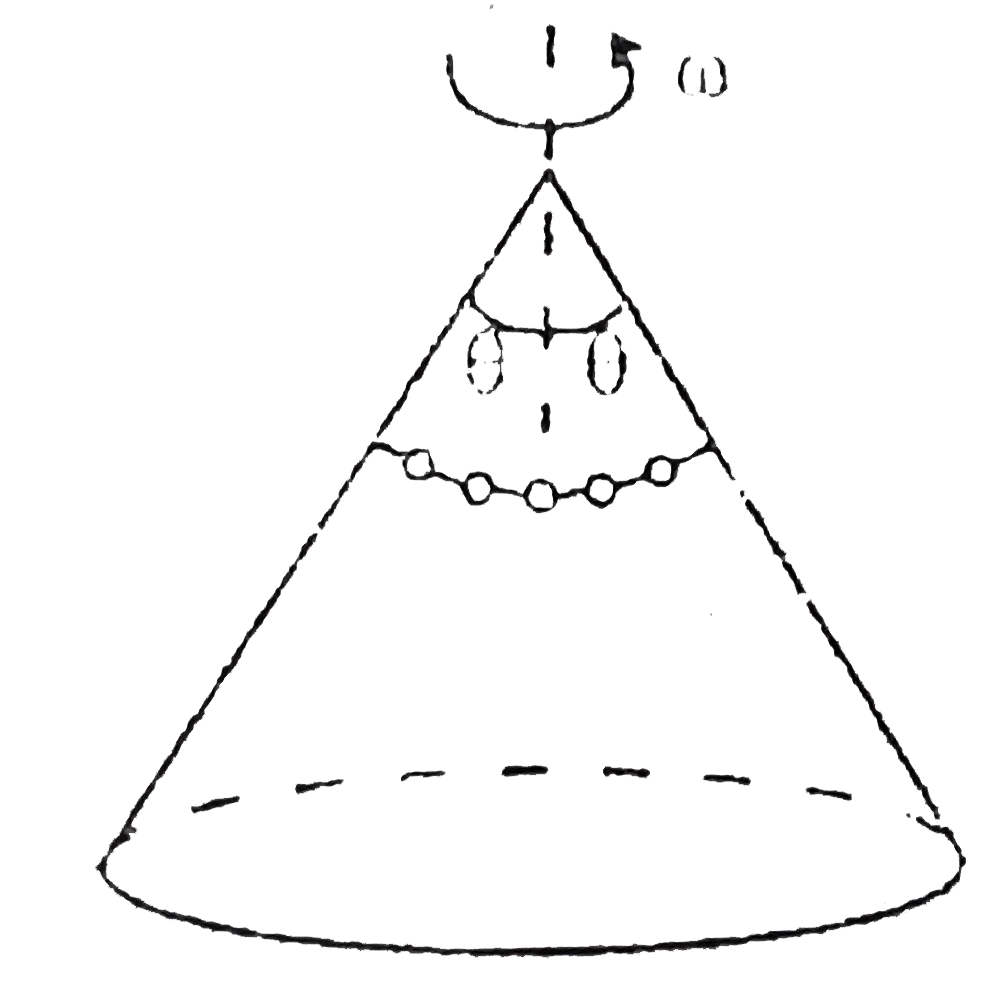
View SolutionA chain of mass 'm' and radius 'r' is placed onto a cone of semi vertical angle θ. Cone rotated with angular velocity ω. Find the tension in the chain if it does not slide on the cone.
View SolutionA rod has a total charge Q uniformly distributed along its length L. If the rod rotates with angular velocity ω about its end, compute its magnetic moment.

View SolutionA liquid has been poured into a cylindrical vessel. What shape will the surface of the liquid have if the vessel is rotated uniformly about its axis with an angular velocity ω ?
View Solution
