Two balls of same mass are projected as shown, by compressing the springs of different force constants K1 and K2 by equal distance. The first ball is projected upwards along smooth wall and the other on the rough horizontal floor with coefficient of friction μ. if the first ball goes up by height h, then the distance covered by the second ball will be

2hK2μK1
hK12μK2
3hK22μK1
hK2μK1
The correct Answer is:4
12K1x2=mgh orx2=2μmgx0K2
12K2x2=μmgx0 or x2=2μmgx0K2
Equating, x0=hμ.K2K1
Topper's Solved these Questions
- TEST PAPERSBook:BANSALChapter:TEST PAPERSExercise:PHYSICS PART (A)
Explore 32 Videos
- TEST PAPERSBook:BANSALChapter:TEST PAPERSExercise:PHYSICS PART (B)
Explore 3 Videos
- TEST PAPERSBook:BANSALChapter:TEST PAPERSExercise:PHYSICS PART- C
Explore 4 Videos
- SEMICONDUCTORSBook:BANSALChapter:SEMICONDUCTORSExercise:CBSE Question
Explore 32 Videos
Similar Questions
A ball is projected from a point on a smooth horizontal floor at an angle θ with the horizontal . Speed of projection is u . Ball collides with the floor several times . Find the total time and horizontal distance covered by the ball .
View SolutionA block of height h is projected along a rough surface of coefficient of friction μ. Find the point of application of the normal force on the block for μk=0.5.
View Solution
Knowledge Check
Two ball of same mass are projected as shown. By compressing equally (say x) the springs of different force constants K1 and K2 by equal magnitude. The first ball is projected upwards along smooth wall and the other on the rough horizontal floor with coefficient of friction μ. If the first ball goes up by height h, then the distance covered by the second ball will be :
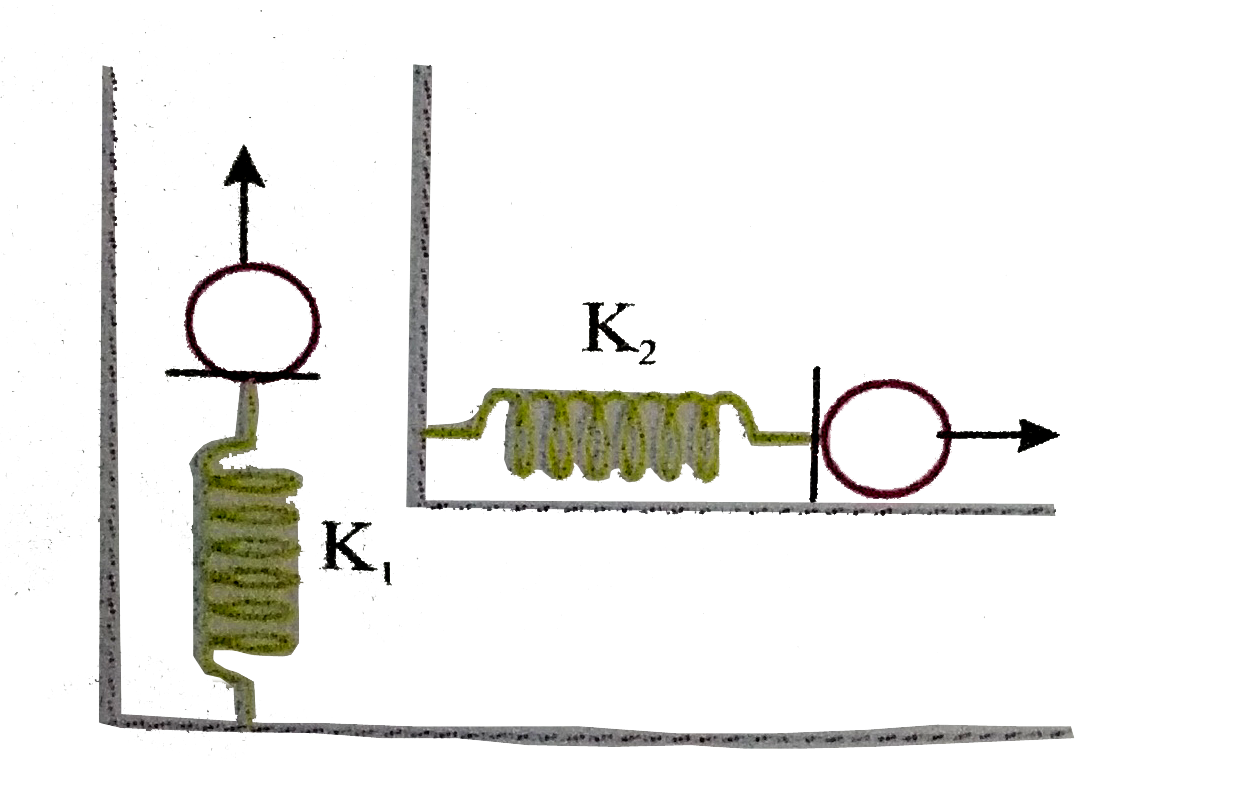 .A2hK2μK1BhK12μK2C3hK22μK1DhK2μK1
.A2hK2μK1BhK12μK2C3hK22μK1DhK2μK1An elastic ball with coefficient of elasticity 1/2 is dropped from rest at a height h on a smooth floor . The total distance covered by the ball is
Amore than 2hBless than 2h but more than 32 hCless than 32 h but more than 43 hDless than 43hA ball is dropped from a height 'h' on to a floor of coefficient of restitution 'e'. The total distance covered by the ball just before second hit is
Ah(1−2e2)Bh(1+2e2)Ch(1+e2)Dhe2
Three small balls of equal mass (m) are suspended from a thread and two springs of same force constant (K) such that the distances between the first and the second ball and the second third ball are the same. Thus the centre of mass of the whole system coincides with the second ball. The thread supporting the upper ball is cut and system starts a free fall. Find the distance of the centre of mass of the system from the second ball when both the springs acquire their natural length in the falling system.
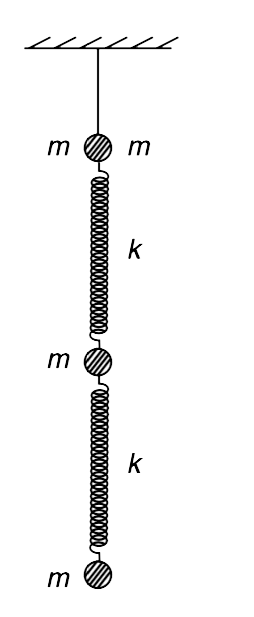
View SolutionA ball (initially at rest) falls vertically for 2 s and hits a smooth plane inclined at 30∘ to the horizontal. The coefficient of restitution is 58. The distance along the plane between the first and second impact of the ball is
View SolutionA ball is dropped on to a horizontal plate from a height h = 9 m above it. If the coefficient of restitution is e = 1/2, the total distance travelled before the ball comes to rest is
View SolutionA ball is dropped on to a horizontal plate from a height h = 9 m above it. If the coefficient of restitution is e=1/2, the total distance travelled before the ball comes to rest is
View SolutionThe masses of five balls at rest and lying at equal distance in a straight line are in geometrical progression with ratio 2 and their coefficients of restitution are each 2/3 . If the first ball is started towards the second with velocity u, then the velocity communicated to 5th ball is
View Solution