A particle of mass m1 collides head on with a stationary particles of mass m2. If m1m2 gte, where e is the coefficient of restitution, then :
m1 will retrun back
m1 will move in same direction
m1 will stop
unpredictable
The correct Answer is:2
m1V=m1V1+m2V2.......(1)
and V2−V1=eV....(2)
On solving V1=V(m1−em2)(m1+m2)
Given m1m2>e
So, V1 is positive and thus, m1 will move in same direction.
Topper's Solved these Questions
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART (A)
32 Videos
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART (B)
3 Videos
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART- C
4 Videos
- SEMICONDUCTORSBOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question
32 Videos
Similar Questions
A particle of mass m1 collides elastically with a stationary particle of mass m2(m1>m2). Find the maximum angle through which the striking particle may deviate as a result of the collision.
View SolutionA particle of mass m1 experiences a perfectlly elastic collision with a stationary particle of mass m2. Match the entires in column I to all the entires in column II.
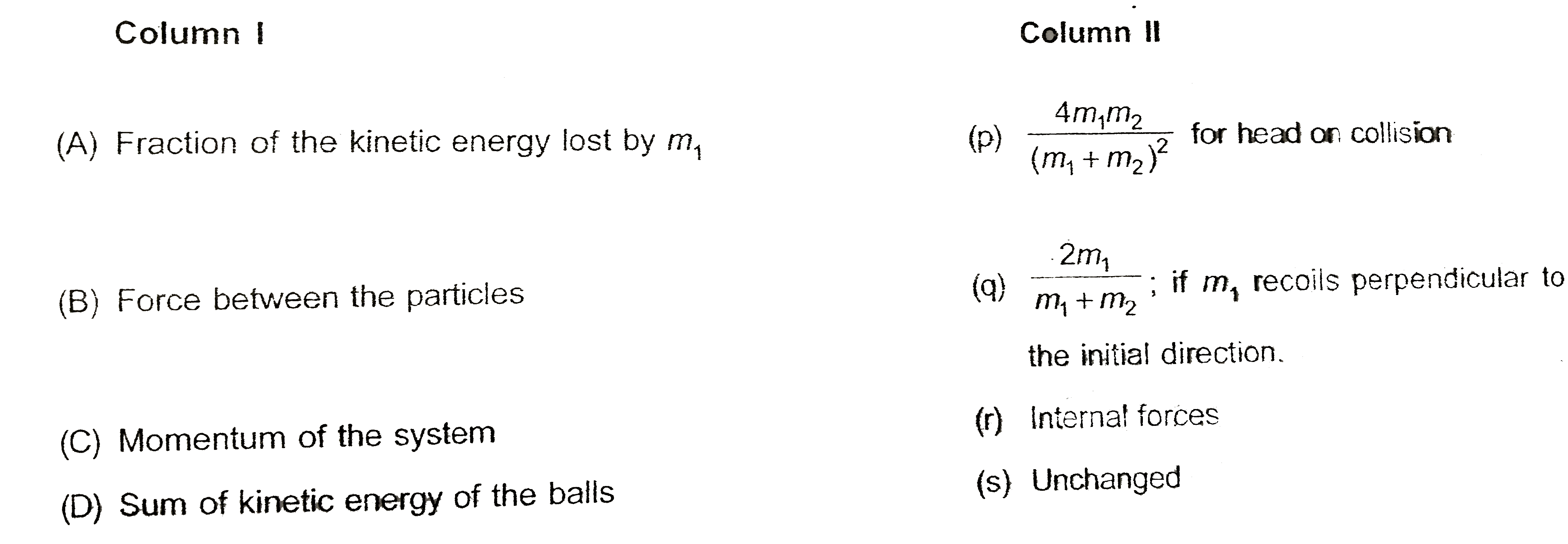
View Solution
Knowledge Check
A partical of mass m1 collides head on with a stationary partical of mass m2. If m1m2>e where e is the coefficient of restitution, then :
Am1 will return backBm1 will move in same directionCm1 will stopDunpredicatableA particle (A) of mass m1 elastically collides with another stationary particle (B) of mass m2. Then :
Am1m2=12 and the particles fly a part in the opposite direction with equal velocities.Bm1m2=13 and the particles fly apart in the opposite direction with equal velocities.Cm1m2=21 and the collision angle between the particles is 60∘ symmetrically.Dm1m2=21 and the particles fly apart symmetrically at an angle 90∘A particle of mass m, collides with another stationary particle of mass M. If the particle m stops just after collision, then the coefficient of restitution for collision is equal to
A1BmMCM−mM+mDmM+m
A particle of mass m1 moving with a velocity of 5 ms−1 collides head on with a stationary particle of mass m2. After collision both the particle move with a common velocity of 4ms−1then the value of m1/m2is :
View SolutionA ball of mass m1, collides elastically and head on with ball of mass m2 at rest. Then
View SolutionA ball moving with velocity 2ms−1 collides head on with another stationary ball of double the mass. If the coefficient of restitution is 0.5, then their velocities (in ms−1) after collision will be
View SolutionThe first ball of mass m moving with the velocity υ collides head on with the second ball of mass m at rest. If the coefficient of restitution is e, then the ratio of the velocities of the first and the second ball after the collision is
View SolutionA block of mass m moving with speed v0 strikes another particle of mass 2m at rest. If collision is head - on and the coefficient of restitution e=1/2 , then the loss in kinetic energy will be
View Solution