A rigid body of radius R, either hollow or solid, lies on a smooth horizontal surface. The body is pulled form rest by a horizontal force acting tengentially from the highest point. The distance travelled by the body in the time in which it makes one full rotation is the same that it will make in one full rotation during pure rolling. The rigid body will be
solid sphere
hollow sphere
circular disc
hollow cylinder
The correct Answer is:4
τ=F×R=Iα
In one full rotation ,
θ=2π=12αt2
t2=4πα=4πIFR
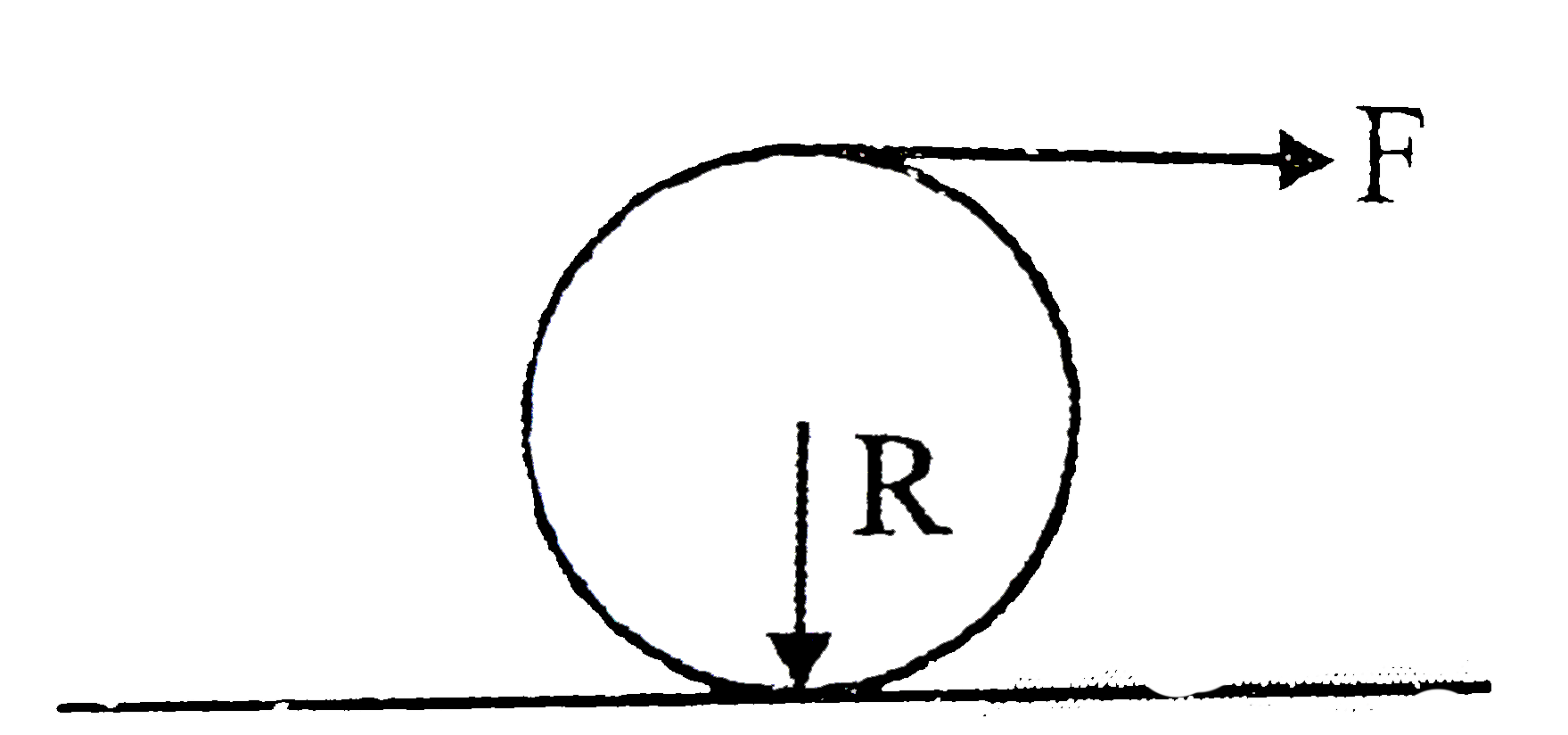
Linear acceleration, a=F/m
Distance travelled in one rotation
=2πR (given)
=12at2=12FM.4πlFR=2πIMR
It gives I=MR2
It is a hollow cylinder
Topper's Solved these Questions
- TEST PAPERSBook:BANSALChapter:TEST PAPERSExercise:PHYSICS PART (A)
Explore 32 Videos
- TEST PAPERSBook:BANSALChapter:TEST PAPERSExercise:PHYSICS PART (B)
Explore 3 Videos
- TEST PAPERSBook:BANSALChapter:TEST PAPERSExercise:PHYSICS PART- C
Explore 4 Videos
- SEMICONDUCTORSBook:BANSALChapter:SEMICONDUCTORSExercise:CBSE Question
Explore 32 Videos
Similar Questions
The reation between the time t and position x for a particle moving on x-axis is given by t=px2+qx, where p and q are constants. The relation between velocity v and acceleration a is as
View SolutionA car is moving along a straight line. It's displacemetn (x)- time (t) grapg is shown in column II. Match the entries in column I with points on graph.
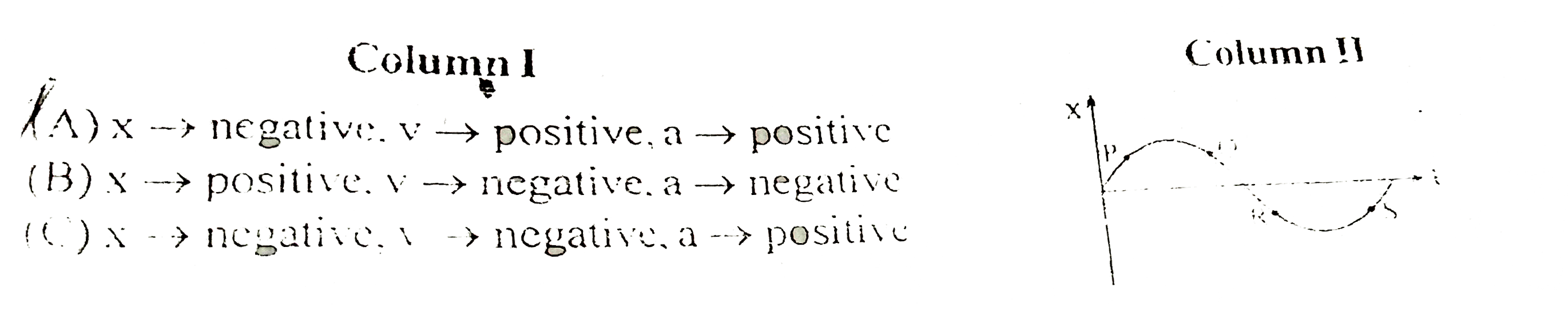
View SolutionIf the depth d to which a bullet of kinetic energy K can penetrate into a human body of modulus of electicity E, using the method of dimension, establish a relation between d, K and E . If K=cdxEy, fill x+y in OMR sheet. Modulus of elasticity =Force/Area ,×lΔl
View SolutionHow does the width of the depletion layer of a p-n junction diode change with decrease in reverse bias?
View SolutionA holow sphere of radius R lies on a smoth horizontal surface. It is pulle dby a horizontl force acting tasngentially from the highest point. Find the distance travelled by the sphere durint eh time it makes oen full rotation.
View SolutionVelocity And Acceleration Of A Point In Rotating Rigid Body In Pure Rotation
View SolutionA rigid body is rotating about a vertical axis. In t second, the axis gradually becomes horizontal. But the rigid body continues to make v rotations per second throughout the time interval of 1 second. If the moment of inertia I of the body about the axis of rotation can he taken as constant, then the torque acting on the body is
View SolutionA body kept on a smooth horizontal surface is pulled by a constant horizontal force applied at the top point of the body. If the body rolls purely on the surface, its shape can be-
View SolutionA solid sphere of radius R lies on a smooth horizontal surface. It is pulled by a horizontal force acting tangentially from the highest point. Find the distance traveled by the sphere during the times it makes one rotation.
View SolutionA string is wrapped around a cylinder of radius R and pulled at the top most point horizontally by a force F The cylinder rolls without slipping on the horizontal surface. The work done by the force by the time the cylinder makes one full rotation is
View Solution(A): In rolling, all points of a rigid body have the same linear speed. (R) : The rotational motion donot change the linear velocity of rigid body.
View Solution(A) : In rolling, all points of a rigid body have the same linear speed. (R ) : The rotational motion donot change the linear velocity of rigid body.
View Solution(A) : In rolling, all points of a rigid body have the same linear speed. (R) : The rotational motion donot change the linear velocity of rigid of rigid body.
View SolutionA body is in pure rolling over a horizontal surface. If the rotational kinetic energy of that body is 40% of its total kinetic energy, then the body is
View SolutionAssertion : Speed of any point on rigid body executing rolling motion can be calculated by expression v=rω, where r is distance of point from instantaneous centre of rotation
Reason : Rolling motion of rigid body can be considered as a pure rotation about instantaneous centre of rotation.
View Solution