Two thin rings of slightly different radii are joined together to make a wheel (see figure) of radius R. There is a very small smooth gap between the two ring. The wheel has a mass M and its centre of mass is at its geometrical centre. The wheel stands on a smooth surface and a small particle of mass m lies at the top (A) in the gap between the rings. The system is released and the particle begins to slide down along the gap. Assume that the ring does not lose contact with the surface.
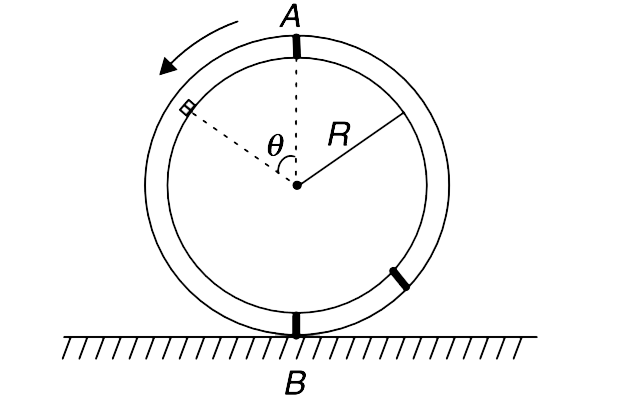
(a) As the particle slides down from top point A to the bottom point B, in which direction does the centre of the wheel move?
(b) Find the speed of centre of the wheel when the particle just reaches the bottom point B. How much force the particle is exerting on the wheel at this instant?
(c) Find the speed of the centre of the wheel at the moment the position vector of the particle with respect to the centre of the wheel makes an angle q with the vertical. Do this calculation assuming that the particle is in contact with the inner ring at desired value of θ
The correct Answer is:(a) First moves to right and then to left
(b) vw=2m√gRM(M+m)
(c) vw=
⎷2m2gRcos2θ(1−cosθ)(M+m)2+Mmco2θ
Step by step video, text & image solution for Two thin rings of slightly different radii are joined together to make a wheel (see figure) of radius R. There is a very small smooth gap between the two ring. The wheel has a mass M and its centre of mass is at its geometrical centre. The wheel stands on a smooth surface and a small particle of mass m lies at the top (A) in the gap between the rings. The system is released and the particle begins to slide down along the gap. Assume that the ring does not lose contact with the surface. (a) As the particle slides down from top point A to the bottom point B, in which direction does the centre of the wheel move? (b) Find the speed of centre of the wheel when the particle just reaches the bottom point B. How much force the particle is exerting on the wheel at this instant? (c) Find the speed of the centre of the wheel at the moment the position vector of the particle with respect to the centre of the wheel makes an angle q with the vertical. Do this calculation assuming that the particle is in contact with the inner ring at desired value of theta by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Knowledge Check
A thin spherical shell of mass M and radius R has a small hole. A particle of mass m released at its mouth. Then
Athe particle will execute S.H.M inside the shellBthe particle will oscillate inside the shell, but the oscillations are not simple harmonicCthe particle will not oscillate, but the speed the particle will go on increasingDnone ot theseFor a uniform ring of mass M and radius R at its centre
Afield and potential both are zeroBfield is zero but potential is GMRCfield is zero but potential is −GM/RDmagnitude of field is GMR2 and potential −GMRA wheel comprises a ring of radius R and mass M and three spokes of mass m each. The moment of inertia of the wheel about its axis is
 A(M+m4)R2B(M+m)R2C(M+3m)R2D(M+m2)R2
A(M+m4)R2B(M+m)R2C(M+3m)R2D(M+m2)R2
The centre of a wheel rolling without slipping on a plane surface moves with a speed v0. A particle on the rim of the wheel at the same level as the centre of wheel will be moving with speed
View SolutionFind the centre of mass of a uniform semicircular ring of radius R and mass M.
View SolutionA ring mass m and radius R has three particle attached to the ring as shown in the figure. The centre of the centre v0. Find the kinetic energy of the system. (Slipping is absent).
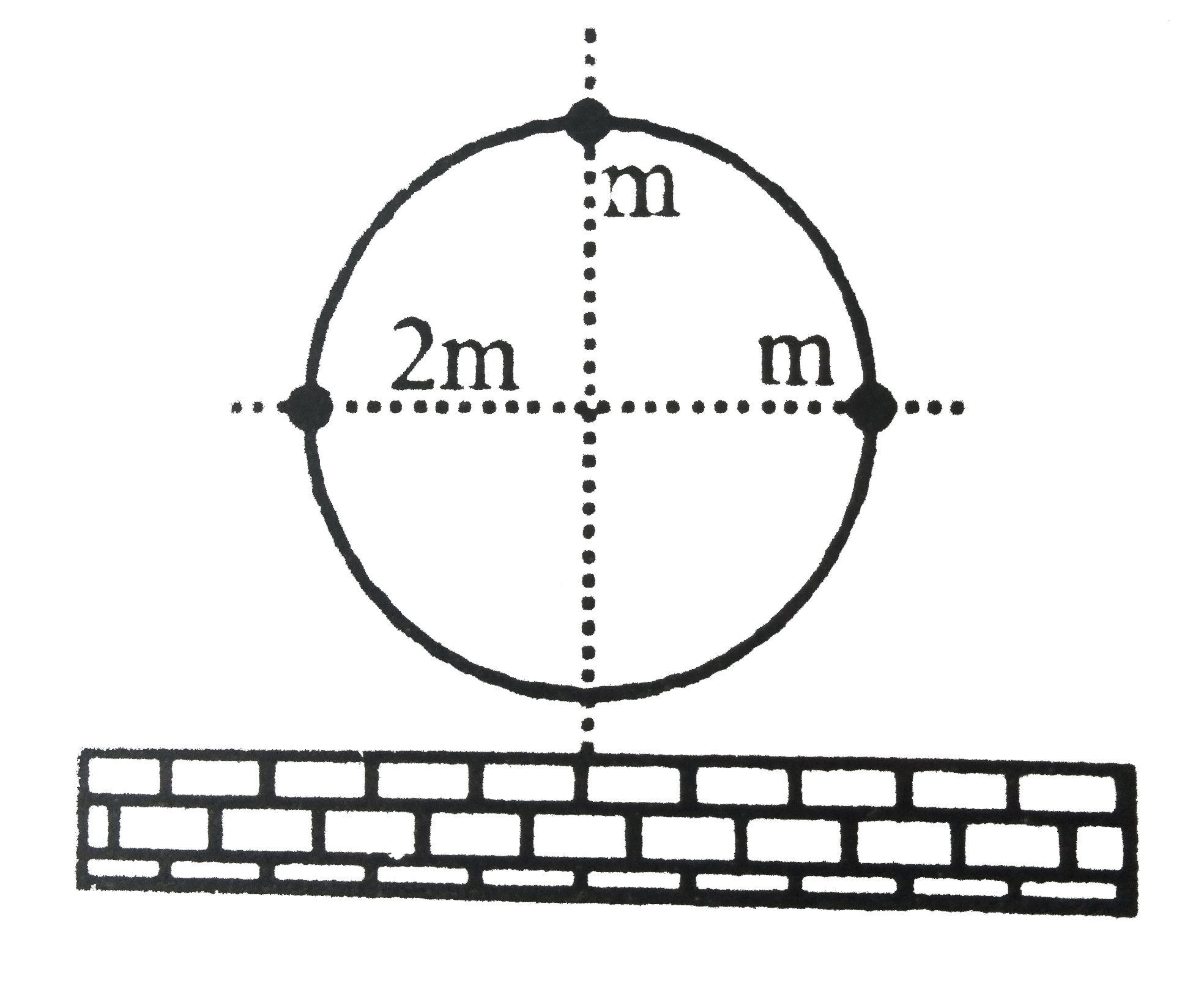 .
.
View SolutionThe centre of a wheel rolling on a plaen surface moves with a speed v0. A particle on the rim of the wheel at the same level as the centre will be moving at speed
View SolutionTwo semicircular rings of linear mass densities λ and 2λ and of radius 'R' each are joined to form a complete ring. The distance of the centre of the mass of complete ring from its geometrical centre is :
View Solution