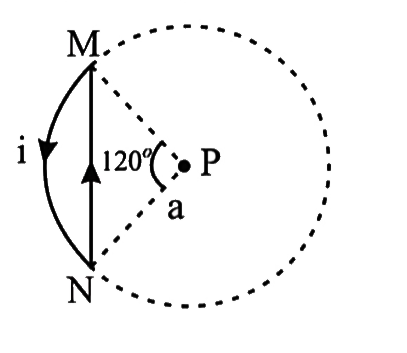
A wire loop carrying I is placed in the x−yplane as shown in fig.
(a) If a particle with charge +Q and mass m is placed at the centre P and given a velocity →v along NP(see figure), find its instantaneous acceleration.
( b) If an external uniform magnetic induction field →B=Bˆi is applied , find the force and the torque acting on the loop due to this field.
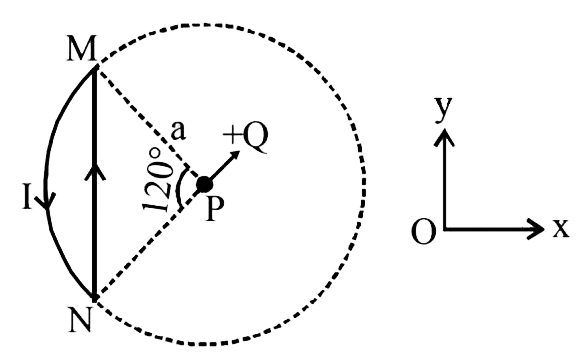
The magnetic field at the centre P due to current in wire NM is

B1=μ02πIr[sin60∘+sin60∘]
B1=μ04πIa/2[√32+√32],B1=μ04π2I√3a
Directed away from the reader perpendicular to the plane of paper sin30∘=ra⇒r=a2
cos30∘=MSa∴MS=√3a2∴MN=√3a
The magnetic field at the centre P due to current in are MN is
B2=μ02π2πla(θ2π)=μ04π2πla[2π/32π]=μ04π×2πl3a
Directed towards the reader perpendicular to the planeof paper. The net magnetic field
B=B1−B2=μ04πa2√3la−μ04π2πl3a=μ02I4πa[√3π3π]=μ04π×2Ia×(.68)
( Directed away fromt he reader perpendicular to the plane of paper ). The force acting on the charged particle Q when it has a velocity v and is instantaneously at the centre is
F=QvBsinθ=QvBsin90∘=QvB
The acceleration produced
A=FM=QvBm=Qvm[μ04π×2Ia×(0.68)],A=0.11μ0IQvma

The direction of acceleration is given by the vector product →v×→B or by applying Fleming's left hand rule ∠RPN=90∘ and ∠MPN=120∘
∴∠MPR=120−90=30∘
Since ∴∠MPQ=60∘∴∠RPQ=30∘
i.e. the acceleration vector makes an angle of 30∘ with the negative x− axis
(b) The Torque acting on the loop in the magnetic field is given by →τ=→M×→B
Where M=IA where A=( Area of PMQNP )−( area of trianle PMN)
=13(πa2)12×MN×PS=πa2312×√3a×a2=a2[π3√34]
→A=a2[π3−√34]ˆk ∴→τ=Ia2[π3−√34]ˆk׈iB,
∴→τ=Bla2[π3−√34]ˆj=0.614Bia2ˆJ
The force acting on the loop is zero u=V0
a=−ILBmV=0 Using
V2−u2=2as⇒V20=2ILBmS⇒S=mV202ILB
Topper's Solved these Questions
- MOVING CHARGES AND MAGNETISMBook:NARAYNAChapter:MOVING CHARGES AND MAGNETISMExercise:Level =I (H.W)
Explore 42 Videos
- MOVING CHARGES AND MAGNETISMBook:NARAYNAChapter:MOVING CHARGES AND MAGNETISMExercise:Level -II (H.W)
Explore 37 Videos
- MOVING CHARGES AND MAGNETISMBook:NARAYNAChapter:MOVING CHARGES AND MAGNETISMExercise:LEVEL-V (SINGLE ANSWER QUESTION)
Explore 85 Videos
- MAGNETISM AND MATTERBook:NARAYNAChapter:MAGNETISM AND MATTERExercise:EXERCISE - 4 (SINGLE ANSWER TYPE QUESTION)
Explore 17 Videos
- NUCLEAR PHYSICSBook:NARAYNAChapter:NUCLEAR PHYSICSExercise:LEVEL-II-(H.W)
Explore 9 Videos
Similar Questions
A wire loop carrying I is placed in the x−yplane as shown in fig.
(a) If a particle with charge +Q and mass m is placed at the centre P and given a velocity →v along NP(see figure), find its instantaneous acceleration.
( b) If an external uniform magnetic induction field →B=Bˆi is applied , find the force and the torque acting on the loop due to this field.

View SolutionA wire loop carrying a current I is placed in the xy-plane as shown in figure
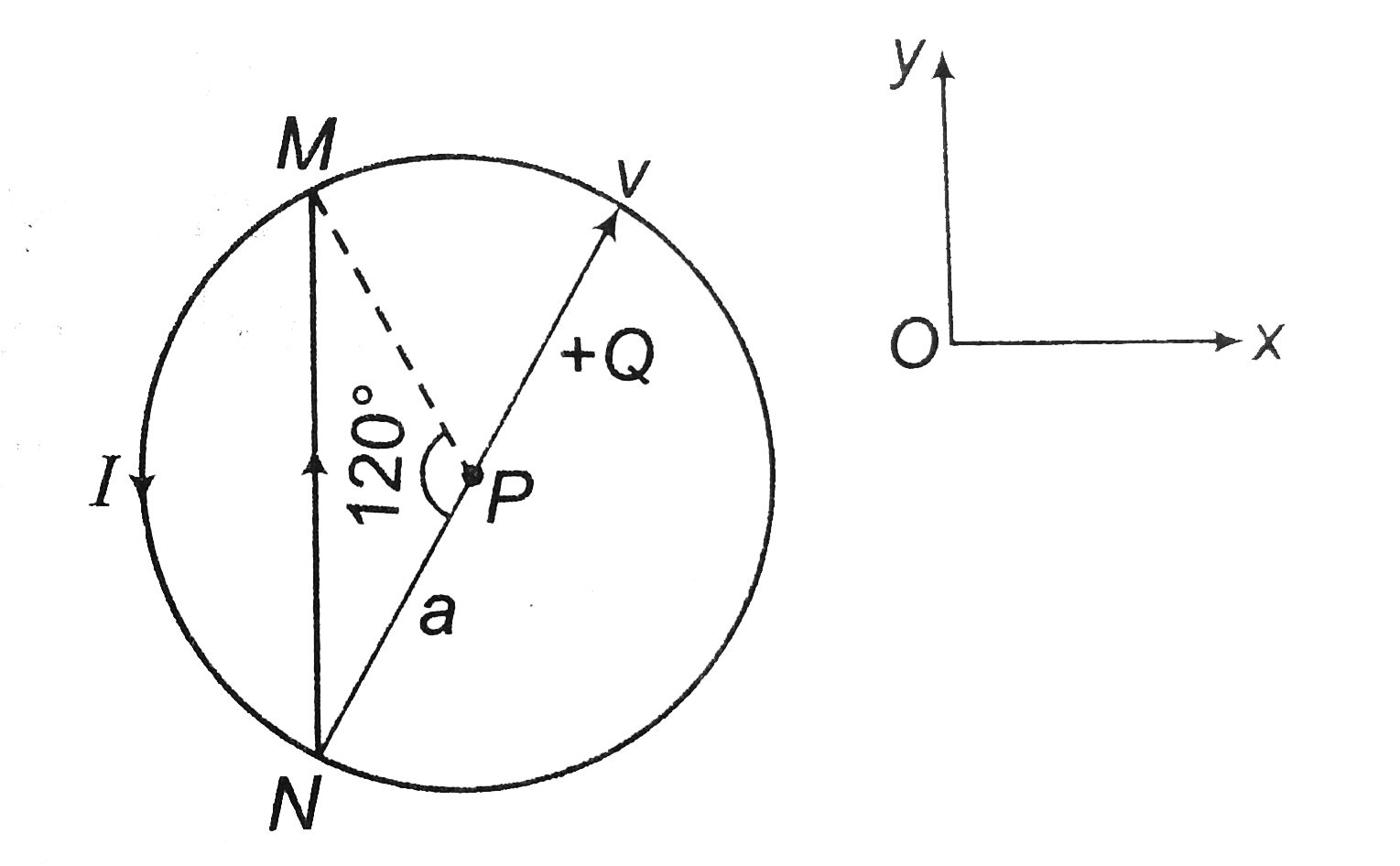
a. If a particle chsarge +Q and mass m is placed at in centre P and given a velocity v along NP (see figure), find its instaneous acceleration.
If an external uniform magnetic induction field B=Bˆi is applied, find the force and the torque acting on the loop due to this field.
View Solution
Knowledge Check
A wire loop carrying current I is placed in the x-y plane as shown. Magnetic induction at P is
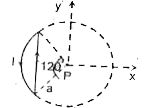 Aμ0I2a(√3π−13)Bμ0I2a(13−π√3)Cμ0I2a(13+√3π)Dμ0I2a(√3π−13)
Aμ0I2a(√3π−13)Bμ0I2a(13−π√3)Cμ0I2a(13+√3π)Dμ0I2a(√3π−13)A dipole of magnetic moment →m=30ˆjAm2 is placed along the y-axis in a uniform magnetic field →B=(2ˆi+5ˆj)T. The torque acting on it is
A−40ˆkNmB−50ˆkNmC−60ˆkNmD−70ˆkNm
A wire loop carrying a current I is placed in the x-y plane. (a) If a particle with charge +Q and mass m is placed at the center P and given a velocity →v along NP, find its acceleration. (b) if an external uniform magnetic induction →B=Bˆi is applied, find the force and the torque acting on the top due to this field.
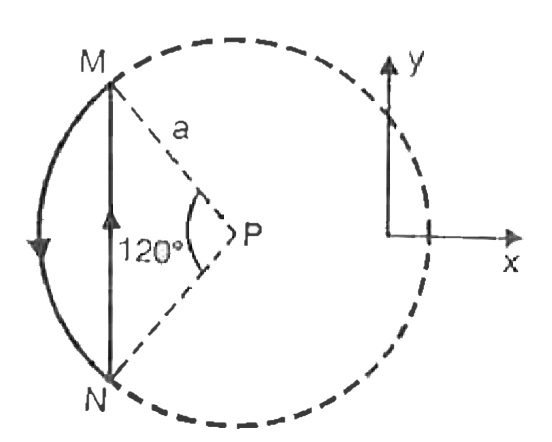
View SolutionA wire loop ABCDE carrying a current I is placed in the x−y plane as shown in the figure. A particle of mass m and charge q is projected from origin with velocity
→V=V0√2(ˆi+ˆj)m/sec.
(a) Find the instaneous acceleration
(b) If an external magnetic field →B=B0ˆi is applied, find the force and torque acting on the loop due to this field.

View SolutionA wire loop carrying current I is placed in the x−y plane as shown in the figure. If an external uniform magnetic field →B=Bˆi is switched on, then the torque acting on the loop is
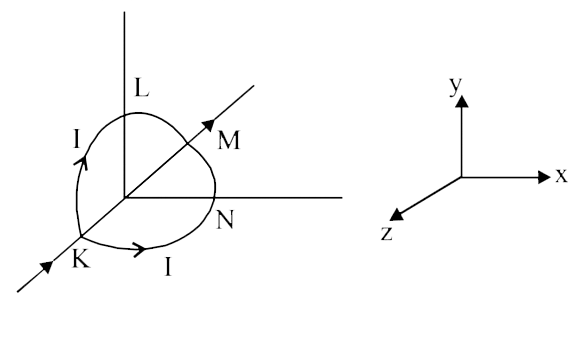
View SolutionA circular loop of radius R is bent along a diameter and given a shapes as shown in the figure. One of the semicircles (KNM) lies in the x−z plane with their centres and the other one (KLM) in the y−z plane with their centres at the origin. current I is flowing through each of the semi circles as shown in figure.
(a) A particle of charge q is released at the origin with a velocity →v=−v0ˆi. Find the instantaneous force →F on the particle . Assume that space is gravity free.
(b) If an external uniform magnetic field B0ˆj is applied , determine the force →F1and→F2 on the semicircles KLMandKNM due to the field and the net force →F on the loop.
View SolutionA circular loop of radius R is bent along a diameter and given a shape as shown in figure. One of 30,A the se micircles (KNM) lies in the xz-plane and the other one (KLM) in the yz-plane with their centres at origin. Current I is flowing through each of the semicircles as shown in figure.
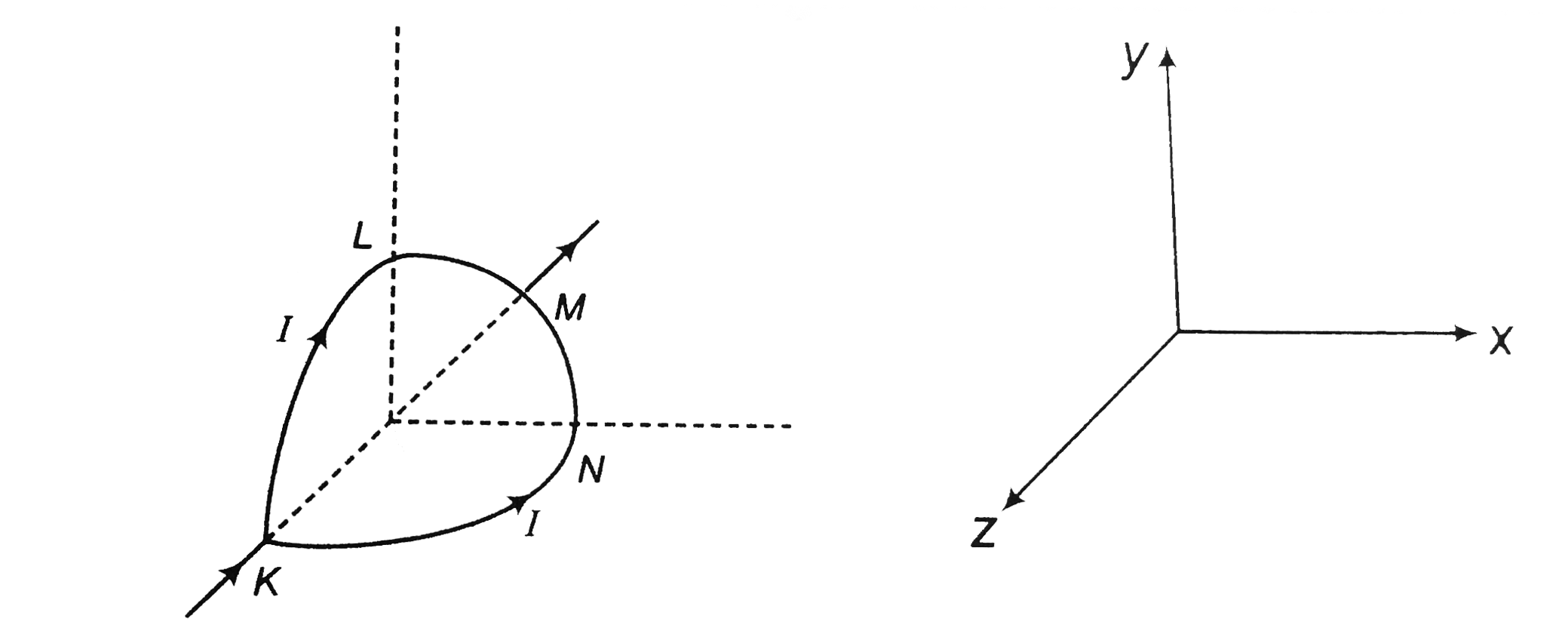
(a) A particle of charge q is released at the origin with a velocity v=−v0ˆi. Find the instantaneous force F on the particle. Assume that space is gravity free.
(b) If an external uniform magnetic field B0ˆj is applied, determine the force F1 and F2 on the semicircles KLM and KNM due to the field and the net force F on the loop.
View Solutionthe force on current carrying wire placed in uniform magnetic field B as shown in figure is

View Solution