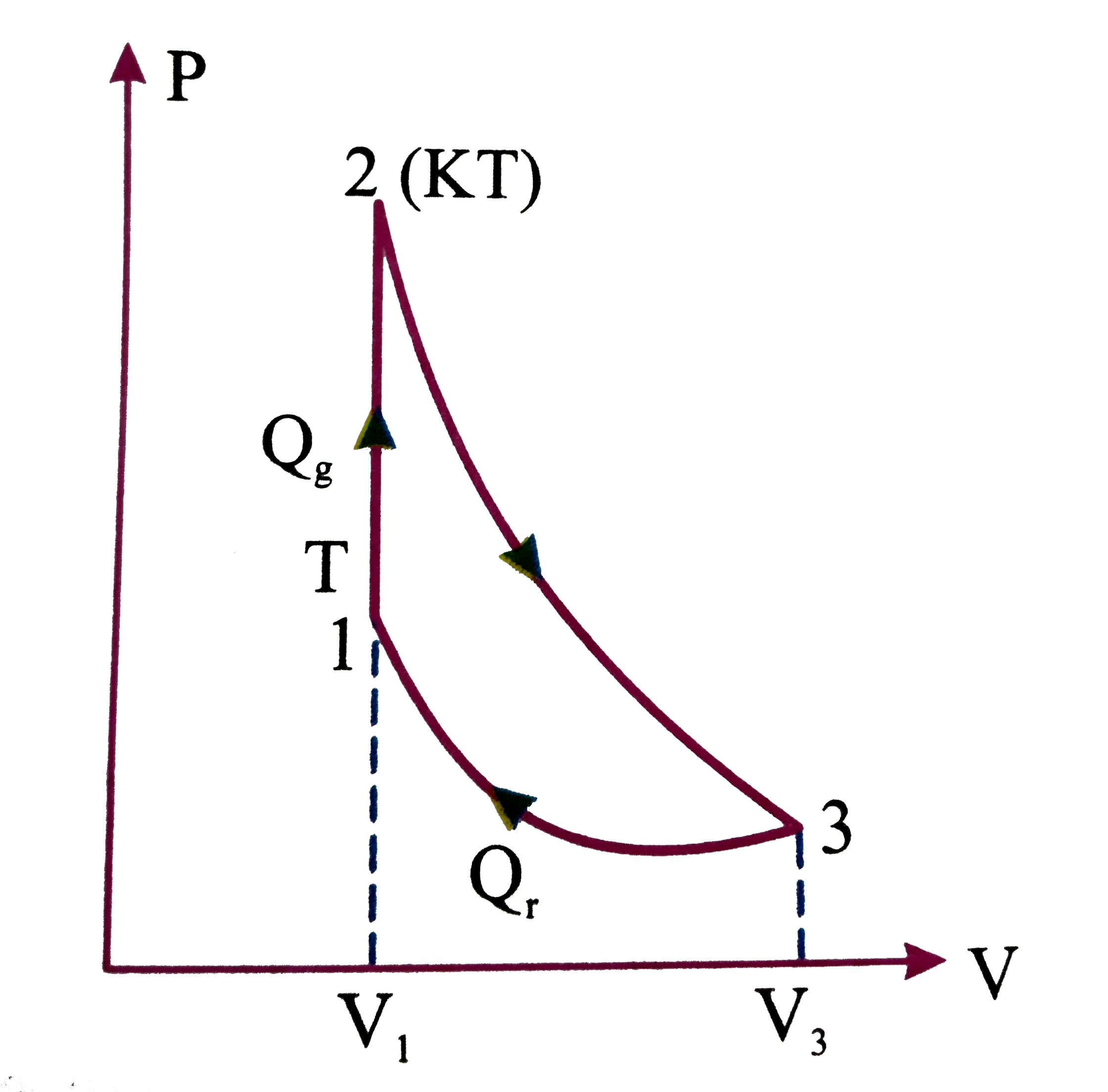
Find the efficiency of a cycle consisting of two isochoric and two isothermal lines if the volume varies $v - f o l d$ and the absolute temperature $τ - f o l d$ within the cycle. The working substance is an ideal gas with the adiabatic exponent $γ$ .

Video Solution

Text Solution

Verified by Experts

We have
$Q'_{1} = τ R T_{0} 1 n v, Q''_{2} = C_{V} T_{0} (τ - 1) Q_{1} = Q'_{1} + Q''_{1}$ and
$Q'''_{2} = R T_{0} 1 n v, Q''_{1} = C_{V} T_{0} (τ - 1)$
as well as $Q_{1} = Q'_{1} + Q_{1}''$ and
$Q_{2}' = Q_{2}'' + Q_{2}'''$
So $η = 1 - \frac{Q'_{2}}{Q_{1}} + 1 = \frac{C_{V} (τ - 1) + R 1 n v}{C_{V} (τ - 1) + τ R 1 n v}$
= $\frac{\frac{τ - 1}{γ - 1} + 1 n v}{\frac{τ - 1}{γ_{1}} + τ 1 n v} = \frac{(τ - 1) 1 n v}{τ 1 n v + \frac{τ - 1}{γ - 1}}$ .
.

|

Updated on:21/07/2023

Class 12 PHYSICS THERMODYNAMICS AND MOLECULAR PHYSICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The efficiency of the cycle shown below in the figure ( consisting of one isobar , one adiabatic and one isotherm ( is 50 % the ratio ,x, between the highest and lowest temperature attained in this cycle obeys ( the working substance is an ideal gas )

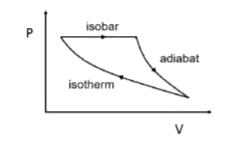A $x = e^{x - 1}$
B $x^{2} = e^{x - 1}$
C $x = e^{x^{2} - 1}$
D $x^{2} = e^{x^{2} - 1}$
Question 2 - Select One
An ideal gas goes through a cycle consisting of ischoric adiabatic and isothermal lines. The isothermal process is perform at minimum temperature. If the absolute temperature varies $K$ times with tn the cycle then find out its effcincy.

A $1 - \frac{ln K}{l}$
B $1 + \frac{ln K}{l}$
C $\frac{ln K}{l}$
D $\frac{l}{ln K}$

IE IRODOV, LA SENA & SS KROTOV-THERMODYNAMICS AND MOLECULAR PHYSICS-The Second Law Of Thermodynamics - Entropy

Home
Ask Doubt
Profile