One mole of a Van der Waals gas which had initially the volume V1 and the temperature T1 was transferred to the state with the volume V2 and the temperature T2. Find the corresponding entropy increment of the gas, assuming its molar heat capacity CV to be known.
We use, ΔS=∫T1,T2V1,T1dS(V,T)=∫T2T1(∂S∂T)V1dT+∫V1V1(∂S∂V)T=T2dV
=∫T2T1CVdTT+∫V2V1RV−bdV=CV1nT2T1+R1nV2−bV1−b
assuming CV,a,b to be known constant.
Topper's Solved these Questions
- THERMODYNAMICS AND MOLECULAR PHYSICSBook:IE IRODOV, LA SENA & SS KROTOVChapter:THERMODYNAMICS AND MOLECULAR PHYSICSExercise:Liquids Capillary Effects
Explore 25 Videos
- THERMODYNAMICS AND MOLECULAR PHYSICSBook:IE IRODOV, LA SENA & SS KROTOVChapter:THERMODYNAMICS AND MOLECULAR PHYSICSExercise:Phase Transformations
Explore 35 Videos
- THERMODYNAMICS AND MOLECULAR PHYSICSBook:IE IRODOV, LA SENA & SS KROTOVChapter:THERMODYNAMICS AND MOLECULAR PHYSICSExercise:Kinetic Theory Of Gases
Explore 51 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBook:IE IRODOV, LA SENA & SS KROTOVChapter:PHYSICAL FUNDAMENTALS OF MECHANICSExercise:Relativistic Mechanics
Explore 49 Videos
Similar Questions
One mole of water being in equilibrium with a negligible amount of its saturated vapour at a temperature T1 was completely converted into saturated vapour at a temperature T2. Find the entropy increment of the system. The vapour is assumed to be an ideal gas, the specific volume of the liquid is negligible on comparsion with that of the vapour.
View SolutionOne mole of a certain gas is contained in a vessel of volume V=0.2501. At a temperature T1=300K the gas pressure is p1=90atm, and at a temperature T2=350K the pressure is p2=110atm. Find the Van der Walls parameters for this gas
View Solution
Knowledge Check
An ideal gas is found to obey and additional law VP2 = constant. The gas is initially at temperature T and volume V . When it expands to a volume 2 V , the temperature becomes
A√2TB2TC2√2TD4TTwo moles of a gas at temperature T and volume V are heated to twice its volume at constant pressure. If CpCv=γ then increase in internal energy of the gas is-
ARTγ−1B2RTγ−1C2RT3(γ−1)D2Tγ−1Which one of the following volume (V)- temperature (T) plots represents the behaviour of one mole of an ideal gas at one atmosphere?
A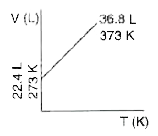 B
B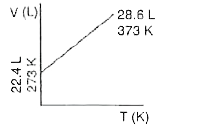 C
C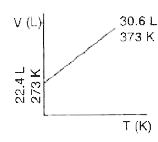 D
D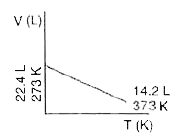
Two moles of a gas at temperature T and volume V are heated to twice its volume at constant pressure. If CpCv=γ then increase in internal energy of the gas is-
View SolutionFind the work performed by one mole of a Van der Walls gas during its isothermal expansion from the volume V1 to V2 at a temperature T.
View SolutionOne mole of an ideal gas goes through a process in which the entropy of the gas changes with temperature T as S=aT+CV1nT, where a is a positive constant. CV is the molar heat capacity of this gas at constant volume. Find the volume dependence of the gas temperature in this process if T=T0 at V=V0.
View SolutionFind the isothermal compressibility x of a Van der Walls gas as a function of volume V at temperature T
By definition, x=−1V∂V∂p.
View SolutionTen moles of a diatomic perfect gas are allowed to expand at constant pressure. The initial volume and temperature are V0 and T0 respectively. If 72RT0 heat is transferred to the gas, then the final volume and temperature are
View Solution