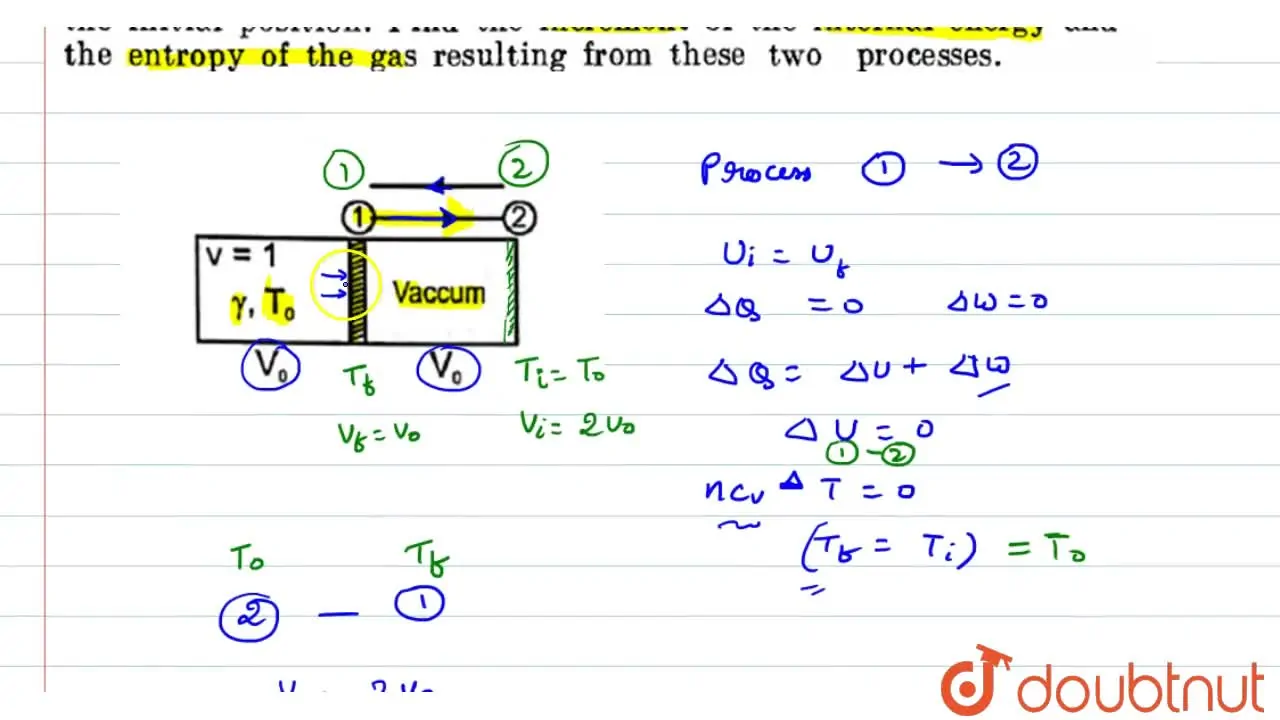
A weightless piston divides a thermally insulated cylinder into two equal parts. One part contains one mole of an ideal gas with adiabatic exponent γ, the other is evacuated. The initial gas temperature is T0. The piston is released and the gas fills the whole volume of the cylinder. Then the piston is slowly displaced back to the initial position. Find the increment of the internal energy and entropy of the gas was resulting from these two processes.
The process consists of two parts. The first part is free expansion in which Uf=Ui. The second part is adiabatic compression in which work done results in change of internal energy. Obviously,
0=UF−Uf+∫V0VfpdV,Vf=2V0
Now in the first part pf=12p0,Vf=2V0, because there is no change of temperature.
In the second part, pVγ=12p0(2V0)γ=2γ−1p0Vγ0
∫V02V0pdV=∫V02V02γ−1p0Vγ0VγdV=[2γ−1p0Vγ0−γ+1V1−γ]V02V0
=2γ−1p0Vγ0V−γ+102−γ+1−1γ−1=−(2γ−1−1)γ−1RT
Thus ΔU=UF−Ui=RT0γ−1(2γ−1−1)
The entropy change ΔS=ΔS1+ΔSII
ΔSI=R1n2 and ΔSII=0 as the process is reversible adiabatic. Thus ΔS=R1n2.
Related Playlists
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Liquids Capillary Effects25videos
THERMODYNAMICS AND MOLECULAR PHYSICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Phase Transformations35videos
THERMODYNAMICS AND MOLECULAR PHYSICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Kinetic Theory Of Gases51videos
THERMODYNAMICS AND MOLECULAR PHYSICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Relativistic Mechanics49videos
PHYSICAL FUNDAMENTALS OF MECHANICS
Similar Questions
Find the capillary pressure
(a) in mercury droplets of diameter d=1.5μm ,
(b) inside a soap bubble diameter d=3.0mm if the surface tension of the soap water solution is α=45mN/m.04:50View SolutionA saturated water vapour is contained in a cylindrical vessel under a weightless piston at a temperature t=100∘C. As a result of a slow introduction of the piston a small fraction of the vapour Δm=0.70g gets condensed. What amount of work was perfomed over the gas ? The vapour is assumed to be ideal, the volume of the liquid is to be neglected.
10:10View SolutionModern vacuum pumps permit to pressures down to p=4.10−15atm to be reached at room temperatures. Assuming that the gas exhausted is nitrogen, find the number of its molecules per 1cm3 and the mean distance between them at this pressure.
08:01View SolutionA rod moves lengthwise with a constant velocity v relative to the inertial reference frame K. At what value of v will the length of the rod in this frame be η=0.5% less than its proper length?
10:57View SolutionA masslesss piston divides a closed thermallyy insulated cylinder into two equal parts. One part contains M = 28 g of nitrogen. At this temperature, one-third of molecules are dissociated into atoms and the other part is evacuated. The piston is released and the gas fills the whole volume of the cylinder at temperature T0. Then, the piston is slowly displaced back to its initial position. calculate the increases in internal energy of the gas. Neglect further dissociation of molecules during, the motion of the piston.
08:45View SolutionA heat-conducting piston can freely move inside a closed thermally insulated cylinder with an ideal gas. In equilibrium the piston divides the cylinder into two equal parts, the gas temperature being equal to T0. The piston is slowly displaced. Find the gas temperature as a function of the ratio η of the volumes of the greater and smaller sections. The adiabatic exponent of the gas is equal to γ.
06:51View SolutionA piston divides a closed gas cylinder into two parts. Initially the piston is kept pressed such that one part has a pressure P and volume 5V and the other part has pressure 8P and volume V, the piston is now left free. Find the new pressure and volume for the isothermal and aidabatic process. (γ=1.5)
View SolutionA weightless piston divides a thermally insulated cylinder into two parts of volumes V and 3V.2 moles of an ideal gas at pressure P=2 atmosphere are confined to the part with volume V=1 litre. The remainder of the cylinder is evacuated. The piston is now released and the gas expands to fill the entire space of the cylinder. The piston is then pressed back to the initial position. Find the increase of internal energy in the process and final temperature of the gas. The ratio of the specific heats of the gas, γ=1.5.
11:34View SolutionA diathermic piston divides adiabatic cylinder of volume V0 into two equal parts as shown in the figure. Both parts contain ideal monoatomic gases. The initial pressure and temperature of gas in left compartment are P0 and T0 while that in right compartment are 2P0 and 2T0. Initially the piston is kept fixed and the system is allowed to acquire a state of thermal equilibrium.
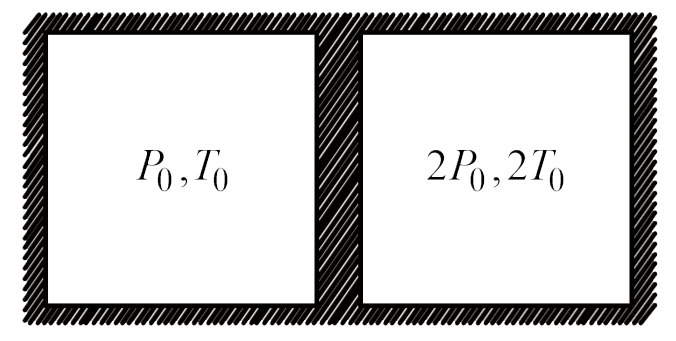
If the pin which was keeping the piston fixed is removed and the piston is allowed to slide slowly such that a state of mechanical equilibrium is achieved. The volume of left compartment when piston is in equilibrium isView SolutionA mass less piston divides a thermally insulated cylinder into two parts having volumes V = 2.5 litre and 3 V = 7.5 litre. 0.1 mole of an ideal gas is confined into the part with volume V at a pressure of P = 10^(5) N//m^(2) . The other part of the cylinder is empty. The piston is now released and the gas expands to occupy the entire volume of the cylinder. Now the piston is pressed back to its initial position. Find final temperature of the gas. [Take R = (25)/(3) J mol^(-1) K^(-1) and gamma = 1.5 for the gas]
08:29View SolutionA heat conducting piston can move freely inside a closed thermally isolated cylinder which contains an ideal gas. In equilibrium the piston divides the cylinder in two equal parts, the temperature of the gas being T_(0) . The piston is slowly displaced. Find the temperature of the gas when the volume of one part is n times greater than that of the other part. The adiabatic exponent of the gas is gamma .
04:59View Solutionएक पिस्टन किसी गैस सिलिंडर को दो भागो में विभाजित करता है | प्रारंभ में पिस्टन को ऐसे दबाते है जिससे एक भाग में दाब P तथा आयतन 5V है और दूसरे भाग में 8P दाब तथा आयतन V है | अब पिस्टन को स्वतंत्र छोड़ दिया जाता है , रुद्धोष्म तथा समतापी प्रक्रमों के लिए नये दाब तथा आयतन की गणना कीजिये ( गैस के लिए γ=1.5 )
View SolutionA heat conducting piston can move freely inside a closed, thermally insulated cylinder with an ideal gas (γ= 5/3). At equilibrium, the piston divides the cylinder into two equal parts, the gas temperature being equal to 300 K. The piston is slowly displaced by an external agent. Find the gas temperature when the volume of the greater section is seven times the volume of the smaller section.
View SolutionA cylindrical vessel made of thermally insulating material is divided into two equal parts by an insulating and. Movable piston. Both parts contain ideal monoatomic gas . The gas in the left part is supplied heat such that the volume of right part becomes one-eight of its initial volume . Work done by the gas in the right part is
 09:49View Solution
09:49View Solutionएक-परमाणुक आदर्श गैस प्रारम्भिक ताप T3 पर, एक पिस्टन युक्त सिलिण्डर में भरी है। पिस्टन को अचानक स्वतंत्र करके गैस को रुद्धोष्म रूप से, ताप तक प्रसारित होने देते हैं। यदि सिलिण्डर में गैस के प्रसार के पहले एवं बाद में गैस स्तम्भों की लम्बाईयाँ क्रमश: L1 तथा L2 हैं, तब T1/T2 का मान है
10:36View Solution