Vessels 1 and 2 contain v=1.2 moles of gaseous helium. The ratio of the vessels' volumes V2/V1=α=2.0, and the ratio of the absolute temperatures of helium in them T1/T2=β=1.5. Assuming the gas be to ideal, find the difference of gas entropies in these vessels, S2−S1.
To calculate the required entropy difference we only have to calculate the entropy difference for a process in which the state of the gas in vessel 1 is changed to that in vessel 2
ΔS=v⎛⎜⎝∫T1αβT1CVdTT+∫T1βT1αβCpdTT⎞⎟⎠
=v(Cp1nα−CV1nαβ)
=v(R1nα−Rγ−11nβ)=vR(1nα−1nβγ−1)
With γ=53,α=2 and β=1.5,v=1.2,
this gives ΔS=0.85Joule/.∘K.
 .
.
Topper's Solved these Questions
- THERMODYNAMICS AND MOLECULAR PHYSICSBook:IE IRODOV, LA SENA & SS KROTOVChapter:THERMODYNAMICS AND MOLECULAR PHYSICSExercise:Liquids Capillary Effects
Explore 25 Videos
- THERMODYNAMICS AND MOLECULAR PHYSICSBook:IE IRODOV, LA SENA & SS KROTOVChapter:THERMODYNAMICS AND MOLECULAR PHYSICSExercise:Phase Transformations
Explore 35 Videos
- THERMODYNAMICS AND MOLECULAR PHYSICSBook:IE IRODOV, LA SENA & SS KROTOVChapter:THERMODYNAMICS AND MOLECULAR PHYSICSExercise:Kinetic Theory Of Gases
Explore 51 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBook:IE IRODOV, LA SENA & SS KROTOVChapter:PHYSICAL FUNDAMENTALS OF MECHANICSExercise:Relativistic Mechanics
Explore 49 Videos
Similar Questions
Two thermally insulated vessels are interconnected by a tube equipped with a valve. One vessel volume V1=101 contains v=2.5 moles of carbon dioxide. The other vessel of volume V1=1001 is evacuated. The valve having been opened, the gas adiabatically expanded. Assuming the gas to obey the Van der Walls equation, find its temperature change accompanying the expansion.
View SolutionA vessel of volume V contains n1 moles of oxygen and n2 moles of carbon dioxide at temperature T. Assuming the gases to be ideal, find,
(a) the pressure of the mixture,
(b) the mean molar mass of the mixture.
View Solution
Knowledge Check
n moles of helium gas are placed in a vessel of volume V Litre, at T K. If V1 is free volume of helium then diameter of He atom is:
A[32V1πNAn]1/3B[3(V−V1)2πNAn]C[6(V−V1)πNAn]1/3D[6V1nNAn]1/3A vessel contains 1 mole of O2 at a temperature T. The pressure of gas is P. An identical vessel containing 1 mole of the gas at a temperature 2T has pressure of -
APB2PC3PD4PA vessel of volume 20L contains a mixture o hydrogen and helium at temperature of 27∘C and pressure 2.0atm The mass of the mixture is 5g. Assuming the gases to be ideal, the ratio of the mass of hydrogen to heat of helium in the given mixture will be
A1:2B2:3C2:1D2:5
Internal energy of n moles of helium at temperature T1K is equal to the internal energy of 2n moles of oxygen gas at temperature T2K then the value of T1T2will be
View SolutionTwo vessels of volume (V1)and(V2) contain the same ideal gas. The pressures in the vessels are (P1)and(P2) and the temperatures are (T1)and(T2) respectively . The two vessels are now connected to each other through a narrow tube. Assuming thar no heat is exchanged between the surrounding and the vessels, find the common pressure and temperature attained after the connection.
View SolutionOne mole of a certain gas is contained in a vessel of volume V=0.2501. At a temperature T1=300K the gas pressure is p1=90atm, and at a temperature T2=350K the pressure is p2=110atm. Find the Van der Walls parameters for this gas
View SolutionTwo vessels of the same volume contain the same gas at same temperature. If the pressure in the vessels is in the ratio of 1 : 2, then
View Solution1 mole of an ideal gas in a cylindrical container have the P-V diagram as shown in figure.If V2=4V1 then the ratio of temperatures T1T2 will be
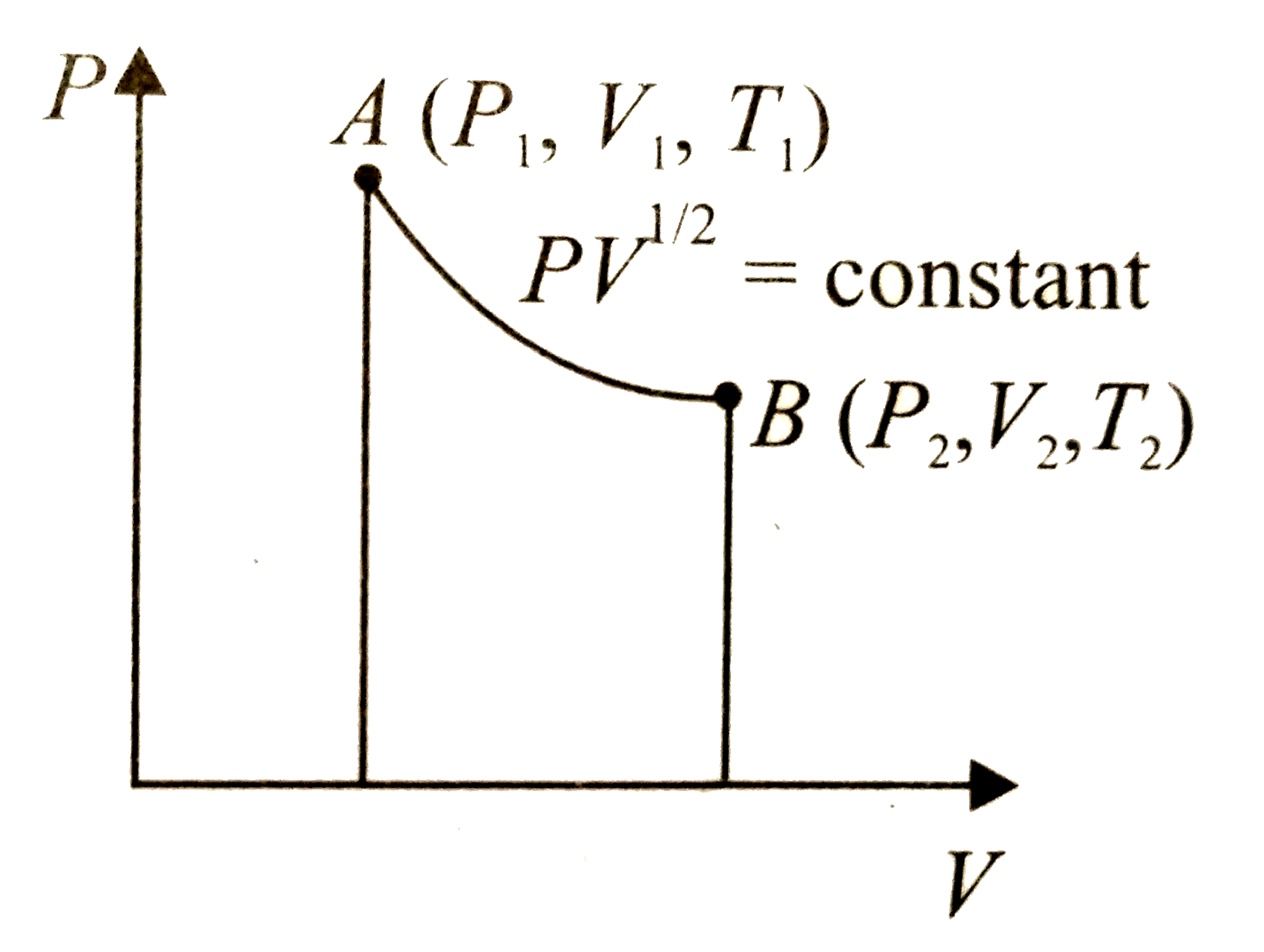
View Solution