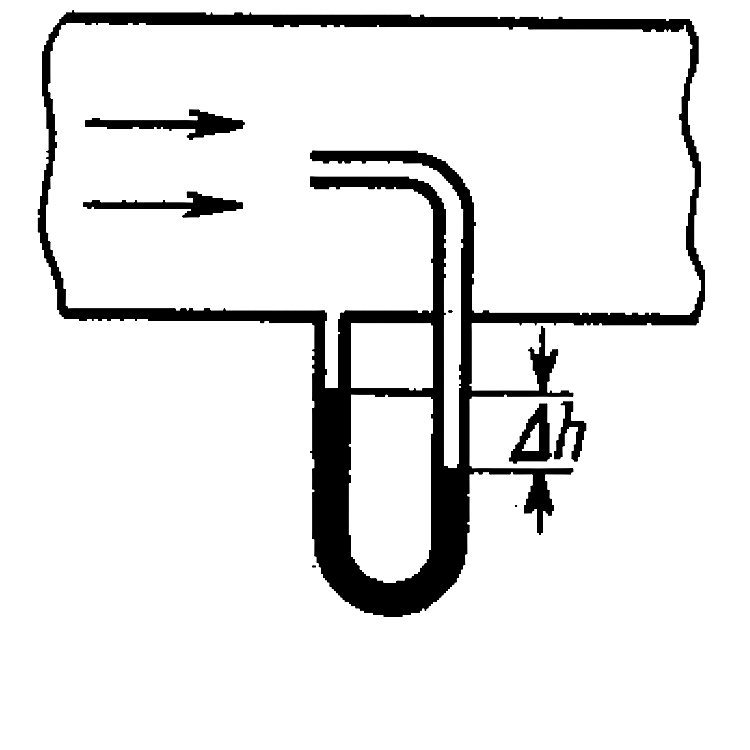
Two manometric tubes are mounted on a horizontal pipe of varying cross-section at the section S1 and S2 (figure). Find the volume of water flowing across the pipe's section per unit time if the difference in water columns is equal to Δh.

From the conservation of mass
v1S1=v2S2 (1)
But S1<S2 as shown in the figure of the problem, therefore
v1>v2
As every streamline is horizontal between 1 & 2, Bernoull's theorem becomes
p+12ρv2=constant, which gives
p1<p2 as v1>v2
As the difference in height of the water column is Δh, therefore
p2−p1=ρgΔh (2)
From Bernoull's theorem between points 1 and 2 a streamline
p1+12ρv21=p2+12ρv22
or, p2−p1=12ρ(v21−v22)
or ρgΔh=12ρ(v21−v22) (3) (using Eq. 2)
using (1) in (3), we get
v1=S2√2gΔhS22−S21
Hence the sought volume of water flowing per see
Q=v1S1=S1S2√2gΔhS22−S21

Topper's Solved these Questions
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Relativistic Mechanics
49 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Elastic Deformation Of Asolid Body
25 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation
36 Videos
- THERMODYNAMICS AND MOLECULAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Transport Phenomena
38 Videos
Similar Questions
A Pitot tube (figure) is mounted along the axis of a gas pipeline whose cross-sectional area is equal to S. Assuming the viscosity to be negligible, find the volume of gas flowing across the section of the pipe per unit time, if the difference in the liquid columns is equal to Δh, and the densities of the liquid and the gas are ρ0 and ρ respectively.
View SolutionA piot tube figure is mounted along the axis of a gas pipeline whose cross -sectional area is equal to S.
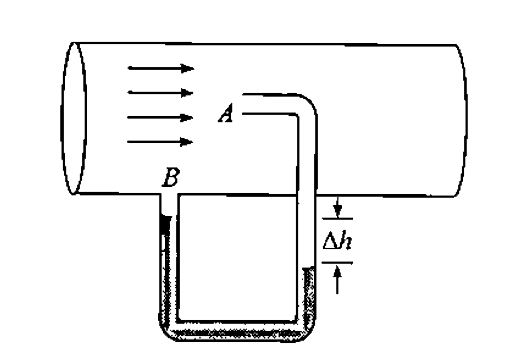
Assuming the viscosity to be negligible, find the volume of the gas flowing across the section of the pipe per unit time, if the difference in the liquid columns is equal to Δh, and the densities of the liquids and the gas are ρ0 and ρ respectively.
View Solution
Knowledge Check
A horizontal pipe of area of cross-section a and 3a respectively, then the ratio of velocity of flow at two different cross-section (If flow is streamline) is
A1:9B9:1C1:3D3:1
Water flows through a horizontal pipe of varying cross-section at the rate of 20 litres per minuts , determine the velocity of water at a point where diameter is 4cm
View SolutionA liquid flows through a horizontal pipe of varying cross section at a rate of 10 litres per minute. Find the velocity of liquid at a point where radius of pipe is 5 cm.
View SolutionWater flows through a horizontal pipe of varying area of cross section at the rate 15 cubic metre per minute. Find the radius of pipe where water velocity is 3 ms−1
View SolutionWater flows through a horizontal pipe of varying area of cross-section at the rate 5 cubic metre per minute. What is the velocity of water at a point where pipe radius is 10 cm?
View SolutionWater flow through horizontal pipe of varying cross-section at the rate of 1/2 liter per second. Determine the velocity of flow of water at a point where the diameter is (a) 4cm (b) 2cm.
View SolutionWater flows through a horizontal pipe of varying area of cross-section at the rate of 10 cubic metre per minute. Determine the velocity of water at a point where radius of pipe is 10cm.
View SolutionHow many litres of water flow out of a pipe having an area of cross-section of 5cm2 in one minute, if the speed of water in the pipe is 30 cm/sec ?
View Solution