The velocity distribution for the flow of a Newtonian fluid between two wide, parallel plates is given by the equation
![The velocity distribution for the flow of a Newtonian fluid between two wide, parallel plates is given by the equation u=(3V)/2[1-(y/h)^(2)] where V is the mean velocity. The fluid has coefficient of viscosity eta Answer the following 3 questions for this situation. Shear stress acting on the bottom wall is](https://d10lpgp6xz60nq.cloudfront.net/physics_images/RES_DPP_PHY_07_E01_040_Q01.png)
u=3V2[1−(yh)2]
where V is the mean velocity. The fluid has coefficient of viscosity η Answer the following 3 questions for this situation.
Shear stress acting on the bottom wall is
τbottom wall=η(3Vh)
τbottom wall=η(3V2h)
τbottom wall=η(6Vh)
τbottom wall=η(Vh)
For this type of parallel flow the shearing stress is given as ta=ηdudy....(i)
For the given distribution
dudy=−3Vyh2....(ii)
(a) Along the bottom wall so that (from eq. (ii))
dudy=3Vh and therefore the shearing stress is τbottom wall=η(3Vh)
(b) Along the plane where y=h/2 it follows from equation (ii) that
dudy=−3Vyh2 and thus the shearing stress is |τ|=η3V2h.
(C)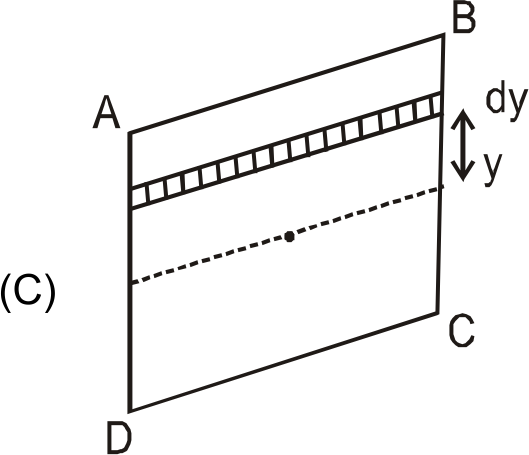 
Rate of volume flow
2∫h03V2(1−y2h2)dy.l=2Vlh
Topper's Solved these Questions
Similar Questions
Find the dimensions of the quantity v in the equation,
v=πp(a2−x2)2ηl
where a is the radius and l is he length of the tube in which the fluid of coefficient of viscosity η is flowing , x is the distacne from the axis of the tube and p is the pressure differnece.
View SolutionThe critical velocity of the flow of a liquid through a pipe of radius 3 is given by vc=(Kηrp), where p is the density and η, is the coefficient of viscosity of liquid. Check if this relation is dimentionally correct.
View Solution
Knowledge Check
The velocity distribution for the flow of a Newtonian fluid between two wide, parallel plates is given by the equation
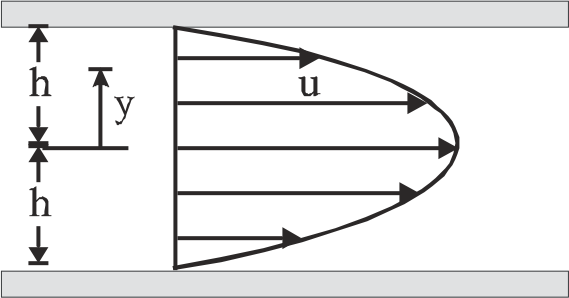
u=3V2[1−(yh)2]
where V is the mean velocity. The fluid has coefficient of viscosity η Answer the following 3 questions for this situation.
Consider a rectangular cross section of dimensions l x 2h as shown. The side AB is parallel to the plates. Volume flow rate through this cross section is
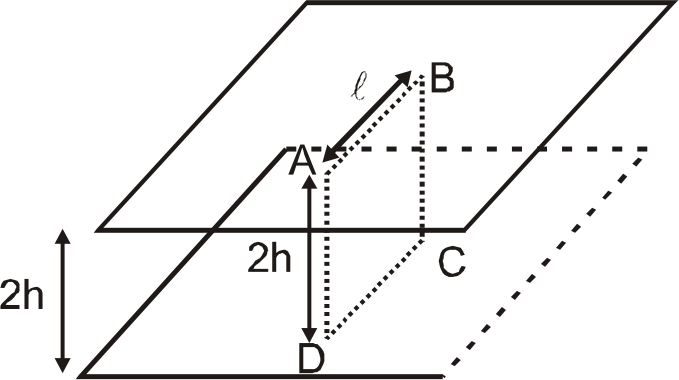AlhV/2BlhVC2lhVD3lhVThe velocity of a moving particle is given by the equation v=(5−t2) The average acceleration of the particle between the 2nd and 3rd seconds is
A−5ms−2B2ma−2C4ms−2D−4ms−2When a liquid flows in a tube, there is relative motion between adjacent layers of the liquid. This force is called the viscous force which tends to oppose the relative motion between the layers of the liquid. Newton was the first person to study the factors that govern the viscous force in a liquid. According to Newton’s law of viscous flow, the magnitude of the viscous force on a certain layer of a liquid is given by
F=−ηAdvdx
where A is the area of the layer dvdx is the velocity gradient at the layer and η is the coefficient of viscosity of the liquid.
A river is 5 m deep. The velocity of water on its surface is 2ms−1 If the coefficient of viscosity of water is 10−3Nsm−2 , the viscous force per unit area is :A10−4Nm−2B2×10−4Nm−2C4×10−4Nm−2D5×10−4Nm−2.
If the velocity of a particle "v" as a function of time "t" is given by the equation,v=a(1-e^(-bt) ,where a and b are constants,then the dimension of the quantity a^2*b^3 will be
View SolutionThe coefficient of viscosity (η) of a fluid moving steadily between two surface is given by the formula (f)=ηAdV/dx where f is the frictional force on the fluid, A is the area in the fluid, and dV/dx is velocity gradient inside the fluid at that area. The SI unit of viscosity is given as :
View SolutionWhen an object moves through a fluid, as when a ball falls through air or a glass sphere falls through water te fluid exerts a viscous foce F on the object this force tends to slow the object for a small sphere of radius r moving is given by stoke's law, Fw=6πηrv. in this formula η in the coefficient of viscosity of the fluid which is the proportionality constant that determines how much tangential force is required to move a fluid layer at a constant speed v, when the layer has an area A and is located a perpendicular distance z from and immobile surface. the magnitude of the force is given by F=ηAv/z. For a viscous fluid to move from location 2 to location 1 along 2 must exceed that at location 1, poiseuilles's law given the volumes flow rate Q that results from such a pressure difference P2−P1. The flow rate of expressed by the formula Q=πR4(P2−P1)8ηL poiseuille's law remains valid as long as the fluid flow is laminar. For a sfficiently high speed however the flow becomes turbulent flow is laminar as long as the reynolds number is less than approximately 2000. This number is given by the formula Re=2¯vρRη In which ¯v is the average speed ρ is the density η is the coefficient of viscosity of the fluid and R is the radius of the pipe. Take the density of water to be ρ=1000kg/m3
Q. Blood vessel is 0.10 m in length and has a radius of 1.5×10−2m blood flows at rate of 10−7m3/s through this vessel. The pressure difference that must be maintained in this flow between the two ends of the vessel is 20 Pa what is the viscosity sufficient of blood?
View SolutionWhen an object moves through a fluid, as when a ball falls through air or a glass sphere falls through water te fluid exerts a viscous foce F on the object this force tends to slow the object for a small sphere of radius r moving is given by stoke's law, Fw=6πηrv. in this formula η in the coefficient of viscosity of the fluid which is the proportionality constant that determines how much tangential force is required to move a fluid layer at a constant speed v, when the layer has an area A and is located a perpendicular distance z from and immobile surface. the magnitude of the force is given by F=ηAv/z. For a viscous fluid to move from location 2 to location 1 along 2 must exceed that at location 1, poiseuilles's law given the volumes flow rate Q that results from such a pressure difference P2−P1. The flow rate of expressed by the formula Q=πR4(P2−P1)8ηL poiseuille's law remains valid as long as the fluid flow is laminar. For a sfficiently high speed however the flow becomes turbulent flow is laminar as long as the reynolds number is less than approximately 2000. This number is given by the formula Re=2¯vρRη In which ¯v is the average speed ρ is the density η is the coefficient of viscosity of the fluid and R is the radius of the pipe. Take the density of water to be ρ=1000kg/m3
Q. If the sphere in previous question has mass of 1×10−5kg what is its terminal velocity when falling through water? (η=1×10−3Pa−s)
View SolutionWhen an object moves through a fluid, as when a ball falls through air or a glass sphere falls through water te fluid exerts a viscous foce F on the object this force tends to slow the object for a small sphere of radius r moving is given by stoke's law, Fw=6πηrv. in this formula η in the coefficient of viscosity of the fluid which is the proportionality constant that determines how much tangential force is required to move a fluid layer at a constant speed v, when the layer has an area A and is located a perpendicular distance z from and immobile surface. the magnitude of the force is given by F=ηAv/z. For a viscous fluid to move from location 2 to location 1 along 2 must exceed that at location 1, poiseuilles's law given the volumes flow rate Q that results from such a pressure difference P2−P1. The flow rate of expressed by the formula Q=πR4(P2−P1)8ηL poiseuille's law remains valid as long as the fluid flow is laminar. For a sfficiently high speed however the flow becomes turbulent flow is laminar as long as the reynolds number is less than approximately 2000. This number is given by the formula Re=2¯vρRη In which ¯v is the average speed ρ is the density η is the coefficient of viscosity of the fluid and R is the radius of the pipe. Take the density of water to be ρ=1000kg/m3
Q. Calculate the highest average speed that blood (ρ≈1000kg/m3) could have and still remain in laminar flow when it flows through the arorta (R=8×10−3m) Take the coeffiicient of viscosity of blood to be 4×10−3Pa−s
View Solution