A container of large uniform cross sectional area A resting on a horizontal surface holds two immiscible non-viscous and incompressible liquids of density d and 3d each of height H/2. The lower density liquid is open to the atmosphere having pressure Po. A tiny hole of area a (a<<A) is punched on the vertical side of the lower container at a height h(0<h<H2) for which range is maximum.
h=H/3
Range R=2H/3
Range R=3H/2
velocity of efflux r v=√23gH
To solve the problem, we need to find the height h at which the hole is punched in the lower liquid so that the range of the liquid that exits the hole is maximized.
1. Understanding the Setup:
- We have two immiscible liquids in a container. The lower liquid has density d and the upper liquid has density 3d.
- Both liquids have a height of H2.
- The lower liquid is open to the atmosphere at pressure P0.
2. Finding the Pressure at the Hole:
- The pressure at a depth h in the lower liquid can be calculated using the hydrostatic pressure formula:
P=P0+dgh
- The pressure at the hole is due to the column of liquid above it. The height of the liquid above the hole is H2+H2−h=H−h.
3. Velocity of the Liquid Exiting the Hole:
- According to Torricelli's theorem, the velocity v of the liquid exiting the hole can be given by:
v=√2P/ρ
- Substituting the pressure we found:
v=√2(P0+dg(H−h))d
4. Maximizing the Range:
- The range R of the liquid can be calculated using the formula:
R=v⋅t
- Where t is the time of flight. The time t for the liquid to fall from height h is given by:
t=√2hg
- Therefore, substituting v and t into the range equation:
R=√2(P0+dg(H−h))d⋅√2hg
5. Finding the Condition for Maximum Range:
- To find the maximum range, we need to differentiate R with respect to h and set the derivative to zero. This will involve some calculus.
- After differentiating and simplifying, we find that the range is maximized when:
h=H3
Final Answer:
The height h at which the hole should be punched for maximum range is:
h=H3
- We have two immiscible liquids in a container. The lower liquid has density d and the upper liquid has density 3d.
- Both liquids have a height of H2.
- The lower liquid is open to the atmosphere at pressure P0.
- The pressure at a depth h in the lower liquid can be calculated using the hydrostatic pressure formula:
P=P0+dgh
- The pressure at the hole is due to the column of liquid above it. The height of the liquid above the hole is H2+H2−h=H−h.
- According to Torricelli's theorem, the velocity v of the liquid exiting the hole can be given by:
v=√2P/ρ
- Substituting the pressure we found:
v=√2(P0+dg(H−h))d
- The range R of the liquid can be calculated using the formula:
R=v⋅t
- Where t is the time of flight. The time t for the liquid to fall from height h is given by:
t=√2hg
- Therefore, substituting v and t into the range equation:
R=√2(P0+dg(H−h))d⋅√2hg
- To find the maximum range, we need to differentiate R with respect to h and set the derivative to zero. This will involve some calculus.
- After differentiating and simplifying, we find that the range is maximized when:
h=H3
The height h at which the hole should be punched for maximum range is:
h=H3
Topper's Solved these Questions
Similar Questions
A container of large uniform cross sectonal area A, resting on horizontal surface, holds two immiscible non viscous and incompresible liquids of density d and 2d, each of height H2 as shown in the figure. Thelower density liquid is open to the atmospher having presure P0. A tiny hole of area (s(s>>A) is punched on the vertical side of the container at a height h(h>H2). Determine
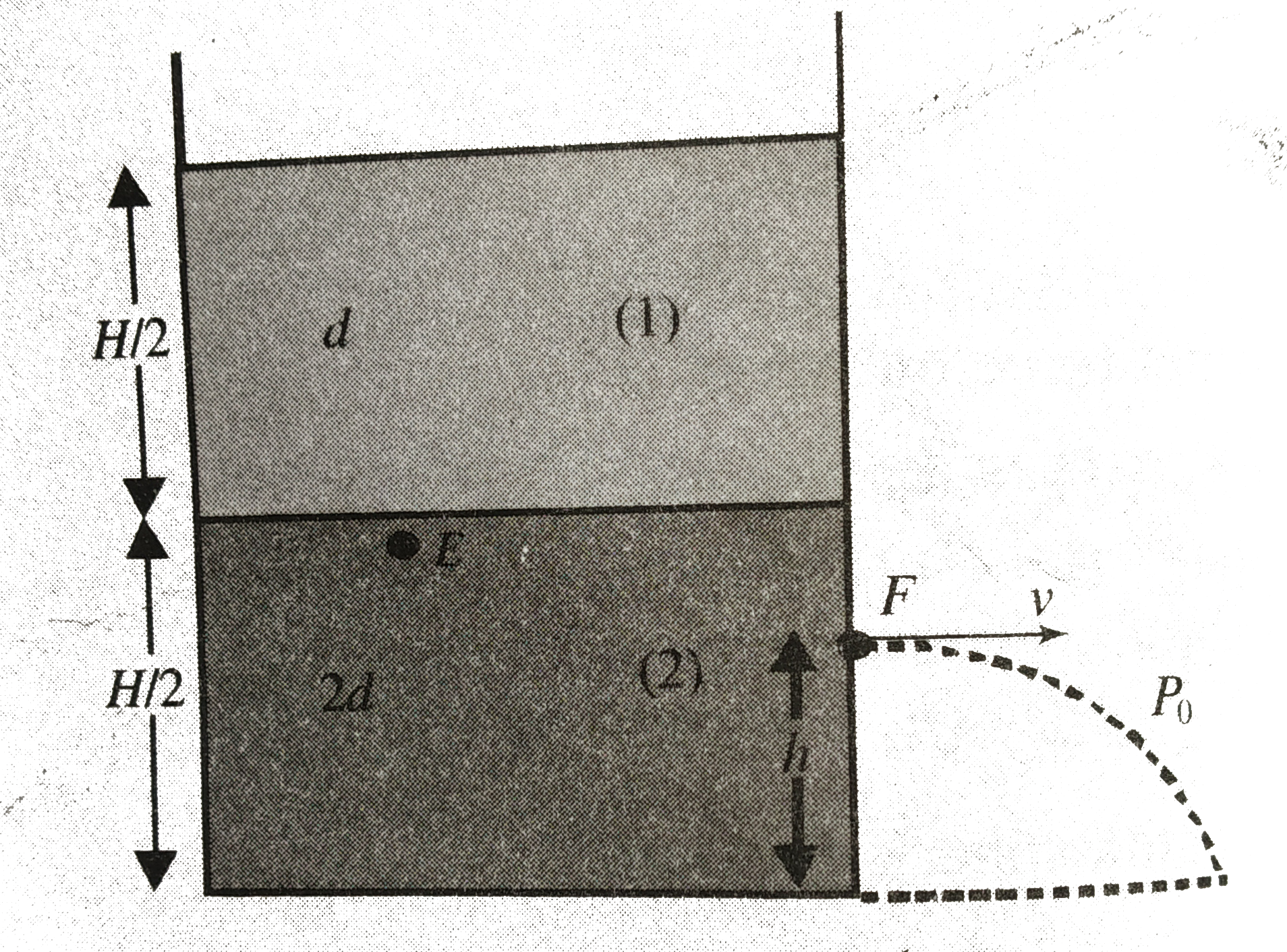
a.the initial speed of efflux of the liquid at the hole
b. the horizontal disance x travelled by the liquid initially
c. the height hm at which at the hole should be punched so that the liquid travels the maximum distance xm initially. also calculate xm (neglect air resistance inte calculations).
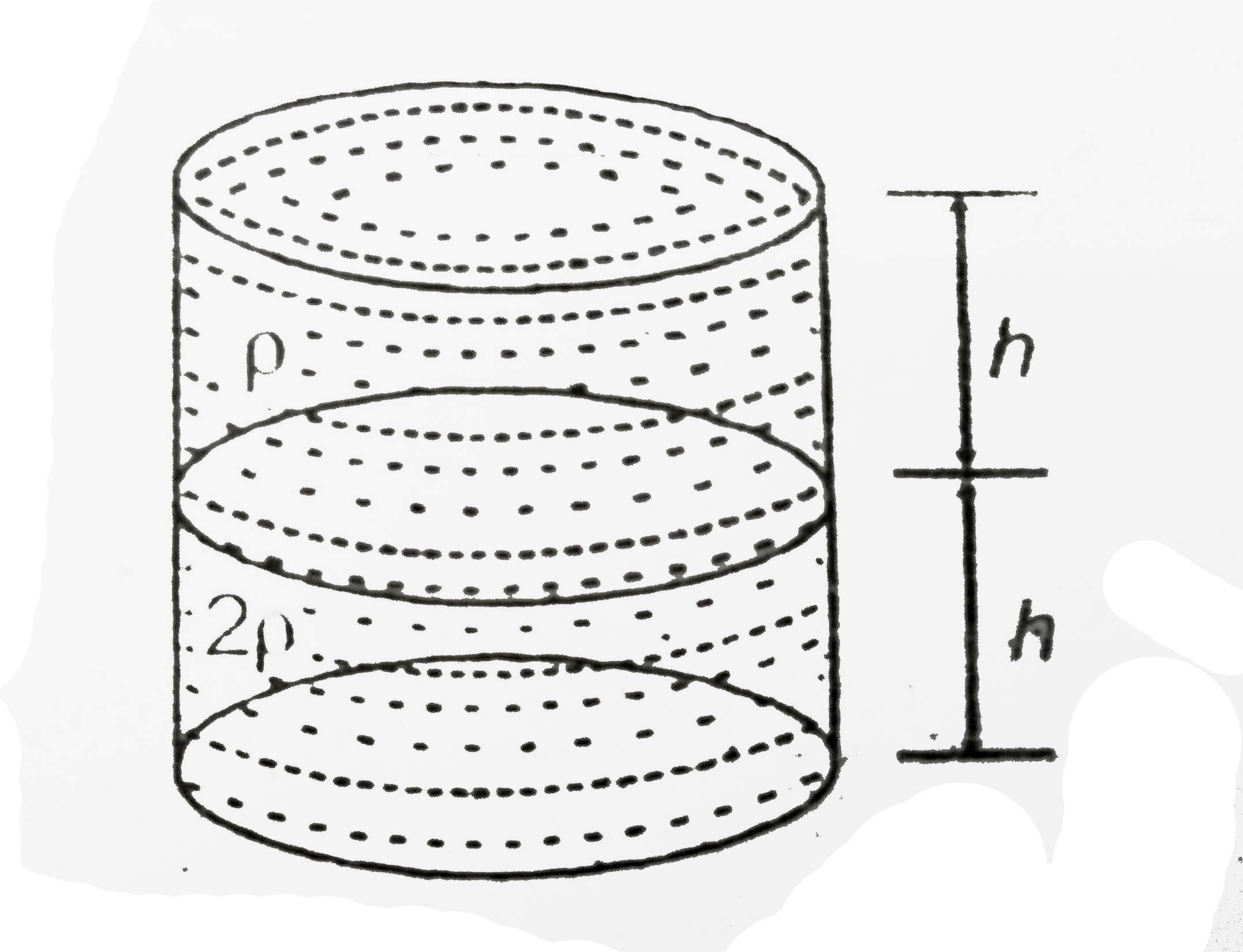
View SolutionA container of cross-section area A resting on a horizontal surface, holds two immiscible and incompressible liquid of densities ρand2ρ as shown in figure. The lower density liquid is open to the atmosphere having pressure P0. The pressure at the bottom of the container is
View Solution
Knowledge Check
Fluids at rest exert a normal force to the walls of the container or to the sruface of the body immersed in the fluid. The pressure exerted by this force at a point inside the liqid is the sum of atmospheric pressure and a factor which depends on the density of the liquid, the acceleration due to gravity and the height of the liquid, above that point. The upthrust acting on a body immersed in a stationary liquid is the net force acting on the body in the upward direction. A number of phenomenon of liquids in motion can be explain by Bernoulli's theorem which relates the pressure, flow speed and height for flow of an ideal incompressible fluid.
A container of large uniform cross sectional area. A resting on a horizontal surface holds two immiscible, non viscous and incompressile liquids of densities d and 2d, each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0.
Situation II:
A cyliner is removed and the original arrangement is restored.A tiny hole of area s(s<<A) is punched on the veritical sideof the containier at a height h(h<H/2)
The initial speed of efflux of the liquid at the hole isA√(4H−3h)g2B√(3H−4h)g2C(√(3H−3h)g2)D√(3H−3h)g2A container of large uniform cross-sectional area A resting on a horizontal surface, holes two immiscible, non-viscon and incompressible liquids of densities d and 2d each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0. A homogeneous solid cylinder of length L(L<H/2) and cross-sectional area A/5 is immeresed such that it floats with its axis vertical at the liquid-liquid interface with length L/4 in the denser liquid,
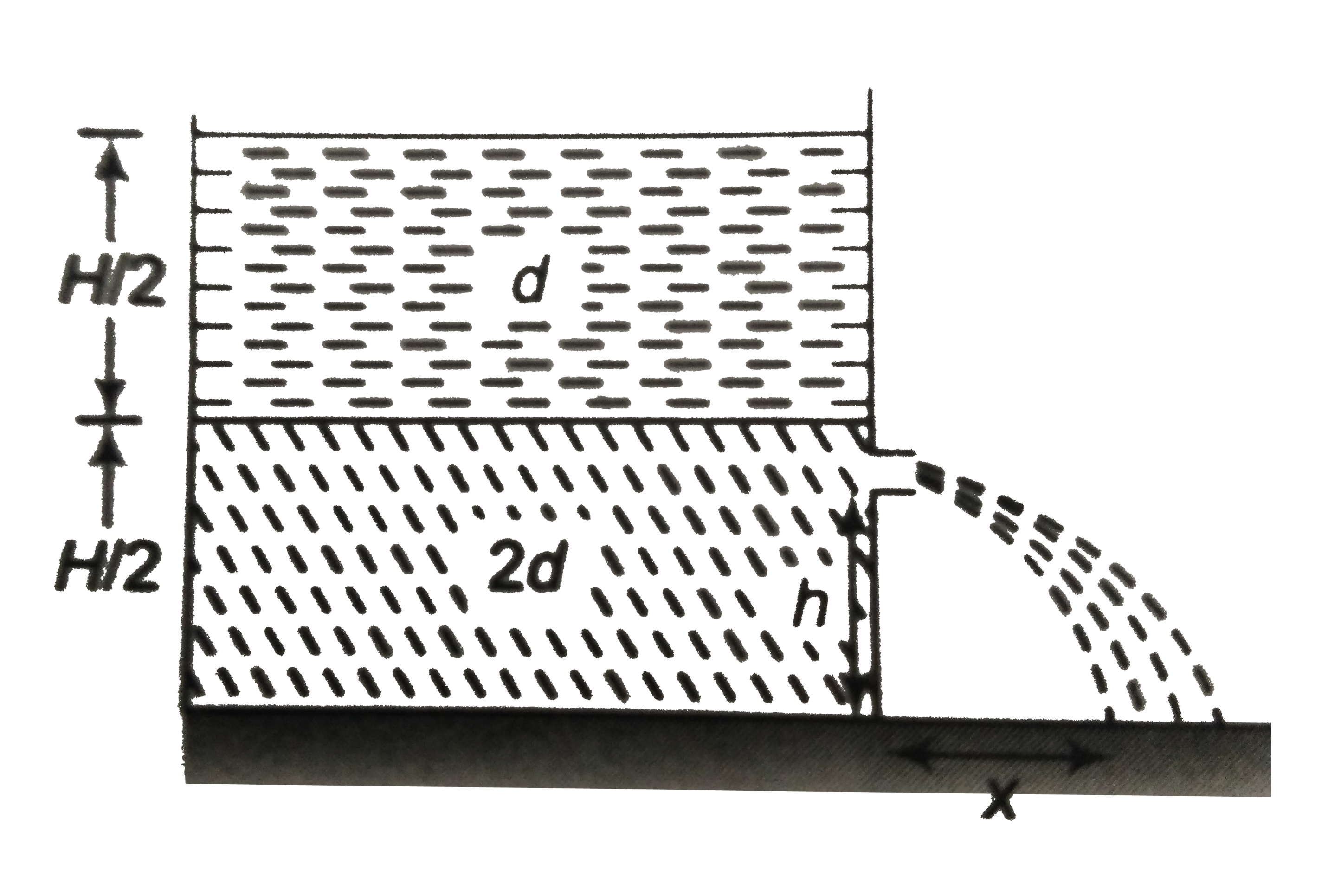
The cylinder is then removed and the original arrangement is restroed. a tiny hole of area s(s<<A) is punched on the vertical side of the container at a height h(h<H/2). As a result of this, liquid starts flowing out of the hole with a range x on the horizontal surface.
The density D of the material of the floation cylinder isA5d/4B3d/4C4d/5D4d/3A container of large uniform cross-sectional area A resting on a horizontal surface, holes two immiscible, non-viscon and incompressible liquids of densities d and 2d each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0. A homogeneous solid cylinder of length L(L<H/2) and cross-sectional area A/5 is immeresed such that it floats with its axis vertical at the liquid-liquid interface with length L/4 in the denser liquid,
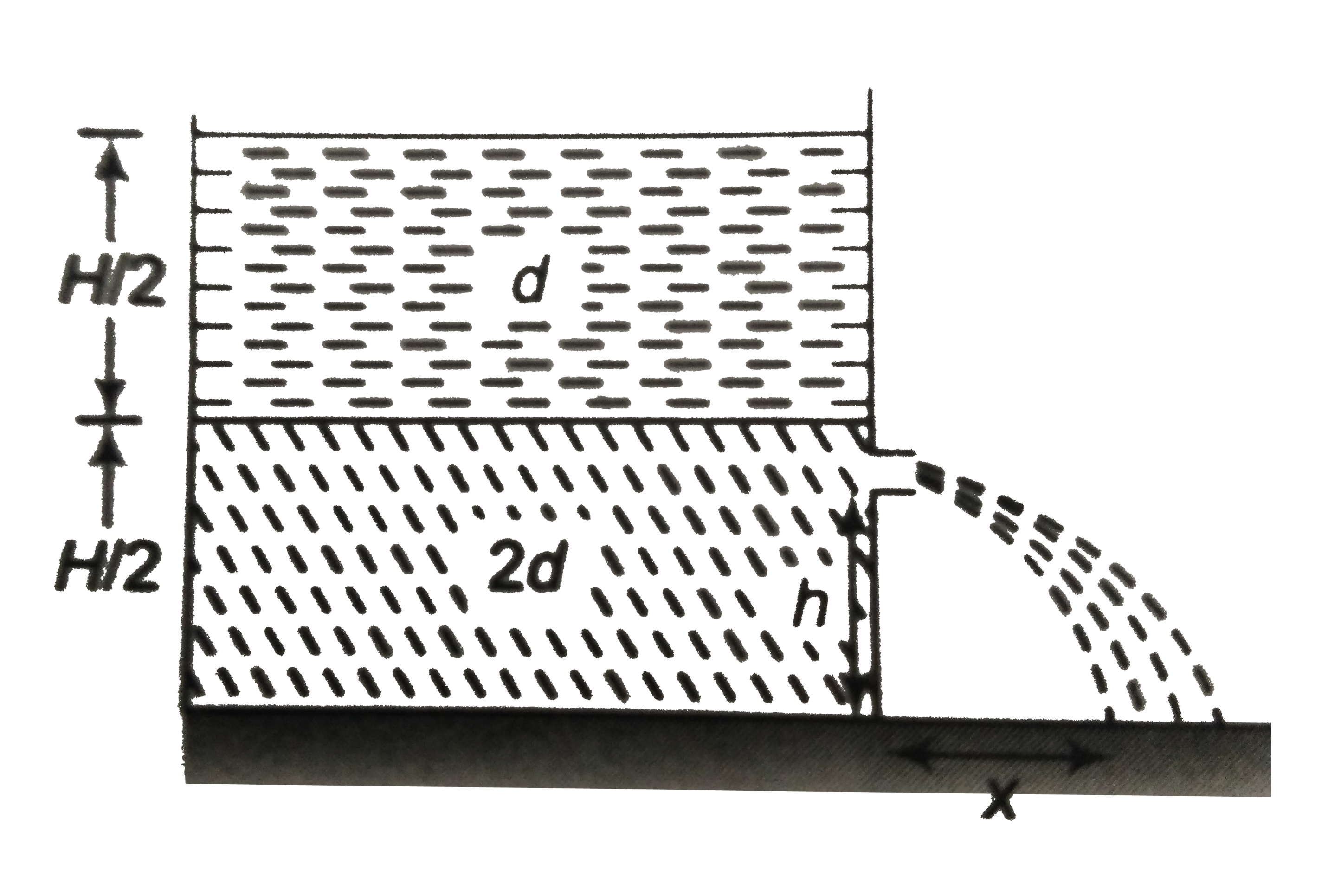
The cylinder is then removed and the original arrangement is restroed. a tiny hole of area s(s<<A) is punched on the vertical side of the container at a height h(h<H/2). As a result of this, liquid starts flowing out of the hole with a range x on the horizontal surface.
The total pressure with cylinder, at the bottom of the container isAp0+(6L+H)4dgBp0+(L+6H)4dgCp0+(L+3H)4dgDp0+(L+2H)4dg
A container of large uniform cross-sectional area A resting on a horizontal surface, holds two immiscible, non-viscous and incompressible liquids of densities d and 2d, each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0.
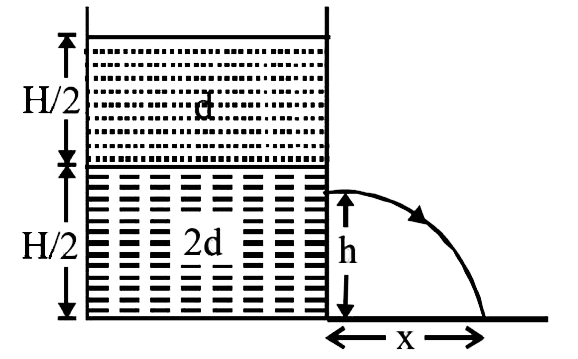
(a) A homogeneous solid cylinder of length L(L<H/2), cross-sectional area A/5 is immersed such that it floats with its axis vertical at the liquid-liquid interface with length L/4 in the denser liquid. Determine:
(i) the density D of the solid and
(ii) the total pressure at the bottom of the container.
(b) The cylinder is removed and the original arrangement is restored. A tiny hole of area s(s<<A) is punched on the vertical side of the container at a height h(h<H/2). Determine:
(i) the initial speed of efflux of the liquid at the hole,
(ii) the horizontal distance x travelled by the liquid initially, and
(iii) the height hm at which the hole should be punched so that the liquid travels the maximum distance xm initially. Also calculate xm.
View SolutionA container of large uniform cross-sectional area A resting on a horizontal surface, holds two-immiscible, non-viscous & incompressible liquids of densities d & 2d, each of height H/2. The lower density liquid is open to the atmoshpere having pressure P0. A homogeneous solid cylinder of length L(L<H/2) corss-sectional area A/5 is immersed such that it floats with its axis vertical at the liquid interface with the length L/4 in the denser liquid. Determine : (i) The density D of the solid & (ii) The total pressure at the bottom of the container.
View SolutionA container of large uniform cross-sectional area A resting on a horizontal surface, holes two immiscible, non-viscon and incompressible liquids of densities d and 2d each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0. A homogeneous solid cylinder of length L(L<H/2) and cross-sectional area A/5 is immeresed such that it floats with its axis vertical at the liquid-liquid interface with length L/4 in the denser liquid,
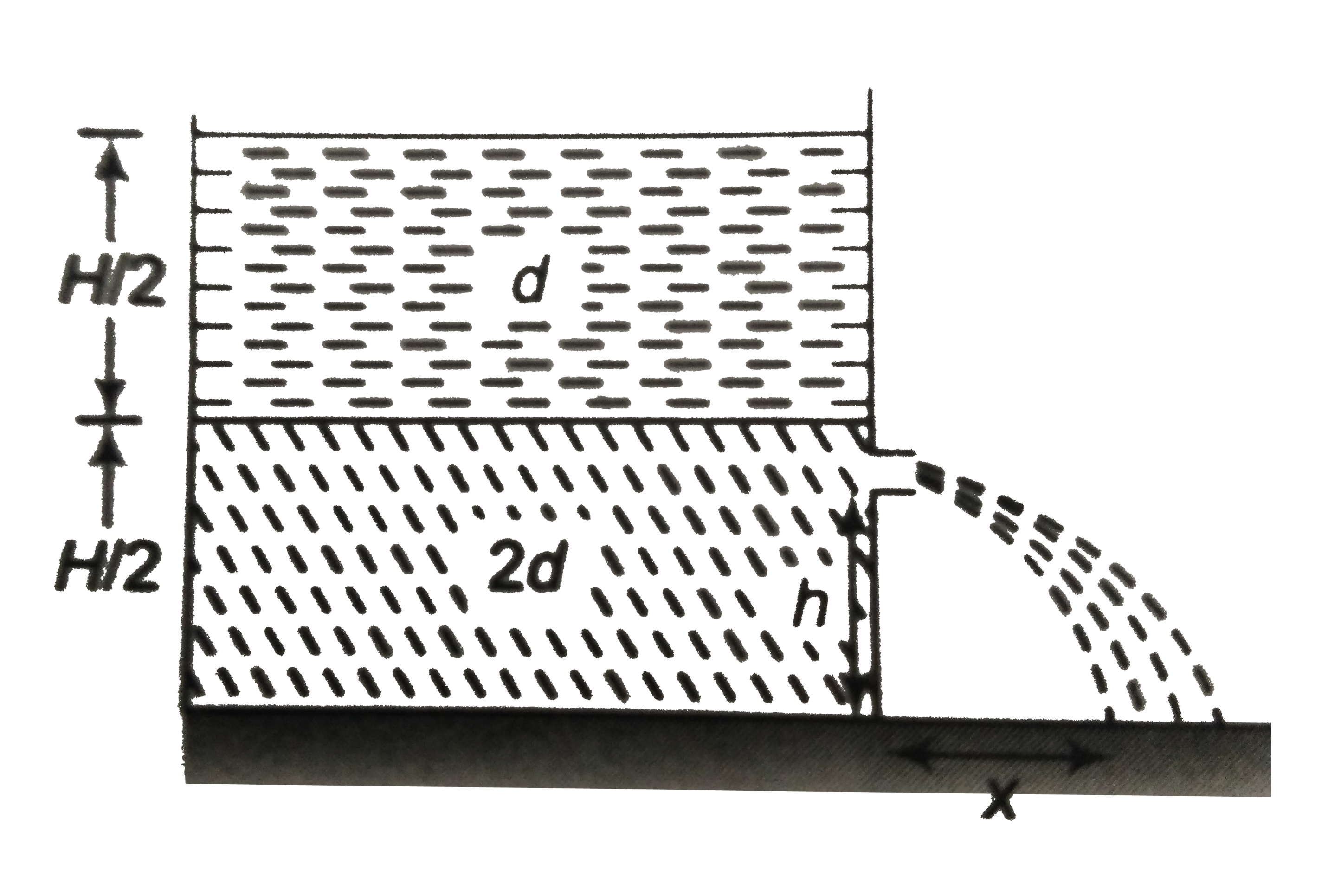
The cylinder is then removed and the original arrangement is restroed. a tiny hole of area s(s<<A) is punched on the vertical side of the container at a height h(h<H/2). As a result of this, liquid starts flowing out of the hole with a range x on the horizontal surface.
The initial speed of efflux without cylinder is
View SolutionA container of large uniform cross-sectional area A resting on a horizontal surface, holes two immiscible, non-viscon and incompressible liquids of densities d and 2d each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0. A homogeneous solid cylinder of length L(L<H/2) and cross-sectional area A/5 is immeresed such that it floats with its axis vertical at the liquid-liquid interface with length L/4 in the denser liquid,
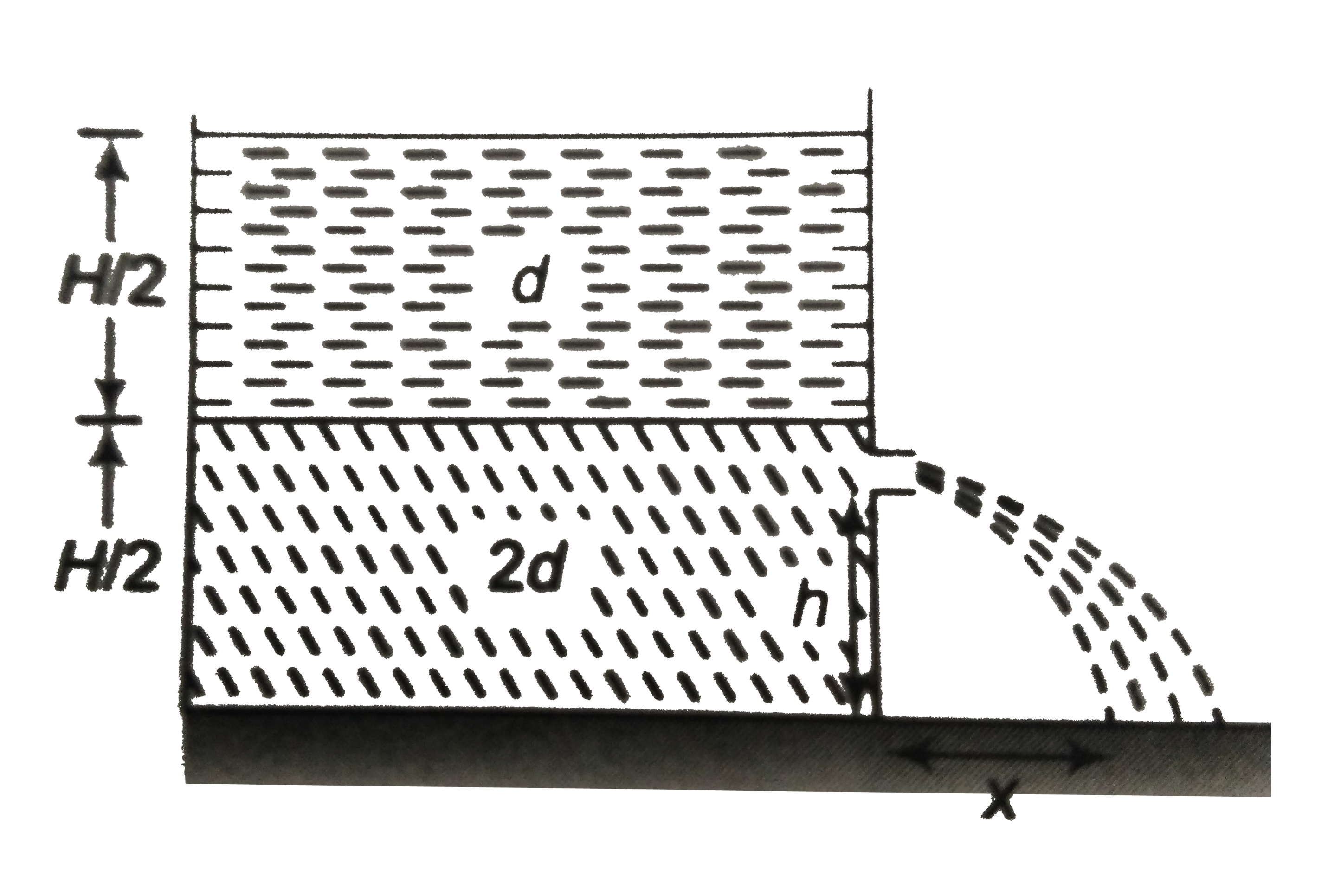
The cylinder is then removed and the original arrangement is restroed. a tiny hole of area s(s<<A) is punched on the vertical side of the container at a height h(h<H/2). As a result of this, liquid starts flowing out of the hole with a range x on the horizontal surface.
The initial value of x is
View SolutionFluids at rest exert a normal force to the walls of the container or to the sruface of the body immersed in the fluid. The pressure exerted by this force at a point inside the liqid is the sum of atmospheric pressure and a factor which depends on the density of the liquid, the acceleration due to gravity and the height of the liquid, above that point. The upthrust acting on a body immersed in a stationary liquid is the net force acting on the body in the upward direction. A number of phenomenon of liquids in motion can be explain by Bernoulli's theorem which relates the pressure, flow speed and height for flow of an ideal incompressible fluid.
A container of large uniform corss sectional area. A resting on a horizontal surface holds two immiscible, non viscous and incompressile liquids of densities d and 2d, each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0.
Situation II:
A cyliner is removed and the original arrangement is restoreed.A tiny hole of area s(s<<A) is punched on the veritical side of the containier at a height h(h<H/2)
The height hm at which the hole should be punched so that the liquid travels the maximum distance is
View Solution
