S is the centre of the circumcircle of ΔABC and if AD⊥BC, prove that ∠BAD=∠SAC. Solution in Bengali
S is the centre of the circum-circle of ΔABC.
∴SA=SB≠SC.
Also, AD⊥BC,∠ADB=90∘,∴∠ABD+∠BAD=90∘
or, ∠ABD−90∘−∠BAD........(1)
Now, in the circle with centre S, the central angle produced by the chord AD =∠ASC and angle in circle ∠ABC
∴ASC=2∠ABC.......(2)
But SA=SC[∴ radii of same circle]
∴∠SAC=∠SCA.......(3)
Then, ∠ASC+∠SAC+∠SCA=180∘
or, ∠ASC+∠SAC+∠SAC=180∘[from(3)]
or ∠ASC+2∠SAC=180∘
or, 2∠ABC+2∠SAC=180∘ [ by (2)]
or, ∠ABC+∠SAC=90∘ [ dividing by 2]
or, ∠ABD+∠SAC=90∘[∴∠ABCand∠ABD are same angle]
or,90∘−∠∠BAD+∠SAC=90∘ [ from (1)]
or, ∠SAC−∠BAD=0, or ∠SAC=∠BAD
Hence ∠ABD=∠SAC

Topper's Solved these Questions
- THEOREMS RELATED TO ANGLES IN A CIRCLEBook:CALCUTTA BOOK HOUSEChapter:THEOREMS RELATED TO ANGLES IN A CIRCLEExercise:EXAMPLES (True or False)
Explore 4 Videos
- THEOREMS RELATED TO ANGLES IN A CIRCLEBook:CALCUTTA BOOK HOUSEChapter:THEOREMS RELATED TO ANGLES IN A CIRCLEExercise:EXAMPLES (Short-Answer Type Questions) MCQs
Explore 5 Videos
- THEOREMS RELATED TO ANGLES IN A CIRCLEBook:CALCUTTA BOOK HOUSEChapter:THEOREMS RELATED TO ANGLES IN A CIRCLEExercise:EXAMPLES (Short Answer Type Questions)
Explore 5 Videos
- THEOREMS RELATED TO CYCLIC QUADRILATERAL Book:CALCUTTA BOOK HOUSEChapter:THEOREMS RELATED TO CYCLIC QUADRILATERAL Exercise:Long -answer type questions (L.A.)
Explore 12 Videos
- THEOREMS RELATED TO TANGENT IN A CIRCLE Book:CALCUTTA BOOK HOUSEChapter:THEOREMS RELATED TO TANGENT IN A CIRCLE Exercise:EXERCISE 4.2
Explore 23 Videos
Similar Questions
In the adjoining figure AD and BE are perpendicular ot BC and AC respectively of the ΔABC. The four points A,B,D,E are concylic.
View SolutionIn the adjoining figure, O is the centre of the circle AC is a diameter and chord DE is parallel to the diameter AC. If ∠CBD=60∘ , find the value of ∠CDE
View SolutionIn the adjacent figure O is the centre of the circle. If ∠OAB=30∘,∠ABC=120∘,∠BCO=y∘and∠COA=x∘, then the find value sof x and y.
View SolutionIn a cyclic quadrilateral, the ratio of three consecutive angle is 1 : 2 : 3 Find the value of first and third angles.
View SolutionThe distance of the point from the centre of a circle with diameter of 16 cm is 17 cm. Then the length of the tangent from the point to the circle is
View SolutionIn any triangle ABC, if the angle bisector of ∠Aand perpendicular bisector of BCintersect, prove that they intersect on the circumcircle of the triangle ABC
View SolutionA Δ ABC is right angled at A. L is a point on BC such that AL⊥ BC. Prove that ∠BAL=∠ACB.
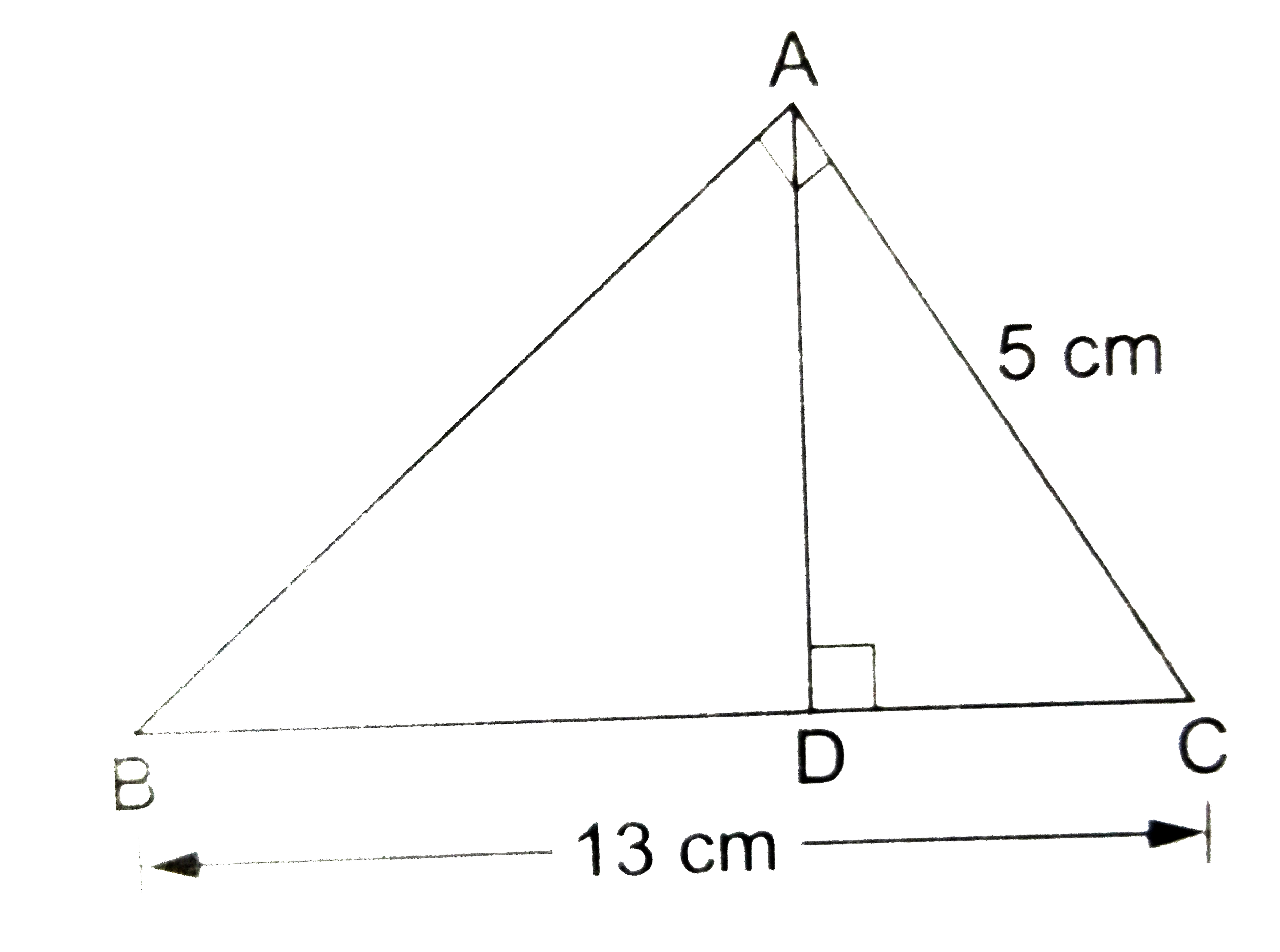
View SolutionΔABC is right-angled at A and AD⊥BC. If BC=13cmandAC=5cm, find the ratio of areas of ΔABCandΔADC.
View Solutionएक △ABC में, AD⊥BC तथा AD2=BD×CD है तो सिद्ध कीजिए कि △ABC का समकोण त्रिभुज है |
View Solution△ABC एक समकोण त्रिभुज है जिसका ∠Aसमकोण है तथा AD⊥BC है तो सिद्ध कीजिए कि BDAD=ABAC
View SolutionO is the orthocentre of ΔABCandAD⊥BC. If AD is produced it interect the circumcircle of ΔABC at the point G. Prove that OD=DG.
View SolutionO is the circumcentre of ΔABCandOD⊥BC. Prove that ∠BOD=∠BAC
View Solutionत्रिभुज ABC में, यदि AD⊥BC तथा AD2=BD×DC हो, तो सिद्ध करो की ∠BAC=90∘|
View Solutionचित्र में यदि AD⊥BC और BDDA=DADC हो, तो सिद्ध करो की ΔABC एक समकोण त्रिभुज है|
View Solutionयदि ABC का समकोण त्रिभुज है जिसका कोण A समकोण है तथा AD⊥BC है, तो BDDC=
View Solution