The isosceles triangle ABC is inscribed in the circle with centre at O.If AP be a diamtere passing through A, then show that AP is internal bisector of ∠BPC. Solution in Bengali
ΔABC is an isosceles triangle of which AB=AC. O is the centre of the in which ΔABC is insrbied and AP is dimeter of the circle.
To prove : AP is the internal bisector of ΔBPC,i.e.∠APB=∠APC.
Construction : Let us join the point O,B,C,C,B,PandC,P.
Proof In ΔABC,AB=AC,∴∠AOB=∠AOC[∴ equal segments of a circle prodcued equal front angles at the centre]
or , 2∠APB=2∠APC [ by thorem -34] or,∠APB=∠APC.
Hence AP is the internal bisector of ∠BPC.

Topper's Solved these Questions
- THEOREMS RELATED TO ANGLES IN A CIRCLEBOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO ANGLES IN A CIRCLEEXERCISE - EXAMPLES (True or False)
4 Videos
- THEOREMS RELATED TO ANGLES IN A CIRCLEBOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO ANGLES IN A CIRCLEEXERCISE - EXAMPLES (Short-Answer Type Questions) MCQs
5 Videos
- THEOREMS RELATED TO ANGLES IN A CIRCLEBOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO ANGLES IN A CIRCLEEXERCISE - EXAMPLES (Short Answer Type Questions)
5 Videos
- THEOREMS RELATED TO CYCLIC QUADRILATERAL BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO CYCLIC QUADRILATERAL EXERCISE - Long -answer type questions (L.A.)
12 Videos
- THEOREMS RELATED TO TANGENT IN A CIRCLE BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO TANGENT IN A CIRCLE EXERCISE - EXERCISE 4.2
23 Videos
Similar Questions
In the adjoining figure AD and BE are perpendicular ot BC and AC respectively of the ΔABC. The four points A,B,D,E are concylic.
View SolutionIn the adjoining figure, O is the centre of the circle AC is a diameter and chord DE is parallel to the diameter AC. If ∠CBD=60∘ , find the value of ∠CDE
View SolutionIn the adjacent figure O is the centre of the circle. If ∠OAB=30∘,∠ABC=120∘,∠BCO=y∘and∠COA=x∘, then the find value sof x and y.
View SolutionIn a cyclic quadrilateral, the ratio of three consecutive angle is 1 : 2 : 3 Find the value of first and third angles.
View SolutionThe distance of the point from the centre of a circle with diameter of 16 cm is 17 cm. Then the length of the tangent from the point to the circle is
View SolutionΔABC में, ∠A का समद्विभाजक AD भुजा BC पर लम्ब है। दर्शाइए कि AB = AC है और ΔABC समद्विबाहु है।
View SolutionABC एक समद्विबाहु त्रिभुज है जिसमें AB = AC और ∠B तथा ∠C के समद्विभाजक P पर प्रतिच्छेद करते हैं | साबित करें कि BP = CP और साथ ही AP,∠BAC का समद्विभाजक है |
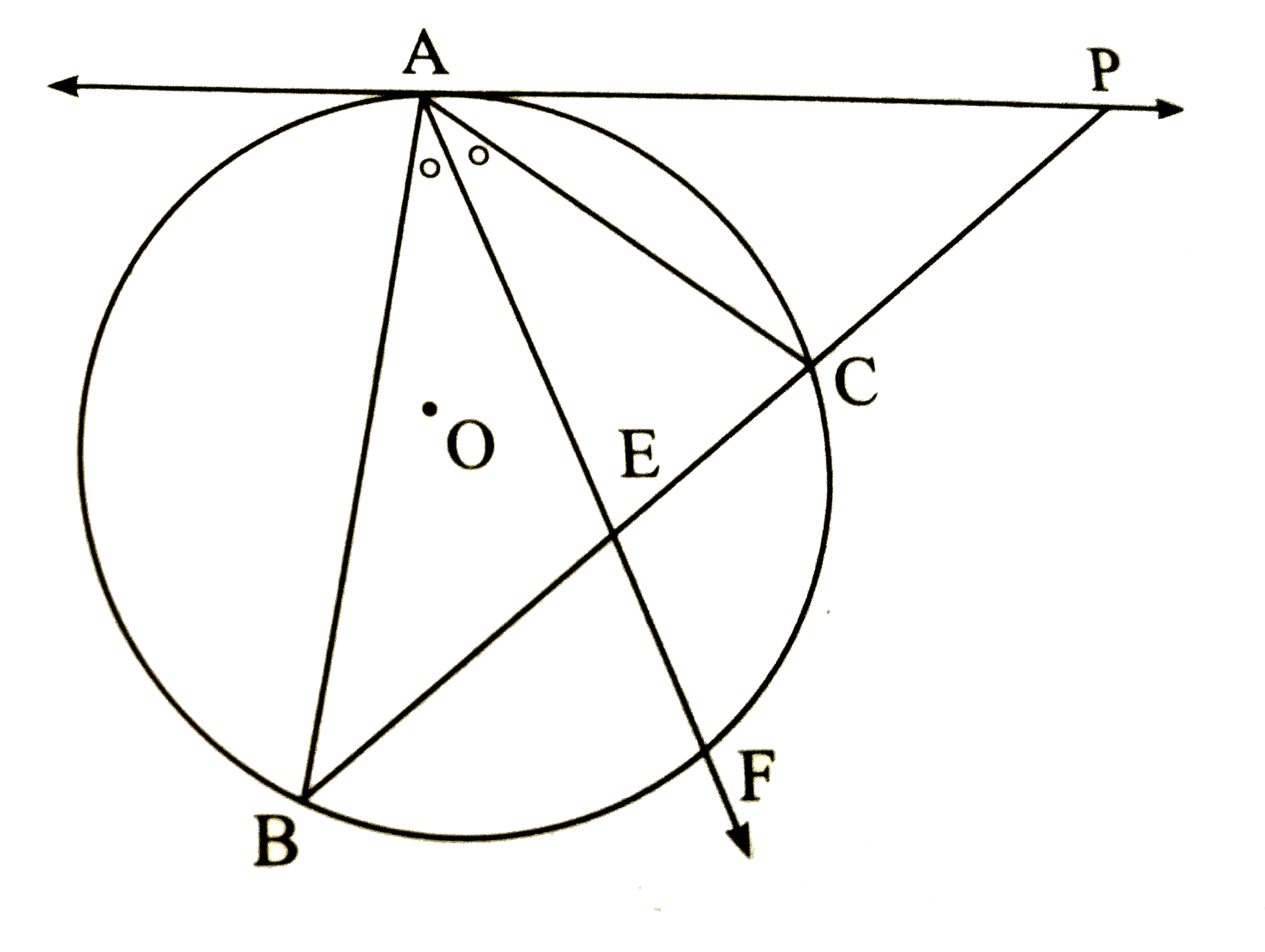
View SolutionΔABC is inscribed in a circle with centre O and line AP is a tangent at the point A. Ray AF is the bisector of ∠BAC
Prove that seg AP≅ seg PE.
View SolutionThe circle drawn with hypotenuse of a right angled triangle as diamter passes through the __________
View SolutionThe isosceles triangle ABC is inscribed in a circle with centre at O. If AP is a diameter of the circle. Then prove that AP is the bisetors of ∠BPC.
View Solutionत्रिभुज ABC में कोण B और C के समद्विभाजक बिंदु O पर काटते है|यदि बढ़ा हुआ AO भुजा BC को P पर काटता हो, तो सिद्ध करो की :
AP,∠BAC का समद्विभाजक है|
View SolutionABC is an isosceles triangle with AB = AC. Draw AP ⊥ BC to show that A = B.
View SolutionABC is an isosceles triangle with AB = AC. A circle through B touching AC at the middle point intersects AB at P. Then, AP : AB is
View Solutionদেখাও যে, একটি প্রদত্ত বৃত্তে অন্তর্লিখিত সমদ্বিবাহু ত্রিভুজসমূহের মধ্যে সমবাহু ত্রিভুজের পরিসীমা বৃহত্তম।
View SolutionABC is an isosceles triangle with AB = AC. A circle through B touching AC at the middle point intersects AB at P. Then AP : AB is :
View Solution
