Two containers of equal volume contain the same gas at pressure P1 and P2 and absolute temperature T1 and T2, respectively. On joining the vessels, the gas reaches a common pressure P and common temperature T. The ratio P/T is equal to
P1T1+P2T2
P1T1+P2T2(T1+T2)2
P1T2+P2T1(T1+T2)2
P12T1+P22T2
The correct Answer is:D
n1=P1VRT1 (for 1st vessel)
n2=P2VRT2 (for 2nd vessel)
If both vessels are joined together
n=n1+n2
⇒P(2V)RT=P1VRT1+P2VRT2
⇒PT=P12T1+P22T2
Similar Questions
An ideal gas in a thermally insulated vessel at internal pressure = P4, volume =V1 and absolute temperature =T1 expands irreversibly against zero external pressure, as shown in the diagram. The final internal pressure, volume and absolute temperature of the gas are P2,V2andT2, respectively. For this expansion.
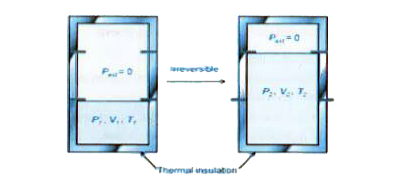
View SolutionTwo containers of equal volume contain the same gas at pressures P1andP2 and absolute temperatures T1andT2 respectively. On joining the vessels, the gas reaches a common pressure P and a common temperature T. Find the value P/T.
View SolutionTwo containers of equal volume contain the same gas at pressures P1andP2 and absolute temperatures T1andT2 respectively. On joining the vessels, the gas reaches a common pressure Panda common temperature T. Find the value P/T.
View SolutionTwo containers of equal volume contain the same gas at pressure P1 and P2 and absolute temperature T1 and T2, respectively. On joining the vessels, the gas reaches a common pressure P and common temperature T. The ratio P/T is equal to
View SolutionAn ideal gas in a thermally insulated vessel at internal pressure =P_(1) , volume =V_(1) and absolute temperature =T_(1) expands irreversibly against zero external pressure, as shown in the diagram. The final internal pressure, volume and absolute temperature of the gas are P_(2), V_(2) and T_(2) respectively. For this expansion.
View SolutionAn ideal gas in a thermally insulated vessel at internal pressure =P_(1) , volume =V_(1) and absolute temperature =T_(1) expands irreversibly against zero external pressure, as shown in the diagram. The final internal pressure, volume and absolute temperature of the gas are P_(2), V_(2) and T_(2) respectively. For this expansion.
View SolutionTwo containers of equal volume contain the same gas at pressure P_(1) and P_(2) and absolute temperature T_(1) and T_(2) , respectively. On joining the vessels, the gas reaches a common pressure P and common temperature T . The ratio P//T is equal to
View Solutionn_(1) moles of a monoatomic gas are contained in a vessel A of volume V_(1) at pressure P_(1) and temperature T_(1).n_(2) moles of the same gas are contained in a vessel B of volume V_(2) at pressure P_(2) and temperature T_(2) . The two vessels are now connected by a tube. Obtain the expression for common temperature T in the Vessels.
View Solution
