An ideal gas in a thermally insulated vessel at internal pressure $= P_{1}$ , volume $= V_{1}$ and absolute temperature $= T_{1}$ expands irreversibly against zero external pressure, as shown in the diagram. The final internal pressure, volume and absolute temperature of the gas are $P_{2}, V_{2}$ and $T_{2}$ respectively. For this expansion.

A

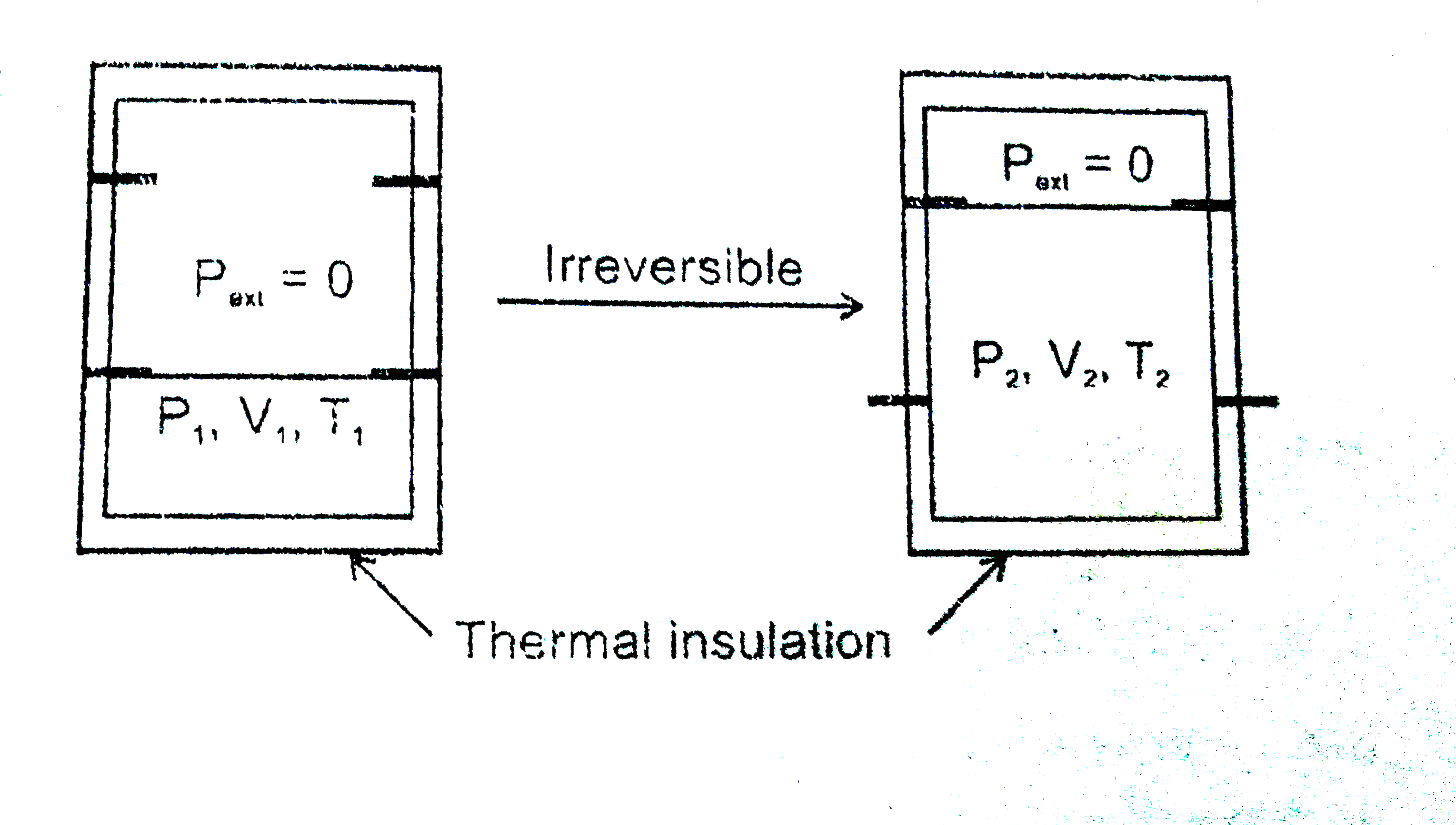
$q = 0$

B

$T_{2} = T_{1}$

C

$P_{2} V_{2} = P_{t} V_{1}$

D

$P_{2} V_{2}^{γ} = P_{1} V_{1}^{γ}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A, B, C

Since the vessel is thermally insulated so
$q = 0$
$p_{e x t} = 0$ , so $w = 0$
so $Δ U = 0$ (ideal gas)
Hence $Δ T = 0$
$\Rightarrow Δ T = 0 \Rightarrow T_{2} = T_{1} \Rightarrow P_{2} V_{2} = P_{1} V_{1}$
The process is however adiabatic irriversible.
So we cannot applly $P_{2} V_{2}^{γ} = P_{1} V_{1}$
Hence ans is $(A), (B), (C)$

Updated on:21/07/2023

|

Class 12 CHEMISTRY THERMODYNAMICS

Topper's Solved these Questions

Similar Questions

RESONANCE ENGLISH-THERMODYNAMICS-Exercise-3 Part-1: JEE (ADVANCED)

Home
Ask Doubt
Profile