A charged sphere of radius 0.02 m has charge density of 1 cm-2. The work done when a charge of 40 nano coulomb is moved from infinity to a point that is at a distance of 0.04 m from the centre of the sphere
1.44πJ
2J
14.4πJ
1.44J
The correct Answer is:C
To solve the problem, we need to calculate the work done when moving a charge from infinity to a point at a distance of 0.04 m from the center of a charged sphere. Here are the steps to find the solution:
Step 1: Identify the given values
- Radius of the sphere, R=0.02m
- Surface charge density, σ=1C/m2 (Note: 1 cm−2 = 10000 C/m2)
- Charge to be moved, q′=40nC=40×10−9C
- Distance from the center of the sphere, r=0.04m
Step 2: Calculate the total charge Q on the sphere
The total charge Q on the sphere can be calculated using the formula:
Q=σ⋅A
where A is the surface area of the sphere given by A=4πR2.
Calculating A:
A=4π(0.02)2=4π(0.0004)=0.005024m2
Now substituting A into the equation for Q:
Q=σ⋅A=1⋅0.005024=0.005024C
Step 3: Calculate the work done W
The work done in moving the charge q′ from infinity to a distance r from the center of the sphere is given by:
W=kQq′r
where k=9×109N m2/C2.
Substituting the values:
W=9×109⋅0.005024⋅40×10−90.04
Step 4: Simplify the equation
Calculating the numerator:
0.005024⋅40×10−9=0.00020096×10−9=2.0096×10−13
Now substituting this back into the equation for W:
W=9×109⋅2.0096×10−130.04
Calculating 2.0096×10−130.04:
2.0096×10−130.04=5.024×10−12
Now substituting this value back into the equation for W:
W=9×109⋅5.024×10−12=4.5216×10−2J
Step 5: Final Calculation
To express the work done in terms of π:
W≈14.4π×10−2J
Conclusion
The work done when a charge of 40 nano coulombs is moved from infinity to a point that is at a distance of 0.04 m from the center of the sphere is approximately:
W≈14.4πJ
- Radius of the sphere, R=0.02m
- Surface charge density, σ=1C/m2 (Note: 1 cm−2 = 10000 C/m2)
- Charge to be moved, q′=40nC=40×10−9C
- Distance from the center of the sphere, r=0.04m
The total charge Q on the sphere can be calculated using the formula:
Q=σ⋅A
where A is the surface area of the sphere given by A=4πR2.
A=4π(0.02)2=4π(0.0004)=0.005024m2
Q=σ⋅A=1⋅0.005024=0.005024C
The work done in moving the charge q′ from infinity to a distance r from the center of the sphere is given by:
W=kQq′r
where k=9×109N m2/C2.
W=9×109⋅0.005024⋅40×10−90.04
Calculating the numerator:
0.005024⋅40×10−9=0.00020096×10−9=2.0096×10−13
W=9×109⋅2.0096×10−130.04
Calculating 2.0096×10−130.04:
2.0096×10−130.04=5.024×10−12
W=9×109⋅5.024×10−12=4.5216×10−2J
To express the work done in terms of π:
W≈14.4π×10−2J
The work done when a charge of 40 nano coulombs is moved from infinity to a point that is at a distance of 0.04 m from the center of the sphere is approximately:
W≈14.4πJ
Topper's Solved these Questions
- APPENDICES ( REVISION EXERCISE )Book:AAKASH SERIESChapter:APPENDICES ( REVISION EXERCISE )Exercise:REVISION EXERCISE (CUTTENT ELECTRICITY )
Explore 108 Videos
- APPENDICES ( REVISION EXERCISE )Book:AAKASH SERIESChapter:APPENDICES ( REVISION EXERCISE )Exercise:REVISION EXERCISE (MOVING CHANGES & MEGNETISM)
Explore 95 Videos
- APPENDICES ( REVISION EXERCISE )Book:AAKASH SERIESChapter:APPENDICES ( REVISION EXERCISE )Exercise:REVISION EXERCISE (MAGNETISM AND MATTER )
Explore 52 Videos
- ALTERNATING CURRENTBook:AAKASH SERIESChapter:ALTERNATING CURRENTExercise:EXERCISE - III
Explore 24 Videos
- APPENDICES (REVISION EXERCISE)Book:AAKASH SERIESChapter:APPENDICES (REVISION EXERCISE)Exercise:LAW OF MOTION
Explore 128 Videos
Similar Questions
A solid sphere of radius R1 and volume charge density ρ=ρ0r is enclosed by a hollow sphere of radius R2 with negative surface charge density σ, such that the total charge in the system is zero . ρ0 is positive constant and r is the distance from the centre of the sphere . The ratio R2/R1 is
View SolutionA charged disc of radius 3 m has charge density σ. Electric field at a distance of 4 m from its centre on axis is _______
View Solution
Knowledge Check
A system consits of a uniformly charged sphere of radius R and a surrounding medium filled by a charge with the volume density ρ=αr, where α is a positive constant and r is the distance from the centre of the sphere. Find the charge of the sphere for which the electric field intensity E outside the sphere is independent of R.
Aα2ε0B2αε0C2παR2DNone of these
A hollow spherical conductor of radius 1m has a charge of 250μC then electric intensity at a point distance of 0.5m from the centre of the spherical conductor is
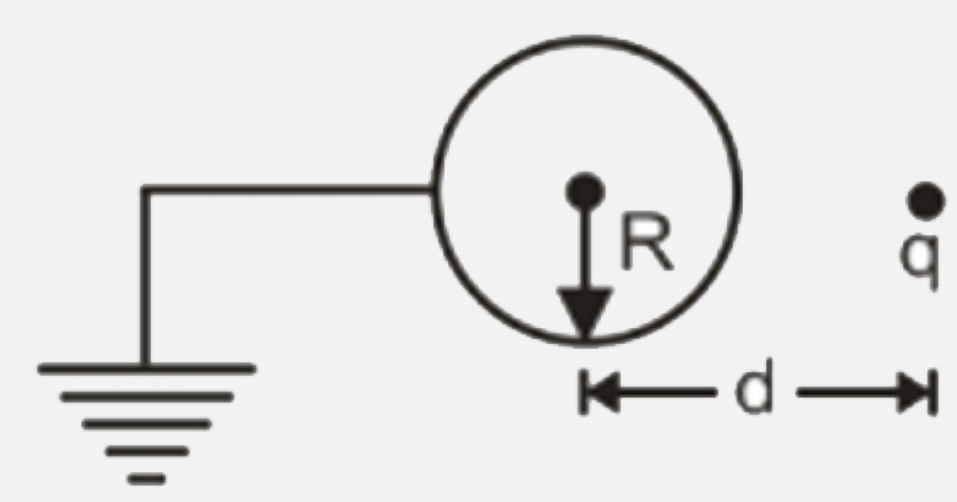
View SolutionIn the shown figure, the charge appearing on the conducting shell (grounded) of Radius R, when a point charge q is kept at a distance of d from the centre of the sphere is:
View SolutionThe diameter of a hollow metallic sphere is 60 cm and the sphere carries a charge of 500μC. The potential at a distance of 100cm from the centre of the sphere will be
View SolutionA hollow metallic sphere of radius 0.1 m is charged to a potential of 5 V. The potential at its centre is
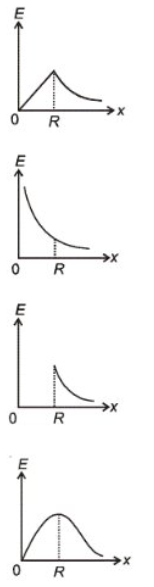
View SolutionA non-conducting solid sphere of radius R, has a uniform charge density. The graph representing variation of magnitude of electric field (E) as a function of distance (x) from the centre of the sphere is
View SolutionA conducting sphere of radius R is charged to a potential of V volts. Then the electric field at a distance r(>R) from the centre of the sphere would be
View SolutionA solid non conducting sphere of radius R has a non-uniform charge distribution of volume charge density, ρ=ρ0rR, where ρ0 is a constant and r is the distance from the centre of the sphere. Show that : (i) the total charge on the sphere is Q=πρ0R3 (ii) the electric field inside the sphere has a magnitude given by, E=KQr2R4
View Solution

