v1andv2 are the velocities of sound at the same temperature in two monoatomic gases of densities ρ1andρ2 respectively. If ρ1ρ2=14 then the ratio of velocities v1andv2 is
1:2
4:1
2:1
1:4
The correct Answer is:C
To solve the problem, we need to find the ratio of the velocities of sound in two monoatomic gases given their densities. Let's break it down step by step:
Step 1: Understand the relationship between velocity of sound and density
The velocity of sound v in a medium is given by the formula:
v=√P⋅γρ
where:
- P is the pressure,
- γ is the adiabatic index (ratio of specific heats, Cp/Cv),
- ρ is the density of the gas.
Step 2: Write the expressions for the velocities of the two gases
For the first gas:
v1=√P⋅γρ1
For the second gas:
v2=√P⋅γρ2
Step 3: Find the ratio of the velocities
To find the ratio v1v2, we divide the two expressions:
v1v2=√P⋅γρ1√P⋅γρ2
This simplifies to:
v1v2=√ρ2ρ1
Step 4: Substitute the given density ratio
We are given that:
ρ1ρ2=14
From this, we can find ρ2ρ1:
ρ2ρ1=4
Step 5: Calculate the ratio of velocities
Now substituting this back into our equation for the ratio of velocities:
v1v2=√4=2
Step 6: Final ratio
Thus, the ratio of the velocities v1:v2 is:
v1:v2=2:1
Conclusion
The final answer is that the ratio of the velocities v1 and v2 is 2:1.
---
The velocity of sound v in a medium is given by the formula:
v=√P⋅γρ
where:
- P is the pressure,
- γ is the adiabatic index (ratio of specific heats, Cp/Cv),
- ρ is the density of the gas.
For the first gas:
v1=√P⋅γρ1
For the second gas:
v2=√P⋅γρ2
To find the ratio v1v2, we divide the two expressions:
v1v2=√P⋅γρ1√P⋅γρ2
This simplifies to:
v1v2=√ρ2ρ1
We are given that:
ρ1ρ2=14
From this, we can find ρ2ρ1:
ρ2ρ1=4
Now substituting this back into our equation for the ratio of velocities:
v1v2=√4=2
Thus, the ratio of the velocities v1:v2 is:
v1:v2=2:1
The final answer is that the ratio of the velocities v1 and v2 is 2:1.
Topper's Solved these Questions
- WAVESBOOK - AAKASH SERIESCHAPTER - WAVESEXERCISE - EXERCISE-II (Pipes)
13 Videos
- WAVESBOOK - AAKASH SERIESCHAPTER - WAVESEXERCISE - EXERCISE-II (Beats : )
20 Videos
- WAVESBOOK - AAKASH SERIESCHAPTER - WAVESEXERCISE - EXERCISE-II (Strings (Speed of a travelling wave:))
23 Videos
- WAVE OPTICSBOOK - AAKASH SERIESCHAPTER - WAVE OPTICSEXERCISE - PROBLEMS (LEVEL - II)
33 Videos
- WAVES OPTICSBOOK - AAKASH SERIESCHAPTER - WAVES OPTICSEXERCISE - EXERCISE -III (POLARISITION)
10 Videos
Similar Questions
The velocities of sound at same temperature in two monoatomic gases densities ρ1 and ρ2 are v1 and v2 repectively , if ρ1ρ2=4, then the value of v1v2 will be
View SolutionA solid sphere having volume V and density ρ floats at the interface of two immiscible liquids of densites ρ1 and ρ2 respectively. If ρ1<ρ<ρ2, then the ratio of volume of the parts of the sphere in upper and lower liquid is
View SolutionIf two liquids of same mass but densities ρ1 and ρ2 respectively are mixed, then the density of the mixture is:
View SolutionIf at same temperature and pressure, the densities for two diatomic gases are respectively d1andd2 , then the ratio of velocities of sound in these gases will be
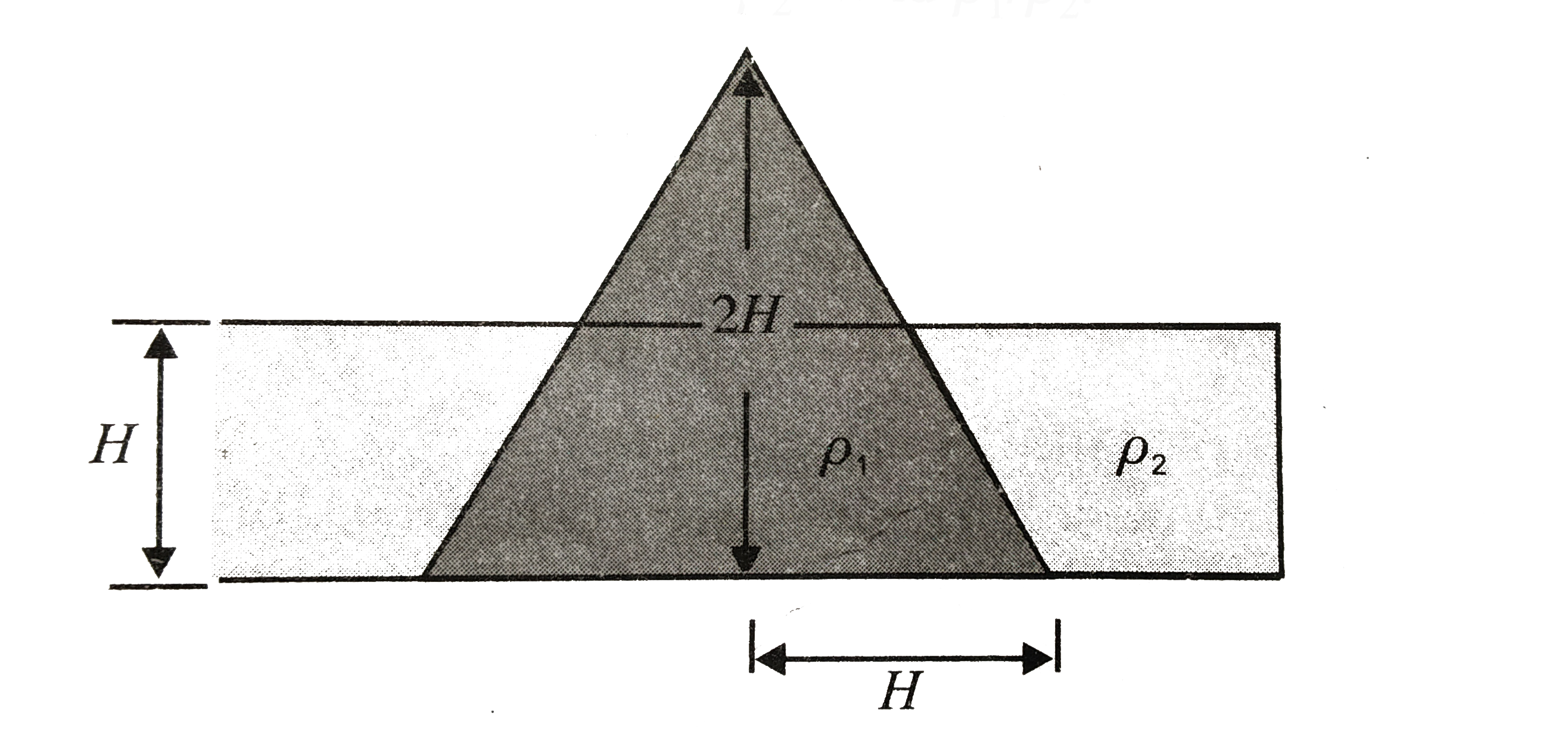
View SolutionThe light cone is in equilibrium under the action of hydrostatic forces of two liquids of densities ρ1 and ρ2. Find ρ1/ρ2. Find ρ1 and ρ2.
View SolutionThe velocities of sound in an ideal gas at temperature T1andT2 K are found to be V1andV2 respectively. If ther.m.s velocities of the molecules of the same gas at the same temperatures T1andT2 are v1andv2 respectively then
View SolutionThe velocities of sound in an ideal gas at temperature T1andT2 K are found to be V1andV2 respectively. If ther.m.s velocities of the molecules of the same gas at the same temperatures T1andT2 are v1andv2 respectively then
View SolutionEqual masses of two substance of densities ρ1andρ2 are mixed together. What is the density of the mixture?
View SolutionWhat is the significance of resistance ratio, ρ300K/ρ4.2K ?
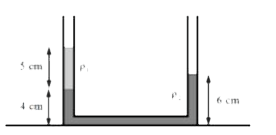
View SolutionA narrow U-tube placed on a horizontal surface contains two liquids of densities ρ1 and ρ2 . The columns of the liquids are indicated in the figure. The ratio ρ1ρ2 is equal to :
View Solution
