PR is tangent to a circle, with centre O and radius 4 cm, at point Q. If ∠POR=90∘,OR=5cmand OP=203cm, then (in cm) the length of PR :
3
163
233
253
The correct Answer is:D
To solve the problem, we will follow these steps:
1. Understand the Geometry: We have a circle with center O and radius 4 cm. The line PR is tangent to the circle at point Q. We know that the radius at the point of tangency is perpendicular to the tangent line. Therefore, angle OQR = 90°.
2. Identify Given Values:
- Radius (OQ) = 4 cm
- Length OR = 5 cm
- Length OP = 20/3 cm
3. Use Pythagorean Theorem: In triangle OPR, we can apply the Pythagorean theorem since it is a right triangle (angle OQR = 90°). The sides are:
- OR (one leg) = 5 cm
- OP (hypotenuse) = 20/3 cm
- PR (the other leg, which we need to find)
According to the Pythagorean theorem:
OP2=OR2+PR2
4. Substitute the Values:
(203)2=52+PR2
4009=25+PR2
5. Convert 25 to a Fraction:
25=2259
So, we rewrite the equation:
4009=2259+PR2
6. Isolate PR^2:
PR2=4009−2259
PR2=1759
7. Take the Square Root:
PR=√1759=√1753
Simplifying √175:
√175=√25×7=5√7
Therefore:
PR=5√73
8. Final Answer:
The length of PR is 5√73 cm.
- Radius (OQ) = 4 cm
- Length OR = 5 cm
- Length OP = 20/3 cm
- OR (one leg) = 5 cm
- OP (hypotenuse) = 20/3 cm
- PR (the other leg, which we need to find)
OP2=OR2+PR2
(203)2=52+PR2
4009=25+PR2
25=2259
So, we rewrite the equation:
4009=2259+PR2
PR2=4009−2259
PR2=1759
PR=√1759=√1753
Simplifying √175:
√175=√25×7=5√7
Therefore:
PR=5√73
The length of PR is 5√73 cm.
Topper's Solved these Questions
- GEOMETRYBook:KIRAN PUBLICATIONChapter:GEOMETRYExercise:QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE- XIV)
Explore 90 Videos
- GEOMETRYBook:KIRAN PUBLICATIONChapter:GEOMETRYExercise:TRY YOURSELF
Explore 25 Videos
- GEOMETRYBook:KIRAN PUBLICATIONChapter:GEOMETRYExercise:QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE- XII)
Explore 90 Videos
- DISCOUNTBook:KIRAN PUBLICATIONChapter:DISCOUNTExercise:Test Yourself
Explore 10 Videos
- LCM AND HCF Book:KIRAN PUBLICATIONChapter:LCM AND HCF Exercise:Test Yourself
Explore 18 Videos
Similar Questions
Knowledge Check
PR is tangent to a circle, with centre O and radius 4 cm, at point Q. If ∠POR=90∘,OR=5cmand OP=203cm, then (in cm) the length of PR :
A3B163C233D253PR is a tangent at a point Q on a circle, in which Q is the centre and its radius is 8 cm. If OR = 16 cm and OP = 10 cm, then the length of PR is-
A18 cmB19 cmC19.8 cmD21.86 cmPT is a tangent to a circle with centre O and radius 6cm. If PT is 8cm then length of OP is:
A10 cm.B12 cm.C16 cm.D9 cm.
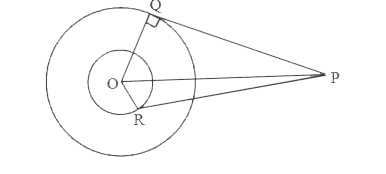
In the given figure, PQ is tangent to outer circle and PR is tangent to inner circle. If PQ = 4cm, OQ = 3 cm and OR = 2 cm then find the length of PR.
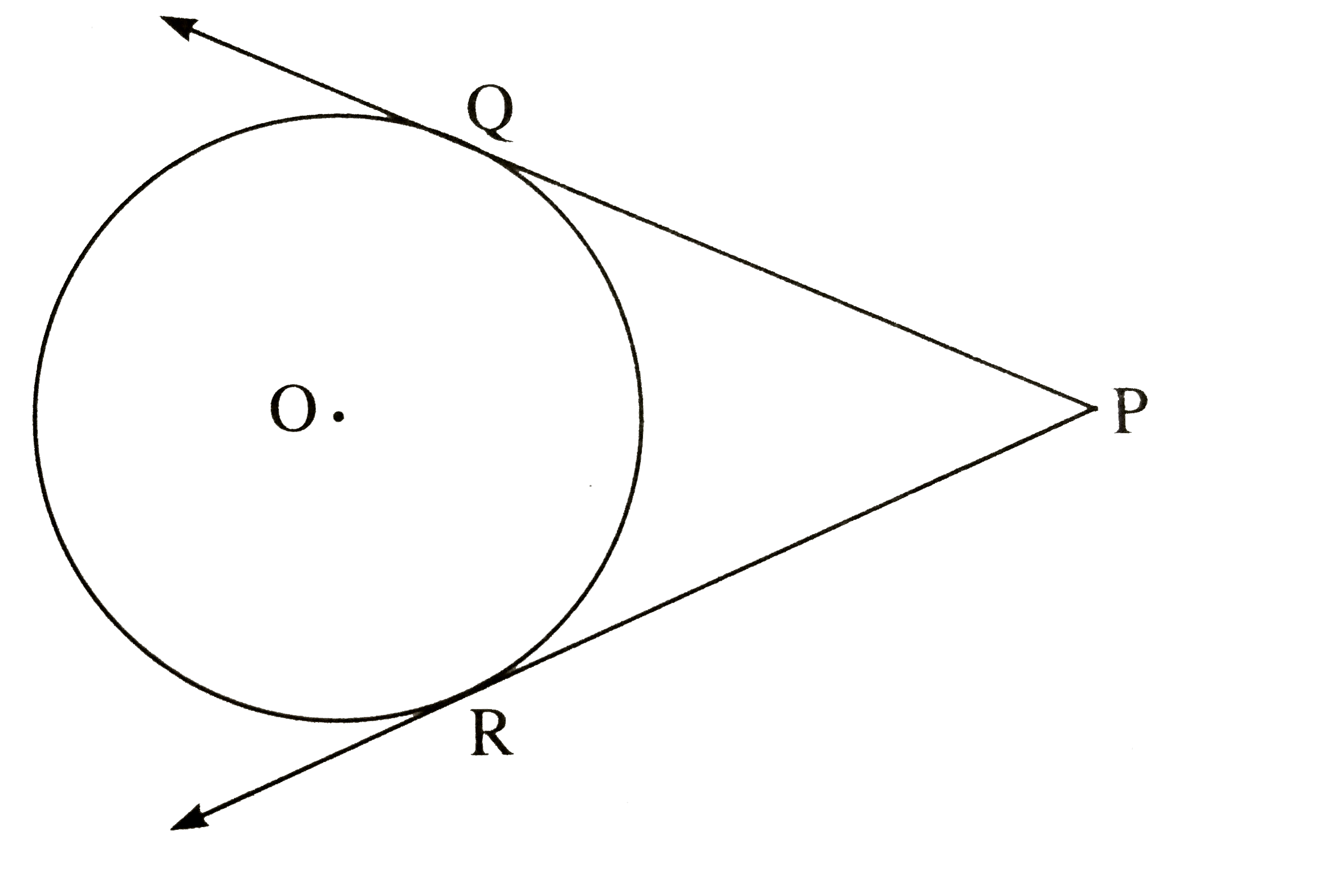
View SolutionIn the figure , PQ and PR are tangents drawn from and external point P to the circle with centre O. Q and R are the points of contact. If PQ = 5 cm then what is the length of segment PR ? Why ?
View SolutionThe circle of radius 4 cm and centre O has a tangent PR. If ∠POR=90∘ and OR = 7 cm and OP=24cm. Calculate the length (in cm.) of PR.
View SolutionAB is a tangent to a circle with centre O. If the radius at the circle is 7 cm and the length of AB is 24 cm, then what is the length (in cm) of OA?
View SolutionAP is tangent to the circle with centre O at point A. OP =10 cm and ∠OPA=30∘. The radiu of the circle is
View Solution