It is desired to make a 20Ω coil of wire, which has a zero thermal coefficient of resistance. To do this, a carbon resistor of resistance R1 is placed in series with an iron resistor of resistance R2. The proportions of iron and carbon are so chosen that R1+R2=20Ω for all temperatures near 20∘C. How long are R1andR2? αcarbon=−0.5×10−3.∘C−1,αiron=5×10−3.∘C(−1)
We need R1(1+α1△t)+R2(1+α2△t)=20,because R1+R2=20where△t=0
We must haveR1∝1R2∝2whit∝1=−0.5×10−3and∝2=5×10−3
Solving the two equations, R1+R2=20 and R1=18.18ΩandR2=1.82Ω
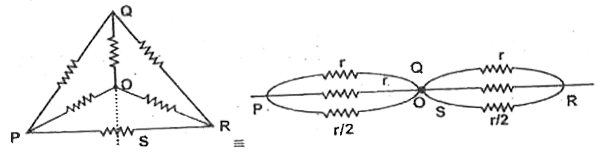
(b) There is a symmetry about the line passing through QO and mid point of PR
RPR=r2=2Ω
( c) There is a symmetry about the line passing through E and mid point of CD.
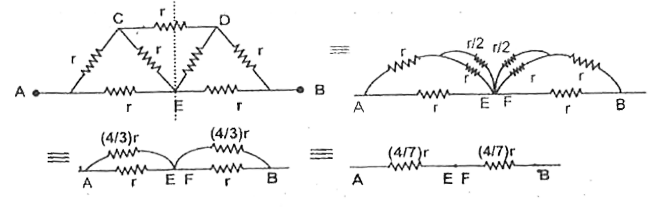
RAB=87r=87Ω
(d) The resistors 3Ω and 6Ω are in series and so are 5Ω and 10Ω resistors. These two series equivalents are in parallel to each other and also to the 4 Hence, the network reduces to the one given in the above figureReq=5.34Ω
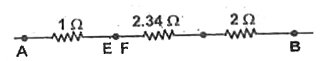
Similar Questions
It is desired to make a 20Ω coil of wire, which has a zero thermal coefficient of resistance. To do this, a carbon resitor fo resistane R1 is placed in series with an iron resistor of resistance R2 the proportions of iron and carbon are so chosen that R1+R2=20Ω for all temperatures near 20∘C. How long are R1andR2? αcarbon=−0.5×10−3.∘C−1,αiron=5×10−3.∘C(−1)
View SolutionA resistor of resistance R is connected to a cell internal resistance 5Ω. The value of R is varied from 1Ω to 5Ω. The power consumed by R
View Solution
Knowledge Check
It is desired to make a long cylindrical conductor whose temperature coefficient of resistivity at 20∘C will be close to zero. If such a conductor is made by assembling alternate disks of iron and carbon, find the ratio of the thickness of a carbon disk to that an iron disk. (For carbon,p=3500×10−8Ωmandα=−0.50×10−3.∘C−1for iron, p=9.68×10−8Ωmandα=6.5×10−3.∘C−1)
A0.36B0.036C1D2
The temperature coefficient of resistance of conductor varies as α(T)=3T2+2T. If R0 is resistance at T = 0and R is resistance at T, then
View SolutionA galvanometer has range 0 − V volt and it has resistance R. When the series resistor of resistance 2R is connected, the range becomes 0 ⋅ V . Then
View SolutionConsider the circuit shown in fig.
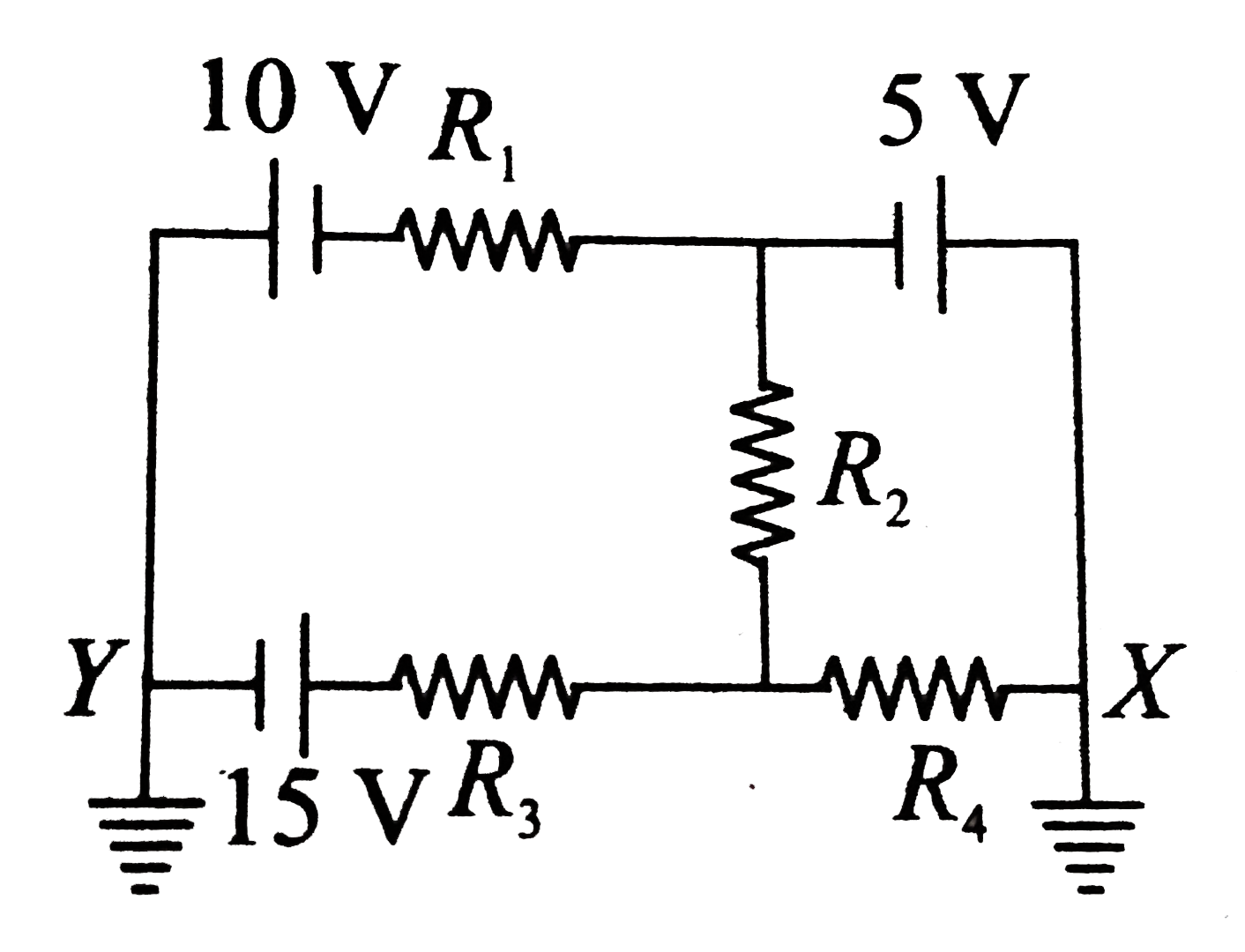
Assuming R1=2Ω, current passing through resistance R1 is
View SolutionTwo resistors of resistance R1 and R2 having R1>R2 are connected in parallel. For equivalent resistance R, the correct statement is
View SolutionTwo resistors of resistance R1 and R2 having R1>R2 are connected in parallel. For equivalent resistance R, the correct statement is
View SolutionShow by a diagram how two resistors R1andR2 are joined in parallel. Obtain an expression for the total resistance of the combination.
View SolutionA resistance R of therimal coefficient of resistivity α is connected in parallel with a resistance 3R. Having thermal coeffiecinet of resistivity 2α Find the value of αeff
View Solution
