It is desired to make a 20Ω coil of wire, which has a zero thermal coefficient of resistance. To do this, a carbon resitor fo resistane R1 is placed in series with an iron resistor of resistance R2 the proportions of iron and carbon are so chosen that R1+R2=20Ω for all temperatures near 20∘C. How long are R1andR2? αcarbon=−0.5×10−3.∘C−1,αiron=5×10−3.∘C(−1)
To solve the problem of finding the resistances R1 and R2 of the carbon and iron resistors that together make a total resistance of 20Ω with a zero thermal coefficient of resistance, we can follow these steps:
Step 1: Understand the given values
We know:
- The total resistance R1+R2=20Ω
- The temperature coefficients of resistance:
- αcarbon=−0.5×10−3∘C−1
- αiron=5×10−3∘C−1
Step 2: Write the resistance formulas
The resistance of a material at a temperature T can be expressed as:
R=R0(1+αΔT)
where R0 is the resistance at a reference temperature (let's assume 20∘C), α is the temperature coefficient, and ΔT is the change in temperature.
Step 3: Set up the equations for the resistances at a temperature T
For carbon:
R′1=R1(1+αcarbonΔT)
For iron:
R′2=R2(1+αironΔT)
Step 4: Set up the equation for total resistance at temperature T
At temperature T, the total resistance must still equal 20Ω:
R′1+R′2=20
Substituting the expressions for R′1 and R′2:
R1(1+αcarbonΔT)+R2(1+αironΔT)=20
Step 5: Simplify the equation
Expanding this gives:
R1+R2+R1αcarbonΔT+R2αironΔT=20
Since R1+R2=20:
20+R1αcarbonΔT+R2αironΔT=20
This simplifies to:
R1αcarbonΔT+R2αironΔT=0
Factoring out ΔT:
ΔT(R1αcarbon+R2αiron)=0
Step 6: Set the coefficient to zero
Since ΔT cannot be zero, we have:
R1αcarbon+R2αiron=0
Step 7: Solve for the ratio of resistances
Rearranging gives:
R1αcarbon=−R2αiron
Thus, we can express the ratio:
R1R2=−αironαcarbon
Substituting the values:
R1R2=−5×10−3−0.5×10−3=10
This means:
R1=10R2
Step 8: Substitute into the total resistance equation
Substituting R1=10R2 into R1+R2=20:
10R2+R2=20
11R2=20
R2=2011≈1.818Ω
Then substituting back to find R1:
R1=10R2=10×2011=20011≈18.182Ω
Final Results
Thus, the resistances are:
R1≈18.182Ω,R2≈1.818Ω
We know:
- The total resistance R1+R2=20Ω
- The temperature coefficients of resistance:
- αcarbon=−0.5×10−3∘C−1
- αiron=5×10−3∘C−1
The resistance of a material at a temperature T can be expressed as:
R=R0(1+αΔT)
where R0 is the resistance at a reference temperature (let's assume 20∘C), α is the temperature coefficient, and ΔT is the change in temperature.
For carbon:
R′1=R1(1+αcarbonΔT)
For iron:
R′2=R2(1+αironΔT)
At temperature T, the total resistance must still equal 20Ω:
R′1+R′2=20
Substituting the expressions for R′1 and R′2:
R1(1+αcarbonΔT)+R2(1+αironΔT)=20
Expanding this gives:
R1+R2+R1αcarbonΔT+R2αironΔT=20
Since R1+R2=20:
20+R1αcarbonΔT+R2αironΔT=20
This simplifies to:
R1αcarbonΔT+R2αironΔT=0
Factoring out ΔT:
ΔT(R1αcarbon+R2αiron)=0
Since ΔT cannot be zero, we have:
R1αcarbon+R2αiron=0
Rearranging gives:
R1αcarbon=−R2αiron
Thus, we can express the ratio:
R1R2=−αironαcarbon
Substituting the values:
R1R2=−5×10−3−0.5×10−3=10
This means:
R1=10R2
Substituting R1=10R2 into R1+R2=20:
10R2+R2=20
11R2=20
R2=2011≈1.818Ω
Then substituting back to find R1:
R1=10R2=10×2011=20011≈18.182Ω
Thus, the resistances are:
R1≈18.182Ω,R2≈1.818Ω
Topper's Solved these Questions
- ELECTRIC CURRENT AND CIRCUITBook:CENGAGE PHYSICS ENGLISHChapter:ELECTRIC CURRENT AND CIRCUITExercise:Exercise 5.2
Explore 50 Videos
- ELECTRIC CURRENT AND CIRCUITBook:CENGAGE PHYSICS ENGLISHChapter:ELECTRIC CURRENT AND CIRCUITExercise:Subjective
Explore 29 Videos
- ELECTRIC CURRENT AND CIRCUITBook:CENGAGE PHYSICS ENGLISHChapter:ELECTRIC CURRENT AND CIRCUITExercise:Solved Examples
Explore 12 Videos
- ELECTRIC CURRENT & CIRCUITSBook:CENGAGE PHYSICS ENGLISHChapter:ELECTRIC CURRENT & CIRCUITSExercise:Kirchhoff s law and simple circuits
Explore 15 Videos
- ELECTRIC FLUX AND GAUSS LAWBook:CENGAGE PHYSICS ENGLISHChapter:ELECTRIC FLUX AND GAUSS LAWExercise:MCQ s
Explore 38 Videos
Similar Questions
The temperature coefficient of resistance of conductor varies as α(T)=3T2+2T. If R0 is resistance at T = 0and R is resistance at T, then
View SolutionA resistance R of therimal coefficient of resistivity α is connected in parallel with a resistance 3R. Having thermal coeffiecinet of resistivity 2α Find the value of αeff
View Solution
Knowledge Check
It is desired to make a long cylindrical conductor whose temperature coefficient of resistivity at 20∘C will be close to zero. If such a conductor is made by assembling alternate disks of iron and carbon, find the ratio of the thickness of a carbon disk to that an iron disk. (For carbon,p=3500×10−8Ωmandα=−0.50×10−3.∘C−1for iron, p=9.68×10−8Ωmandα=6.5×10−3.∘C−1)
A0.36B0.036C1D2
Consider the circuit shown in fig.
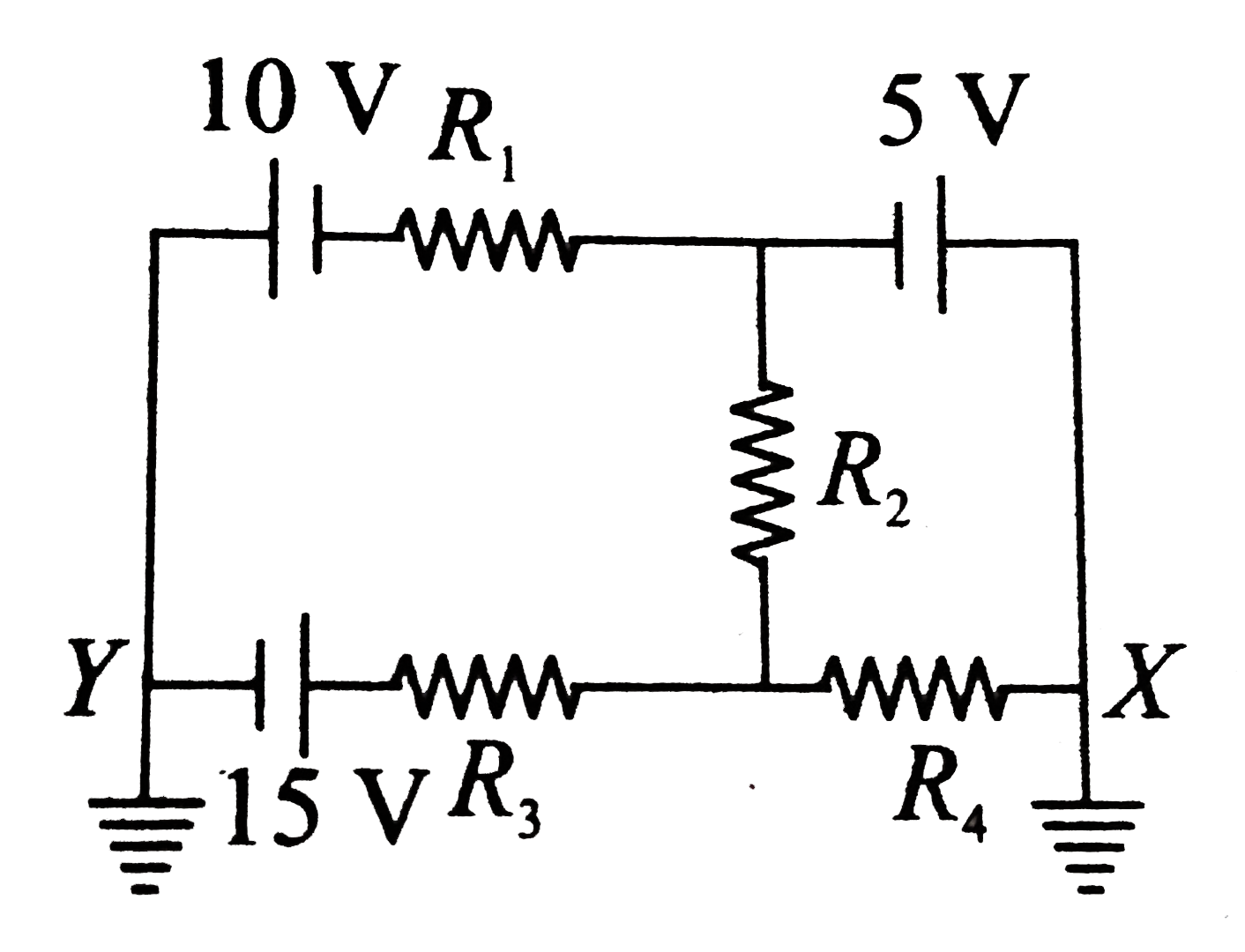
Assuming R1=2Ω, current passing through resistance R1 is
View SolutionShow by a diagram how two resistors R1andR2 are joined in parallel. Obtain an expression for the total resistance of the combination.
View SolutionTwo wires of resistance R1 and R2 have temperature coefficient of resistance α1 and α2 respectively. These are joined in series. The effective temperature coefficient of resistance is
View SolutionTwo wires of resistance R1 and R2 have temperature coefficient of resistance α1 and α2 respectively. These are joined in series. The effective temperature coefficient of resistance is
View SolutionA carbon and an aluminium wire are connected in series. If the combination has resistance of 30Ω at 30∘C, what is the resistance of each wire at 0∘Cso that resistance of the combination does not change with temperature.(ac=−0.5×10−3/∘C,aA=4×10−3/∘C)
View SolutionA resistor of resistance R is connected to a cell internal resistance 5Ω. The value of R is varied from 1Ω to 5Ω. The power consumed by R
View SolutionTwo resistors of resistance R1 and R2 having R1>R2 are connected in parallel. For equivalent resistance R, the correct statement is
View Solution