It is desired to make a 20.0Ω coil of wire whose temperature coefficient of resistance is zero. To do this, a carbon resistor of resistance R1 is placed in series with an iron resistor of resistance R2. The proportion of iron and carbon are so chosen that R1+R2=20Ω for all temperatures near 20∘C. Find the values of R1 and R2. Temperature coefficient of resistance for carbon, αC=−0.5×10−3/∘C and that of iron is αFe=5×10−3/∘C.
Let Δt∘C be the rise in temperature.
As per question we need,
R1(1+αCΔt)+R2(1+αFeΔt)=20
Since, R1+R2=20, therefore,
R1αCΔt+R2αFeΔt=0
or R1+αC=−R21+αFe
or R1×(+0.5×10−3)=−R2×5×10−3
or R1=10R2
So, 10R2+R2=20or11R2=20
or R2=20/11=1.82Ω
and R1=10×1.82=18.2Ω
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - PRADEEPCHAPTER - CURRENT ELECTRICITYEXERCISE - Conceptual Problems
3 Videos
- CURRENT ELECTRICITYBOOK - PRADEEPCHAPTER - CURRENT ELECTRICITYEXERCISE - Very short Q/A
7 Videos
- COMMUNICATION SYSTEMSBOOK - PRADEEPCHAPTER - COMMUNICATION SYSTEMSEXERCISE - MODEL TEST PAPER-2
9 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - PRADEEPCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - Exercise
191 Videos
Similar Questions
It is desired to make a 20Ω coil of wire, which has a zero thermal coefficient of resistance. To do this, a carbon resitor fo resistane R1 is placed in series with an iron resistor of resistance R2 the proportions of iron and carbon are so chosen that R1+R2=20Ω for all temperatures near 20∘C. How long are R1andR2? αcarbon=−0.5×10−3.∘C−1,αiron=5×10−3.∘C(−1)
View SolutionIt is desired to make a 20Omega coil of wire which has a zero thermal coefficient of resistance. To do this, a carbon resistor of resistance R, is placed in series with an iron resistor of resistance R. The proportions of Iron and carbon are so chosen that R1,+R2=20Ω for all temperatures near20∘C. How large are Ri andR2 ? (a−(c)=−0.5×10−3/∘C,afe=5×10−3/∘C)
(b) Six equal resistances, each of resistance4Ω are connected to form the following figure. What is the resistance between any two corners?
(c) In the network shown in figuro, each resistance is 1Ω. What is the effective resistance between A and B.
(d) Find the equivalent resistance between points A and B of the network shown in the given diagram.
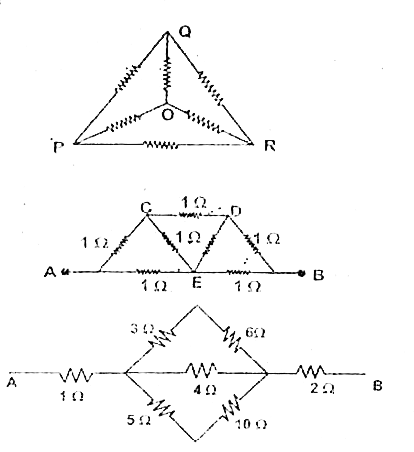
View Solution
Knowledge Check
The temperature coefficient of resistance of conductor varies as α(T)=3T2+2T. If R0 is resistance at T = 0and R is resistance at T, then
AR=R0(6T−2)BR=2R0(3+2T0CR=R0(1+T2+T2)DR=R−0(1−T+T2T3)The temperature coefficient of resistance of wire is 0.001259∘C−1. If resistance is 1Ω at 300 K, then 2Ω will be at:
A1400 KB1100 KC1127 JD1154 KThe temperature coefficient of resistance for a wire is 0.00125∘C−1. At 300 K its resistance is 1Ω. The temperature at which the resistance becomes 2Ω is
A450 KB1127 KC454 KD900 K
If resistors of resistance R1 and R2 are connected in parallel,then resultant resistance is "
View SolutionThe temperature coefficient of a resistance wire is 0.0012∘C−1. At 300 K, its resistance is 1Ω. At what temperature the resistance of the wire will be 2Ω ?
View SolutionThe temperature coefficient of resistance of the material of a wire is 0.00125∘C−1. Its resistance at 27∘C is 1Ω. At what temperature will its resistance be 2Ω ?
View SolutionThe temperature coefficient of resistance of a wire is 0.00145∘C−1. At 100∘C its resistance is 2Ω. At what temperature the resistance of the wire be 3Ω?
View SolutionWhen two resistors of resistances R1andR2 are connected in parallel, the net resistance is 3Ω. When connected in series, its value is 16Ω. Calculate the values of R1andR2.
View Solution
