The ratio of the time periods of small oscillation of the insulated spring and mass system before and after charging the masses is
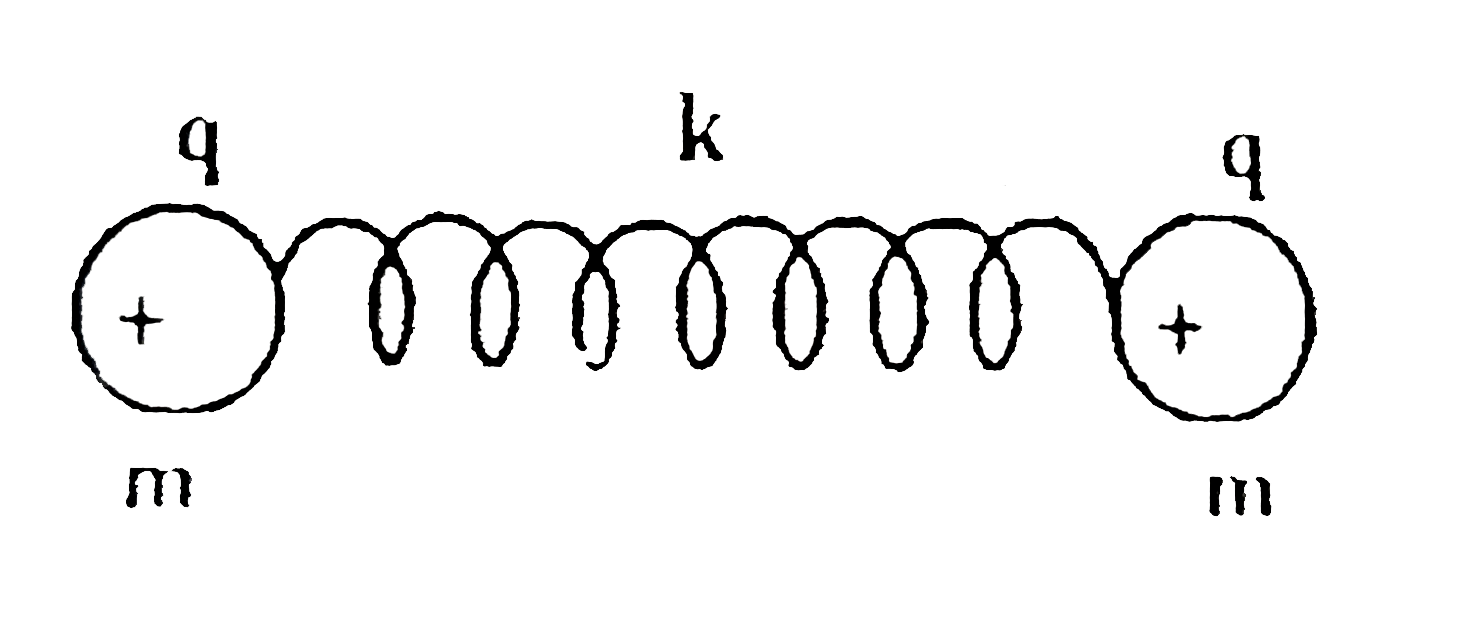
≥1
>1
≤1
=1
In equilibrium of the charged small bodies 14πε0q2(l0+x0)2=kx0 where x0 is the elongation in the spring in equilibrium.
Let a further small elongation of x is given to the spring.
Then net restoring force on any of the charged particle is given by,
F=−[k(x0+x)−14πε0q2(l0+x0+x)2]=−kx. Since x<<x0
⇒a=−2kmx As F=μa, where reduced mass μ=m×mm+m=m2
⇒a=−ω2x,
Hence ω=√2km⇒T=2π√m2k
In absence of charge, T0=2π√m2k
Therefore TT0=1
∴(D)
Similar Questions
The time period of small oscillations of mass m :-
_S01_040_Q01.png)
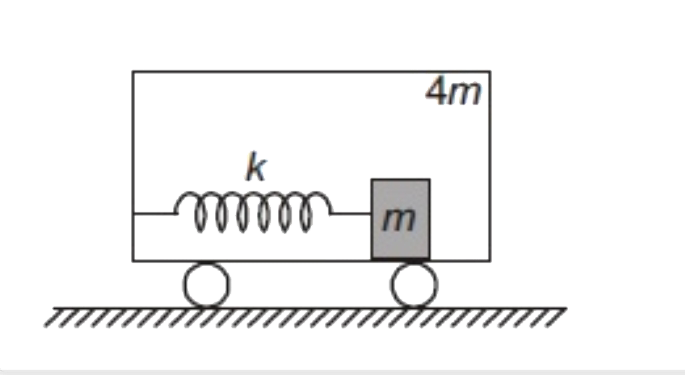
View SolutionA block of mass m is attached to a cart of mass 4m through spring of spring constant k as shown in the figure. Friction is absent everywhere. The time period of oscillations of the system, when spring is compressed and then released, is
View SolutionTime period of a given spring-mass system is

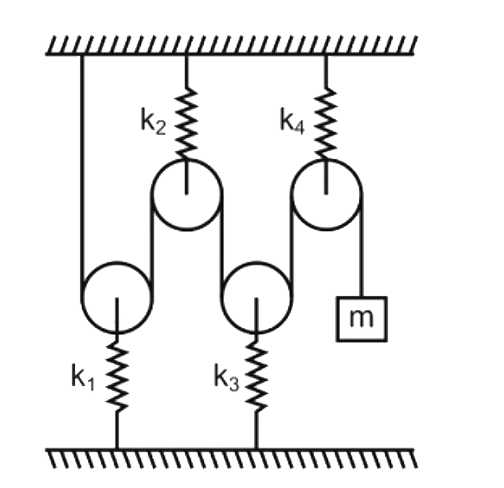
View SolutionIn the arrangement shown in the diagram, pulleys are small and springs are ideal. k1=k2=k3=k4=10Nm−1 are force constants of the springs and mass m=10kg. If the time period of small vertical oscillations of the block of mass m is given by 2πx seconds, then find the value of x.
View SolutionA spring is cut into two halves. What happens to its period of oscillation of a mass suspended from it, before cutting and after cutting ?
View SolutionFind the time period of oscillation of block of mass m. Spring, and pulley are ideal. Spring constant is k.

View Solution
A solid cylinder of mass M is attached to the spring by means of a frintionless pivot. The cylinder is placed on floor. Find the time period of small oscillation of the system, if the floor is sufficiently rought to prevent any slipping of cylinder. Assume stiffness of spring to be K.
View SolutionA solid uniform cylinder of mass m performs small oscillations due to the action of two springs of stiffness k each (figure). Find the period of these oscillation in the absence of sliding.
x
View SolutionIn the figure shown, mass of the plank is m and that of the solid cylinder is 8m. Springs are light. The plank is slightly displaced from equilibrium and then released. Find the period of small oscillations (in seconds) of the plank. There is no slipping at any contact point. The ratio of the mass of the plank adn stiffness of the spring i.e., mK=2π2
View SolutionA vertical spring-mass system with lower end of spring is fixed, made to undergo small oscillations. If the spring is stretched by 25cm, energy stored in the spring is 5J. Find the mass of the block if it makes 5 oscillations each second.
View Solution
