In the figure shown, mass of the plank is m and that of the solid cylinder is 8m. Springs are light. The plank is slightly displaced from equilibrium and then released. Find the period of small oscillations (in seconds) of the plank. There is no slipping at any contact point. The ratio of the mass of the plank adn stiffness of the spring i.e., mK=2π2
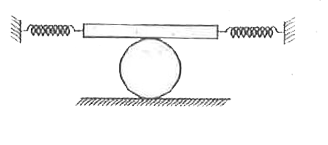
If the plank is displaced slightly by x towards left, then

2Kx−f1=ma2 …(i)
f1+f2=8ma1 …(ii)
τcm=lcmα
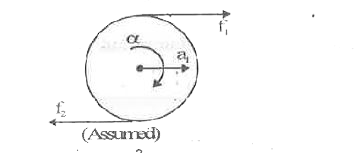
or (f1+f2)R=(8m)R22α
(f1+f2)=(8m)a12 ...(iii)
From (ii) and (iii),
2f1=(8m)×3a12
or f1=(8m)×3a14
and f2=−2ma1
Hence f2 will be in forward direction.
Also, for no slipping, at the point of contant between plank and cylinder
2a1=a2 ...(iv)
f1=(8m)×(3a28)=(3m)a2 ...(v)
from (i) and (v)
2Kx−(3m)a2=ma2
a2=2K4mx
or (−d2xdt2)=(2K4m)x
or d2xdt2=−(24×π22)x
orω2=π24
or ω=π2
or 2πT=π2
or T = 4 seconds.
Similar Questions
A plank of mass 'm' and area of cross - section A is floating as shown in figure. When slightly displaced from mean position, plank starts oscillations. Find time period of these oscillations.

View SolutionFigure shows a system consisting of a massless pulley, a spring of force constant k and a block of mass m. If the block is slightly displaced vertically down from is equilibrium position and then released, the period of its vertical oscillation is

View Solution
A solid cylinder of mass M is attached to the spring by means of a frintionless pivot. The cylinder is placed on floor. Find the time period of small oscillation of the system, if the floor is sufficiently rought to prevent any slipping of cylinder. Assume stiffness of spring to be K.
View SolutionA block of mass m hangs from three springs having same spring constant k. If the mass is slightly displaced downwards, the time period of oscillation will be

View SolutionA block of mass m hangs from a vertical spring of spring constant k. If it is displaced from its equilibrium position, find the time period of oscillations.
View SolutionA particle of mass m is attached with three springs A,B and C of equal force constancts k as shown in figure. The particle is pushed slightly against the spring C and released. Find the time period of oscillation.
 .
.
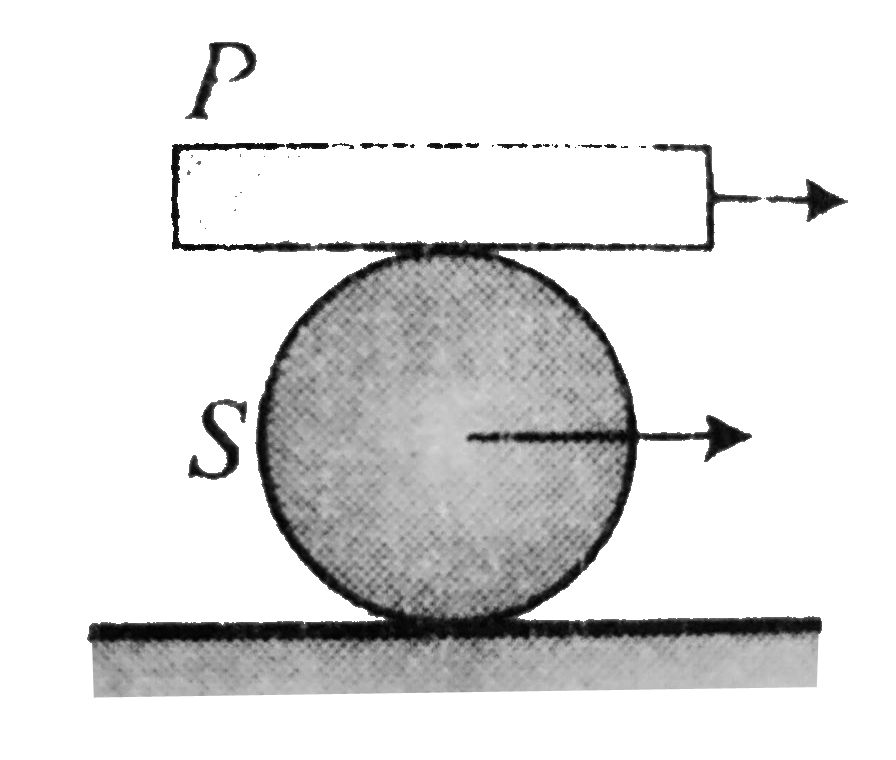
View SolutionA plank P is placed on a solid cylinder S, which rolls on a horizontal surface. The two are of equal mass. There is no slipping at any of the surfaces in contact. The ratio of kinetic energy of P to the kinetic energy of S is:
View SolutionA block of mass m is connected with two ideal pullies and a massless spring of spring constant K as shown in figure. The block is slightly displaced from its equilibrium position. If the time period of oscillation is μπ√mK. Then find the value of μ.

View SolutionA particle of mass m is attached with three springs A,B and C of equal force constancts k as shown in figure. The particle is pushed slightly against the spring C and released. Find the time period of oscillation.
 .
.
View SolutionA solid uniform cylinder of mass m performs small oscillations due to the action of two springs of stiffness k each (figure). Find the period of these oscillation in the absence of sliding.
x
View Solution