A spring is cut into two halves. What happens to its period of oscillation of a mass suspended from it, before cutting and after cutting ?
Before cutting let the period be T1 and after cutting T2 When it is cut into two equal parts the force constant of each part becomes 2k and hence new period T2=1√2T1
Related Playlists
- BOOK - ICSECHAPTER - OSCILLATIONS EXERCISE - LONG ANSWER QUESTIONS3videos
OSCILLATIONS
- BOOK - ICSECHAPTER - OSCILLATIONS EXERCISE - SHORT ANSWER QUESTIONS47videos
OSCILLATIONS
- BOOK - ICSECHAPTER - OSCILLATIONS EXERCISE - ADDITIONAL SOLVED PROBLEMS11videos
OSCILLATIONS
- BOOK - ICSECHAPTER - MOTION IN FLUIDS EXERCISE - SELECTED PROBLEMS (FROM POISEUILLE.S FORMULA)19videos
MOTION IN FLUIDS
- BOOK - ICSECHAPTER - PROPERTIES OF MATTEREXERCISE - MODULE 4 ( TEMPERATURE ) UNSOLVED PROBLEMS12videos
PROPERTIES OF MATTER
Similar Questions
Is oscillation of a mass suspended by a spring simple harmonic ?
View SolutionIf the period of oscillation of mass M suspended from a spring is one second, then the period of 9M will be
View SolutionIf the period of oscillation of mass M suspended. From a spring is one second, then the period of 4 M will be
View SolutionThe ratio of the time periods of small oscillation of the insulated spring and mass system before and after charging the masses is
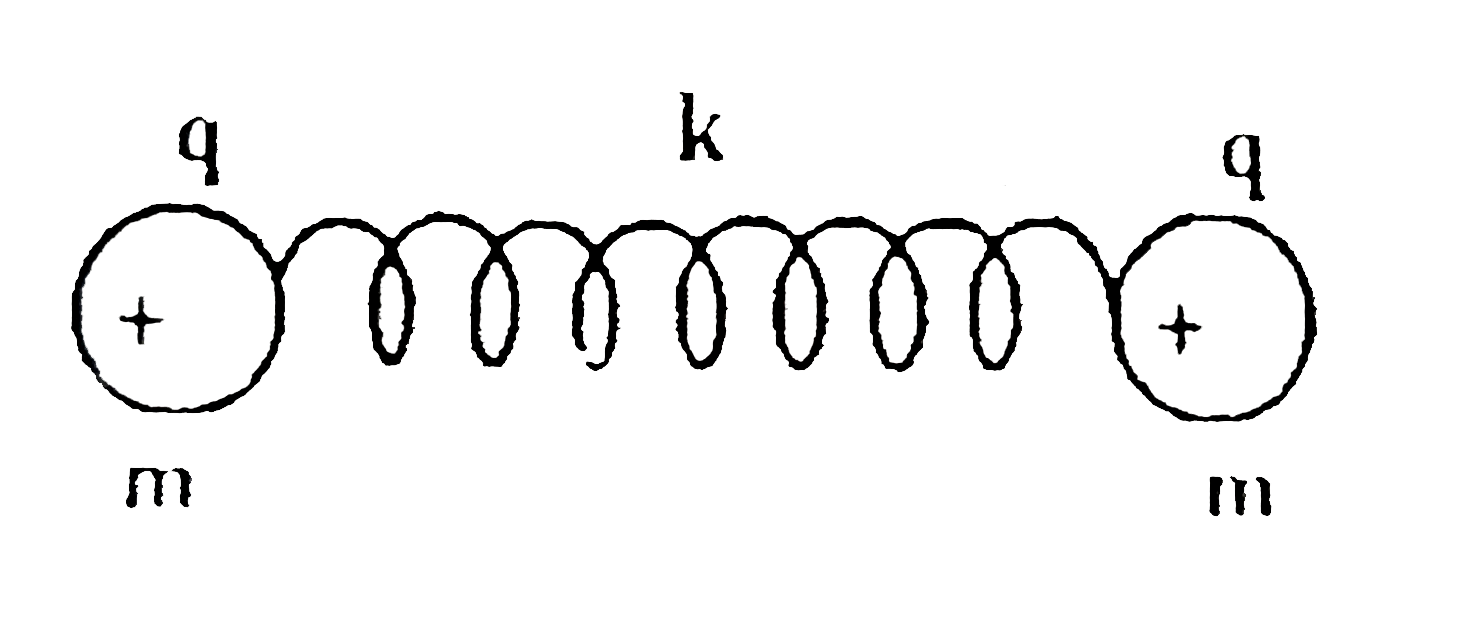 View Solution
View SolutionPeriodic time of oscillation T1 is obtained when a mass is suspended from a spring and if another spring is used with same mass then periodic time of oscillation is T2 . Now if this mass is suspended from series combination of above springs then calculated the time period.
View SolutionA mass m is suspended from a spring. Its frequency of oscillation is f. The spring is cut into two halves and the same mass is suspended from one of the pieces of the spring. The frequency of oscillation of the mass will be
View SolutionA spring has a certain mass suspended from it and its period for vertical oscillations is T1. The spring is now cut into two equal halves and the same mass is suspended from one of the half. The period of vertical oscillation is now T2. The ratio of T2/T1 is
View SolutionA weightless spring has a force constant k oscillates with frequency f when a mass m is suspended from it The spring is cut into three equal parts and a mass 3 m is suspended from it The frequency of oscillation of one part will now become
View SolutionAn object suspended from a spring exhibits oscillations of period T. Now the spring is cut in two halves and the same object is suspended with two halves as shown in figure. The new time period of oscillation will become
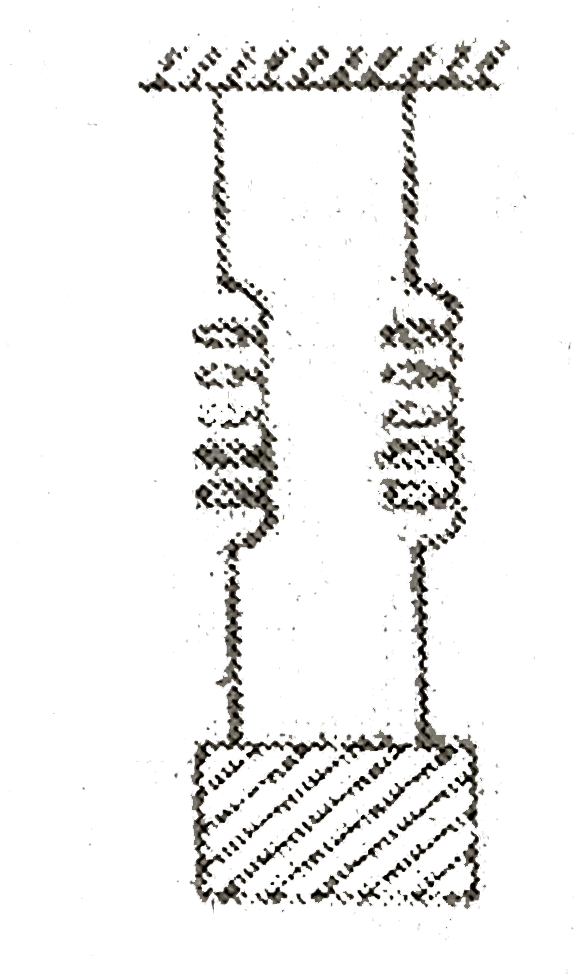 View Solution
View SolutionThe time period of a mass suspended from a spring is T. If the spring is cut into four equal parts and the same mass is suspended from one of the parts, then the new time period will be
View Solution


