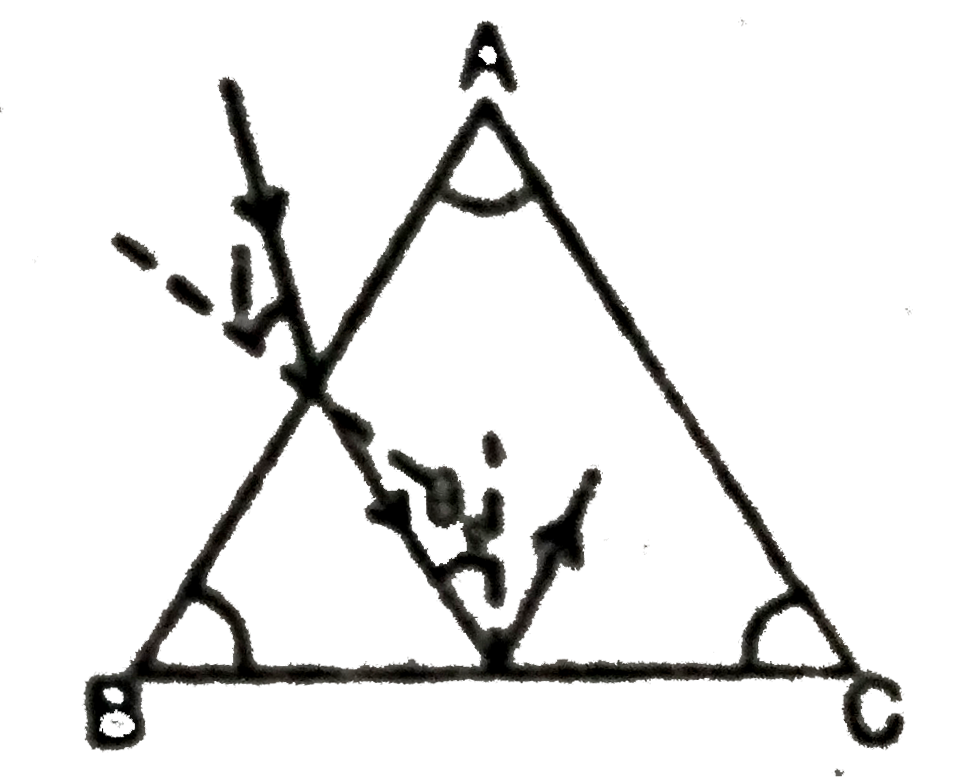
The figure shows the path of a ray passing through an equiangular prism PQR. It is incident on face PR at angle slightly greater than the critical angle for total internal reflection . If the angle α shown in the figure is 30∘ . Calculate the R.I. of the material of the prism.
_E01_145_Q01.png)
The correct Answer is:(73)1/2
_E01_145_S01.png)
For critical angle
μsin(90−r)=1.sin90
cosr=1μ
90−(120−r)=(r−30)
From snell's law
μsin(r−30∘)=1sin30∘
μ[sinrcos30∘−cos30∘−cosrsin30∘]=12
μ[√μ2−1μ×√32−1μ×12]=12
√u2−1×√32=1⇒μ2−1=43⇒μ(73)1/2
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE-04[B]
18 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE-05 (A)
35 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE-3
12 Videos
- CURRENT ELECTRICITYBOOK - ALLENCHAPTER - CURRENT ELECTRICITYEXERCISE - EX.II
66 Videos
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - EXERCISE 4
9 Videos
Similar Questions
The path of a ray of light passing through an equilateral glass prism ABC is shown in figure. The ray of light is incident on face BC at the critical angle for just total internal reflection. The total internal reflection. The total angle of deviation after the refraction at face AC is 108∘. Calculate the refractive index of the glass.
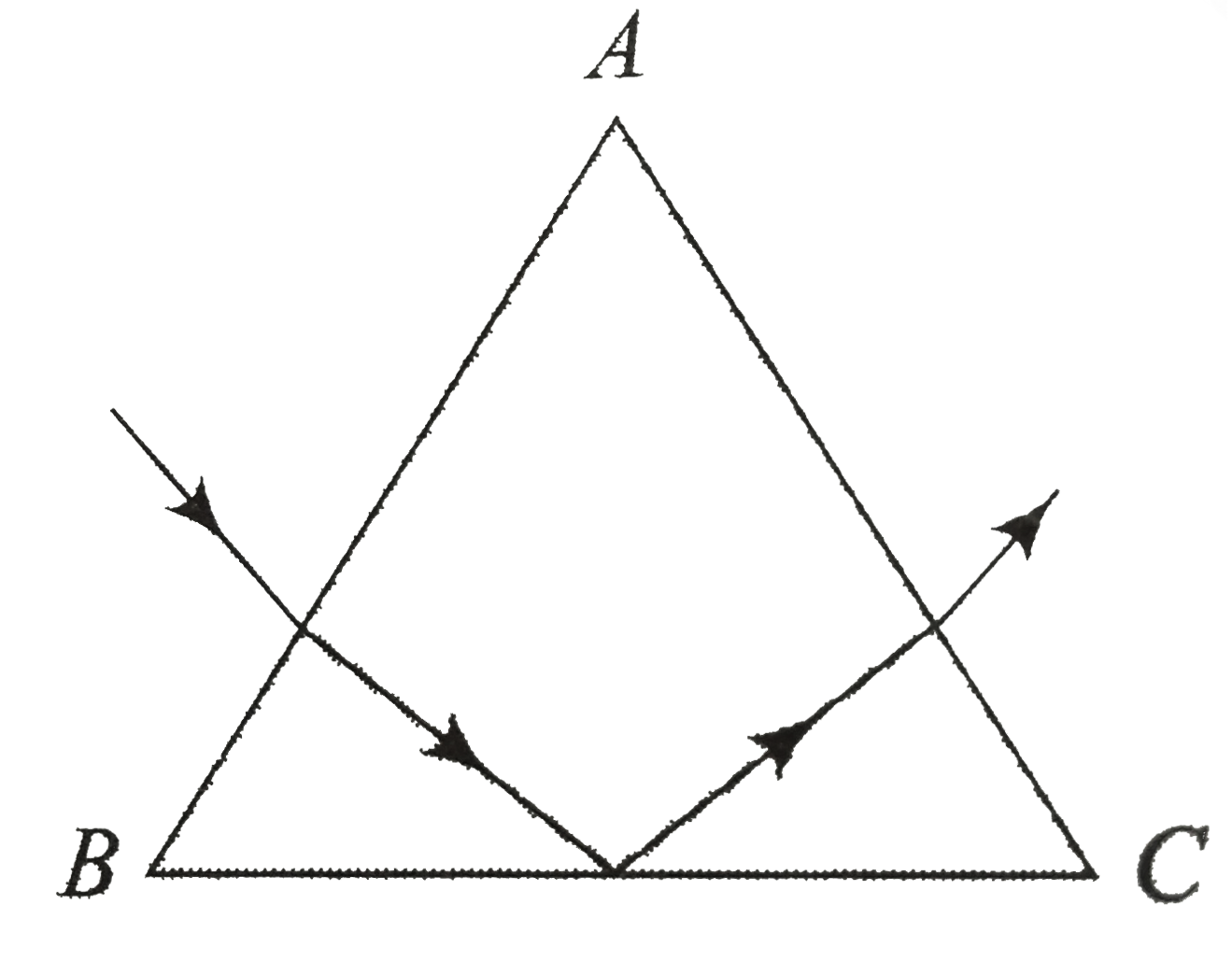
View Solution
A ray is incident on interface of two media at critical angle as shown in the figure.
View SolutionComplete the path of the ray AB through the glass prism in PQR till it emerges out of the prism. Given the critical angle of the glass as 42∘.
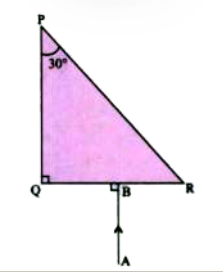
View SolutionA light is incident on face AB of an equilateral glass prism ABC. After refraction at AB, the ray is incident on face BC at the angle slightly greater than critical angle so that it gets reflected from face BC and finally emerges out from face AC. Net deviation angle of the ray is 112∘ anticlockwise. The angle of incidence 'i' has value:
View SolutionThe refractive index of a prism is μ. Find the maximum angle of the prism for which a ray incident on it will be transmitted through other face without total internal reflection.
View SolutionFor a ray of light refracted through a prism of angle 60∘, the angle of incidence is equal to the angle of emergence, each equal to 45∘. Find the refractive index of the material of the prism.
View SolutionA ray of monochromatic light is incident on one refracting face of a prism of angle 75∘. It passes thorugh the prism and is incident on the other face at the critical angle. If the refractive index of the material of the prism is √2, the angle of incidence on the first face of the prism is
View SolutionA ray of light incident normally on an isosceles right angled prism travels as shown in the figure. The least value of the refractive index of the prism must be
View SolutionA ray of light incident at an angle 90° with the face of an equilateral prism passes symmetrically. Calculate the refractive index of the material of the prism
View SolutionAt what angle should a ray of light be incident on the face of a prism of refracting angle 60∘ so that it just suffers total internal reflection at the other face ? The refractive index of the prism is 1.524.
View Solution
