Velocity of particle of mass 2kg varies with time t accoridng to the equation →v=(2tˆi+4ˆj)ms−1. Here t is in seconds. Find the impulse imparted to the particle in the time interval from t=0 to t=2s.
To find the impulse imparted to the particle in the time interval from t=0 to t=2 seconds, we can follow these steps:
Step 1: Determine the velocity at t=0 and t=2
The velocity of the particle is given by the equation:
→v=(2t^i+4^j)m/s
- For t=0:
→v(0)=2(0)^i+4^j=0^i+4^j=4^jm/s
- For t=2:
→v(2)=2(2)^i+4^j=4^i+4^jm/s
Step 2: Calculate the change in velocity (Δ→v)
The change in velocity is given by:
Δ→v=→v(2)−→v(0)
Substituting the values we found:
Δ→v=(4^i+4^j)−(0^i+4^j)=4^i+4^j−0^i−4^j=4^i
Step 3: Calculate the impulse
Impulse (→I) can also be calculated using the formula:
→I=mΔ→v
where m is the mass of the particle.
Given:
- Mass m=2kg
- Change in velocity Δ→v=4^i
Substituting the values:
→I=2kg⋅(4^i)=8^ikg m/s
Final Answer
The impulse imparted to the particle from t=0 to t=2 seconds is:
→I=8^iNs
---
→v=(2t^i+4^j)m/s
→v(0)=2(0)^i+4^j=0^i+4^j=4^jm/s
→v(2)=2(2)^i+4^j=4^i+4^jm/s
Δ→v=→v(2)−→v(0)
Substituting the values we found:
Δ→v=(4^i+4^j)−(0^i+4^j)=4^i+4^j−0^i−4^j=4^i
→I=mΔ→v
where m is the mass of the particle.
- Mass m=2kg
- Change in velocity Δ→v=4^i
→I=2kg⋅(4^i)=8^ikg m/s
The impulse imparted to the particle from t=0 to t=2 seconds is:
→I=8^iNs
Topper's Solved these Questions
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-I
52 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-II
112 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
- NEWTON'S LAWS OF MOTION & FRICTIONBOOK - ALLENCHAPTER - NEWTON'S LAWS OF MOTION & FRICTIONEXERCISE - EXERCISE (JA)
4 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)
24 Videos
Similar Questions
Acceleration of a particle in x-y plane varies with time as a=(2tˆi+3t2ˆj)m/s2 At time t=0, velocity of particle is 2m/s along positive x direction and particle starts from origin. Find velocity and coordinates of particle at t=1s.
View SolutionA particle starts from rest with a time varying acceleration a=(2t−4). Here t is in second and a in m/s2
The velocity time graph of the particle is
View SolutionA particle starts moving rectilinearly at time t=0 such that its velocity v changes with time t according to the equation v=t2−t, where t is in seconds and v in s−1. Find the time interval for which the particle retards.
View SolutionThe position of a particle is expressed as →r=(4t2ˆi+2tˆj) m, where t is time in second. Find the velocity o the particle at t = 3 s
View SolutionThe position of a particle varies with time according to the relation x=3t2+5t3+7t, where x is in m and t is in s. Find
(i) Displacement during time interval t = 1 s to t = 3 s.
(ii) Average velocity during time interval 0 - 5 s.
(iii) Instantaneous velocity at t = 0 and t = 5 s.
(iv) Average acceleration during time interval 0 - 5 s.
(v) Acceleration at t = 0 and t = 5 s.
View SolutionDisplacement of a particle of mass 2 kg varies with time as s=(2t2−2t+10)m. Find total work done on the particle in a time interval from t=0 to t=2s.
View SolutionDisplacement-time graph of a particle moving in a straight line is as shown in figure. Mass of the particle is 2 kg. The total impulse imparted to the particle in a time interval from t=0 to t=6 s in N-s will be
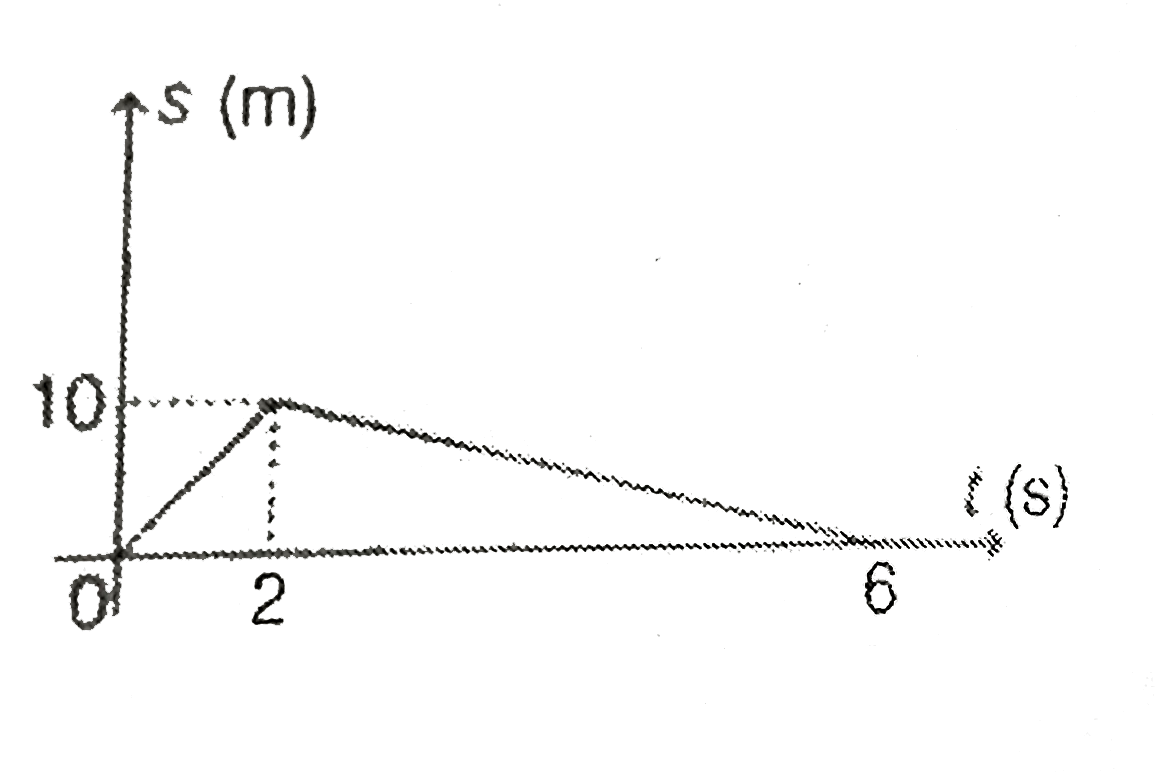
View SolutionA particle starts from rest with a time varying acceleration a=(2t−4). Here t is in second and a in m/s2
Maximum velocity of particle in negative direction is at t=…………… second
View SolutionA particle starts from rest with a time varying acceleration a=(2t−4). Here t is in second and a in m/s2
Particle comes to rest after a time t=………… second
View SolutionThe displacement of a body of mass 2kg varies with time t as S=t2+2t, where S is in seconds. The work done by all the forces acting on the body during the time interval t=2s to t=4s is
View Solution
