Question

Consider a non conduting ring of radius r and mass m and a particle of same mass, both at rest in free space. The particle is on the axis of the ring and far away from the ring. An amount Q of positive charge is uniformly distributed on the ring and the particle is given a positive charge q. The particle is imparted a velocity v towards the centre of the ring. Consider the consequences given in the columns and answer the following question

${\displaystyle \begin{array}{llllll}& \text{Column-I}& & \text{Column-II}& & \text{Column-III}\\ \left(I\right)& \text{Maximum speed of the ring is v}& \left(i\right)& \text{Minimum speed of the particle is}v/2& \left(P\right)& \text{Final speed of the ring and particle is v and zero respectively}.\\ \left(II\right)& \text{Maximum speed of the ring is}v/2& \left(ii\right)& \text{Minimum speed of the particle is zero}& \left(Q\right)& \text{Final speed of the ring and particle is zero and v respectively}.\\ \left(III\right)& \text{Maximum speed of the ring is}\frac{v}{2}[1+\sqrt{1-\frac{Qq}{\pi {\epsilon }_{0}mr{v}^2}}]& \left(iii\right)& \text{Minimum speed of the particle is}\frac{v}{2}[1+\sqrt{1-\frac{Qq}{\pi {\epsilon }_{0}mr{v}^2}}]& \left(R\right)& \text{Final speed of the ring and particle is v/4 and3v/4 respectively}.\\ \left(IV\right)& \text{Maximum speed of the ring is}\frac{v}{2}[1-\sqrt{1-\frac{Qq}{\pi {\epsilon }_{0}mr{v}^2}}]& \left(iv\right)& \text{Minimum speed of the particle is}\frac{v}{2}[1-\sqrt{1-\frac{Qq}{\pi {\epsilon }_{0}mr{v}^2}}]& \left(S\right)& \text{Final speed of the ring and particle is v/2 for both}.\end{array}}$
Which of following options is the correct is the representation if${\displaystyle v=\sqrt{\frac{Qq}{2\pi {\epsilon }_{0}mr}}}$

Options

A. `(II)(i)S`
B. `(I)(ii)P`
C. `(III)(iv)R`
D. `(IV)(iii)Q`

Answer

B

Explanation

Let v be the velocity of particle for which both moves together finally

`p_(i)=p_(f)`
`mv=2mv' implies v'=(v)/(2)" "….(i)`
`E_(i)=E_(f)`
`(1)/(2)mv^(2)((1)/(2)mv^('2))xx2-(kQq)/(r).....(ii)`
`v=sqrt((Qq)/(piepsilon_(0)mr))`
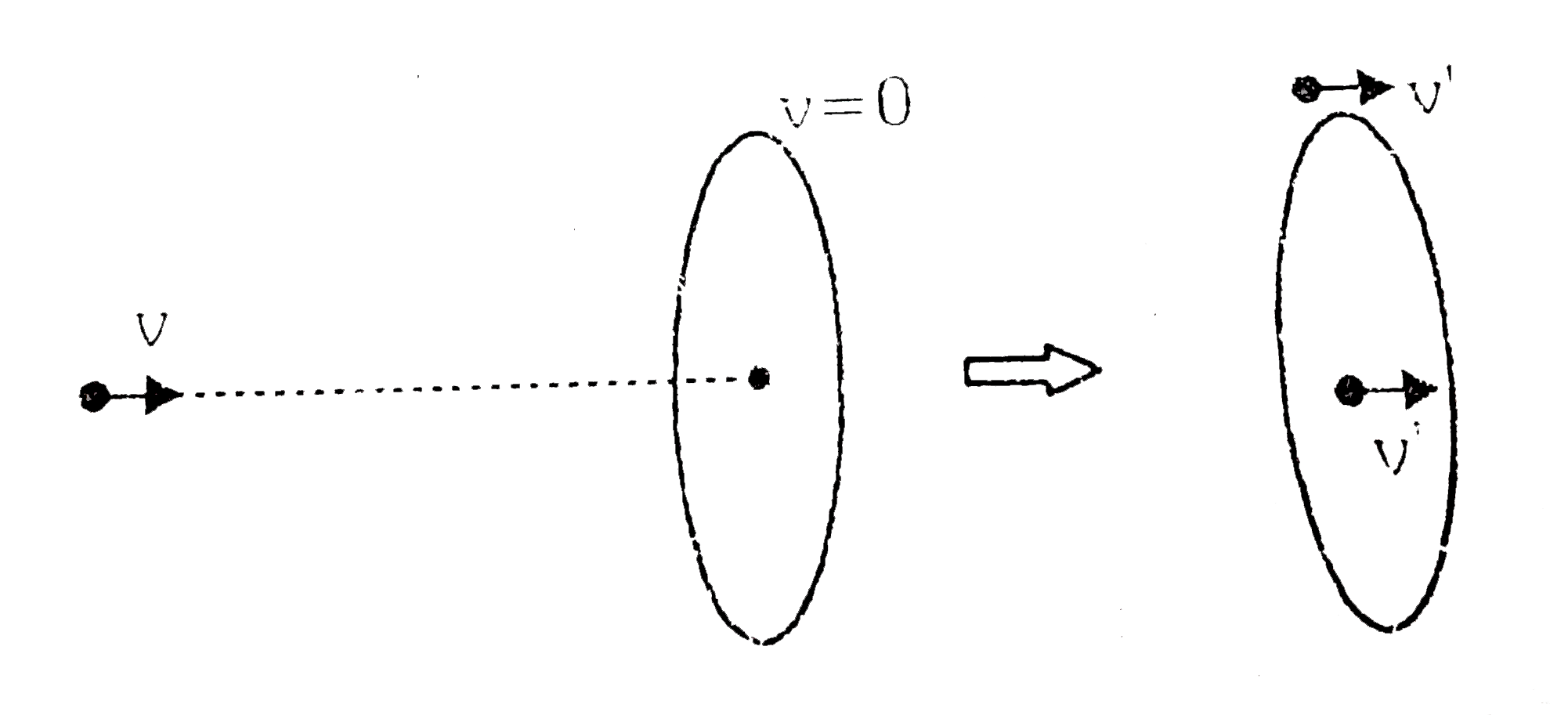
If `vltsqrt((Qq)/(piepsilon_(0)mr))` then velocity of particle continously decrease and that of ring continously increase. Final velocity of particle becomes zero and that of ring becomes v
If `vgtsqrt(Qq)/(piepsilon_(0)mr)` then particle crosses the ring and again it's velocity start increasing and finally becomes v and that of ring becomes zero.
`p_(i)=p_(f)impliesmv=mv_(1)+mv_(2)....(i)`
`(1)/(2)mv^(2)=(1)/(2)mv_(1)^(2)+(1)/(2)mv_(2)^(2)+(kQq)/(r)....(ii)`
Minimum speed of particle
`v_(1)=(v)/(2)[1+sqrt(1-(Qq)/(piepsilon_(0)mrv^(2))]]`

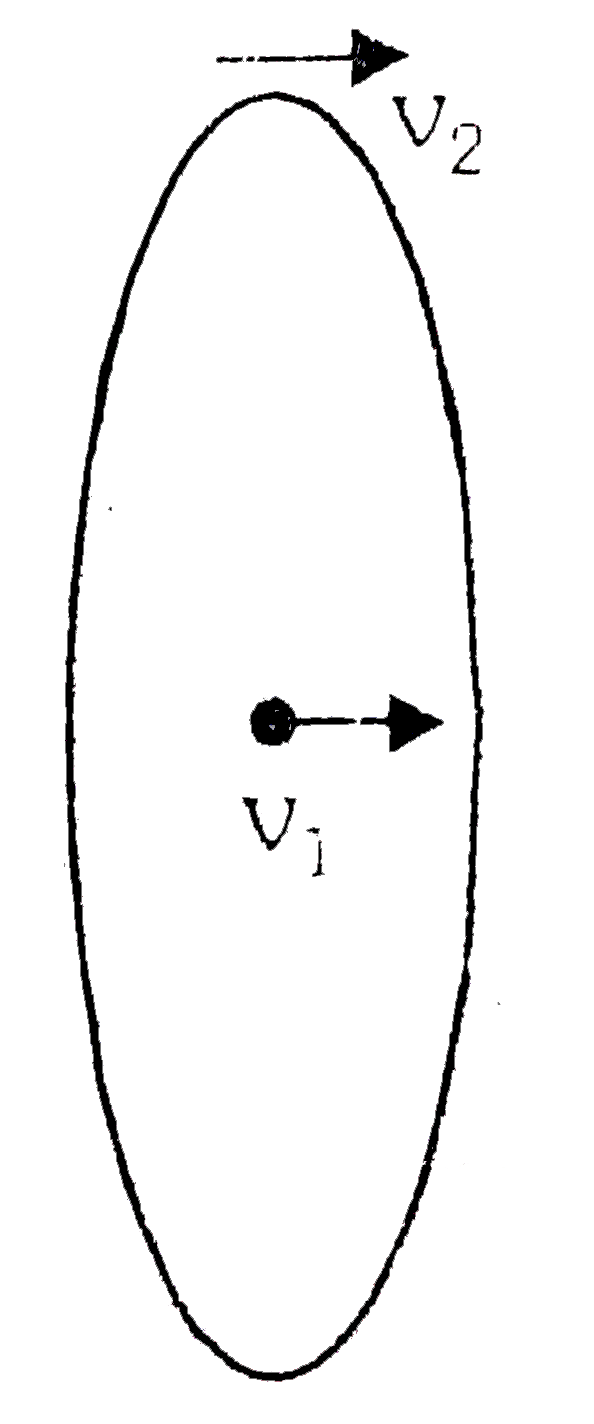
Maximum speed of ring
`v_(2)=(v)/(2)[1-sqrt(1-(Qq)/(piepsilon_(0)mrv^(2))]]`