Two smooth balls A and B, each of mass m and radius R, have their centre at (0,0,R) and (5R,-R,R) respectively, in a coordinate system as shown. Ball A, moving along positive x-axis, collides with ball B. Just before the collision, speed of ball A is 4m/s and ball B is stationary. The collision between the balls is elastic. Velocity of the ball A just after the collsion is
A. (ˆi+√3ˆj)m/s
B. (ˆi−√3ˆj)m/s
C. (2ˆi+√3ˆj)m/s
D. (2ˆi+2ˆj)m/s
(ˆi+√3ˆj)m/s
(ˆi−√3ˆj)m/s
(2ˆi+√3ˆj)m/s
(2ˆi+2ˆj)m/s
The correct Answer is:(A)
To solve the problem step by step, we will analyze the elastic collision between the two balls A and B.
Step 1: Understand the Initial Conditions
- Mass of both balls A and B = m
- Radius of both balls = R
- Position of ball A = (0, 0, R)
- Position of ball B = (5R, -R, R)
- Initial velocity of ball A = 4 m/s along the positive x-axis
- Initial velocity of ball B = 0 m/s (stationary)
Step 2: Determine the Direction of the Collision
Since ball A is moving along the positive x-axis and ball B is stationary, we need to find the direction of the collision. The line connecting the centers of the two balls at the moment of collision will determine the normal direction.
Step 3: Calculate the Normal and Tangential Components
1. Normal Component: This is the component of the velocity that acts along the line connecting the centers of the two balls.
2. Tangential Component: This is the component of the velocity that acts perpendicular to the normal component.
Step 4: Find the Angle of Collision
To find the angle of collision, we can calculate the direction vector from ball A to ball B:
- Vector from A to B = (5R - 0, -R - 0, R - R) = (5R, -R, 0)
The magnitude of this vector is:
Magnitude=√(5R)2+(−R)2=√25R2+R2=√26R2=R√26
Step 5: Calculate the Normal and Tangential Components of Velocity
The unit normal vector ^n from A to B is:
^n=(5R,−R,0)R√26=(5√26,−1√26,0)
The initial velocity of ball A is →vA=(4,0,0).
To find the normal component vAn:
vAn=→vA⋅^n=4⋅5√26+0⋅−1√26+0⋅0=20√26
Step 6: Apply Conservation of Momentum and Kinetic Energy
In an elastic collision, both momentum and kinetic energy are conserved. Since both balls have the same mass, the normal component of velocity of ball A after the collision will be equal to the normal component of ball B before the collision.
Step 7: Calculate the Final Velocity of Ball A
The tangential component of ball A remains unchanged. Therefore:
- Normal component after collision for ball A: v′An=0 (since ball B takes the normal component)
- Tangential component: vAt=4⋅−1√26
The final velocity of ball A can be expressed as:
→v′A=vAt^t+v′An^n
Where ^t is the tangential direction.
Step 8: Combine Components to Get Final Velocity
Using the components calculated, we can express the final velocity of ball A:
→v′A=(4⋅5√26)^i+(4⋅−1√26)^j
Final Answer
After simplifying, we find the final velocity of ball A:
→v′A=(2^i+√3^j) m/s
Conclusion
The correct answer is option A: (hati+√3hatj) m/s.
- Mass of both balls A and B = m
- Radius of both balls = R
- Position of ball A = (0, 0, R)
- Position of ball B = (5R, -R, R)
- Initial velocity of ball A = 4 m/s along the positive x-axis
- Initial velocity of ball B = 0 m/s (stationary)
Since ball A is moving along the positive x-axis and ball B is stationary, we need to find the direction of the collision. The line connecting the centers of the two balls at the moment of collision will determine the normal direction.
1. Normal Component: This is the component of the velocity that acts along the line connecting the centers of the two balls.
2. Tangential Component: This is the component of the velocity that acts perpendicular to the normal component.
To find the angle of collision, we can calculate the direction vector from ball A to ball B:
- Vector from A to B = (5R - 0, -R - 0, R - R) = (5R, -R, 0)
Magnitude=√(5R)2+(−R)2=√25R2+R2=√26R2=R√26
The unit normal vector ^n from A to B is:
^n=(5R,−R,0)R√26=(5√26,−1√26,0)
vAn=→vA⋅^n=4⋅5√26+0⋅−1√26+0⋅0=20√26
In an elastic collision, both momentum and kinetic energy are conserved. Since both balls have the same mass, the normal component of velocity of ball A after the collision will be equal to the normal component of ball B before the collision.
The tangential component of ball A remains unchanged. Therefore:
- Normal component after collision for ball A: v′An=0 (since ball B takes the normal component)
- Tangential component: vAt=4⋅−1√26
→v′A=vAt^t+v′An^n
Where ^t is the tangential direction.
Using the components calculated, we can express the final velocity of ball A:
→v′A=(4⋅5√26)^i+(4⋅−1√26)^j
After simplifying, we find the final velocity of ball A:
→v′A=(2^i+√3^j) m/s
The correct answer is option A: (hati+√3hatj) m/s.
Topper's Solved these Questions
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-I
40 Videos
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-II
43 Videos
- BASIC MATHEMATICS USED IN PHYSICS &VECTORS BOOK - ALLENCHAPTER - BASIC MATHEMATICS USED IN PHYSICS &VECTORS EXERCISE - EXERCISE-IV ASSERTION & REASON
11 Videos
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLENCHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 5 B (Integer Type Questions)
3 Videos
Similar Questions
Two smooth balls A and B, each of mass m and radius R, have their centers at (0, 0, R) and at (5R, -R, R) respectively, in a coordinate system as shown. Ball A, moving along positive x axis, collides with ball B. Just before the collision, speed of ball A is 4m/s and ball B is stationary. The collision between the ball is elastic.

Impulse of the force exerted by A and B during the collision, is equal to
View SolutionA glass ball collides with an identical ball at rest with v0=2 m/sec. If the coefficient of restitution of collision is e = 0.5, find the velocities of the glass balls just after the collision.
View Solution
Knowledge Check
Two identical balls A and B moving with velocities + 0.5 m/s and −0.3.m/s respectively collide head-on elastically. The velocities of the ball A and B after collision will be respectively
A+0.5m/sand+0.3m/sB−0.3m/sand+0.5m/sC + 0.3 m//s and + 0.5 m//sD−0.5m/sand+0.3m/s
A glass ball collides with a smooth horizontal surface (xz plane) with a velocity V=ai−bj. If the coefficient of restitution of collision be e, the velocity of the ball just after the collision will be
View SolutionA ball is moving with velocity 2m/s towards a heavy wall moving towards the ball with speed 1m/s as shown in figure. Assuming collision to be elastic, find the velocity of ball immediately after the collision.
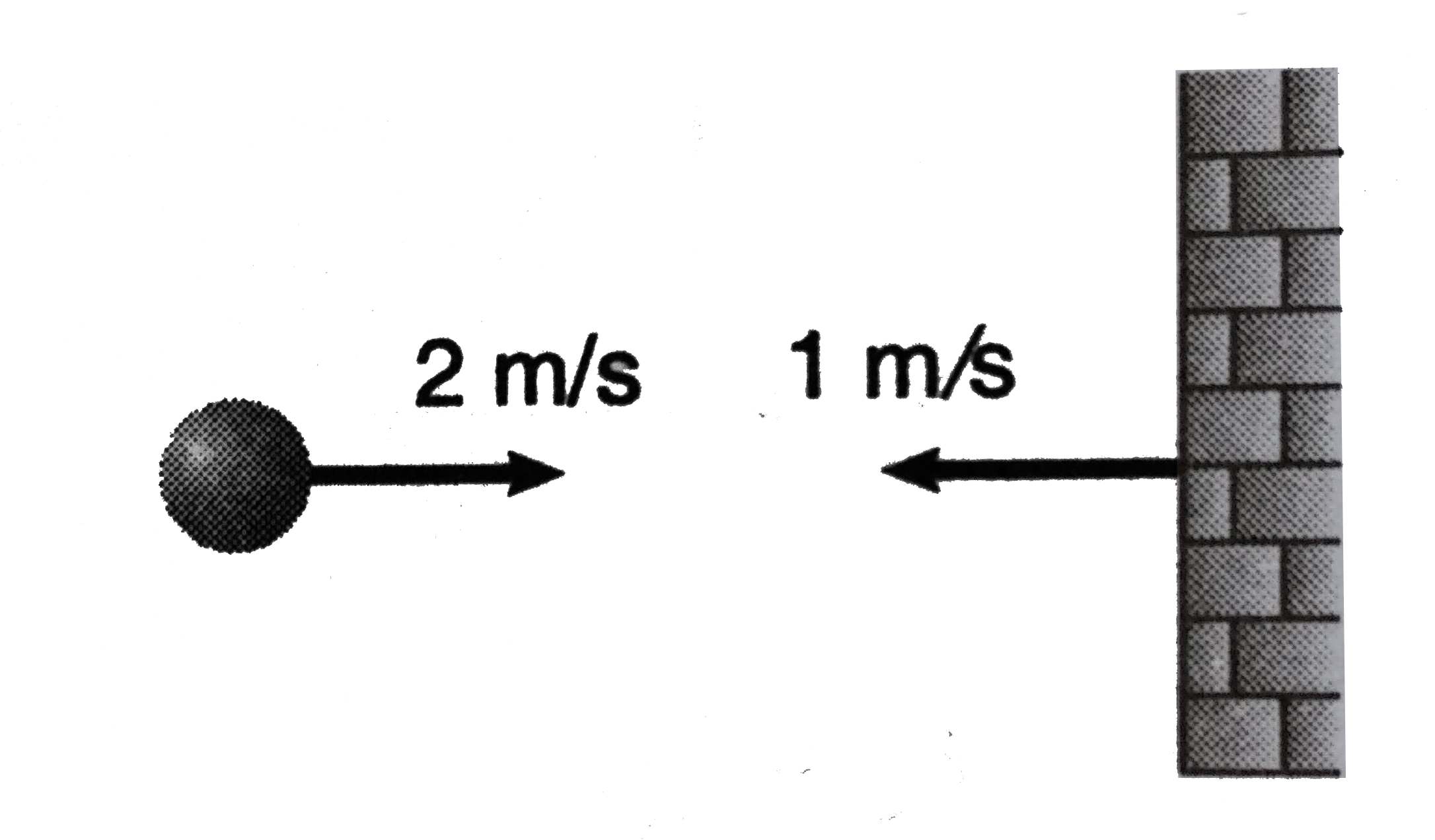
View SolutionA ball is moving with velocity 2m/s towards a heavy wall moving towards the ball with speed 1m/s as shown in figure. Assuming collision to be elastic, find the velocity of ball immediately after the collision.

View SolutionAn iron ball A collides with another stationary iron ball B If the ratio of radii of the balls is n = 2, find the ratio of their speeds just after the collision (e = 0.5)

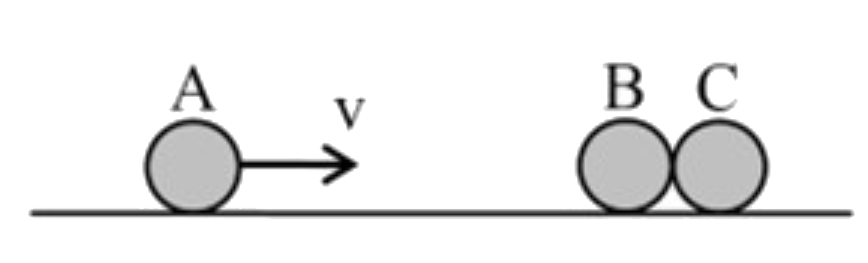
View SolutionThe figure below shows three identical balls A , B and C. Initially , the baiis B and C are at rest and the ball A while moving with a veiocity v, collides with ball B. if the collision is perfectiy elastic , then after the collision
View SolutionA ball A moving with momentum 2ˆi+4ˆj collides with identical ball B moving with momentum 6ˆj . After collision momentum of ball B is 10ˆj . Which of the following statement is correct?
View SolutionA ball A of mass M collides elastically with a similar ball B at rest as shown in figure. Initially velocity of ball A is u m/s. After collision,

View Solution
