A ball A moving with momentum 2ˆi+4ˆj collides with identical ball B moving with momentum 6ˆj . After collision momentum of ball B is 10ˆj . Which of the following statement is correct?
After collision momentum of A is 4ˆi
After collision momentum of A is parallel to momentum of B
After collision momentum of A is 2ˆi
If →PAand→PB are momenta of balls A and B, then Δ(∣∣→PA∣∣+∣∣→PB∣∣)=0
The correct Answer is:C
To solve the problem, we will analyze the momentum of both balls A and B before and after the collision, and then evaluate the given statements based on our calculations.
Step 1: Identify Initial Momenta
- For ball A, the initial momentum PAi=2^i+4^j.
- For ball B, the initial momentum PBi=6^j.
Step 2: Identify Final Momentum of Ball B
- After the collision, the momentum of ball B is given as PBf=10^j.
Step 3: Calculate Change in Momentum for Ball B
- The change in momentum for ball B can be calculated as:
ΔPB=PBf−PBi=10^j−6^j=4^j
- The magnitude of this change is:
|ΔPB|=4
Step 4: Apply Conservation of Momentum
According to the law of conservation of momentum, the total momentum before the collision should equal the total momentum after the collision. Thus, we have:
PAi+PBi=PAf+PBf
Substituting the known values:
(2^i+4^j)+(6^j)=PAf+(10^j)
This simplifies to:
2^i+10^j=PAf+10^j
Step 5: Solve for Final Momentum of Ball A
From the equation above, we can isolate PAf:
PAf=2^i+10^j−10^j=2^i
Step 6: Calculate Change in Momentum for Ball A
Now we can calculate the change in momentum for ball A:
ΔPA=PAf−PAi=(2^i)−(2^i+4^j)=−4^j
The magnitude of the change is:
|ΔPA|=4
Step 7: Evaluate the Statements
Now, we evaluate the statements provided in the question:
1. Statement A: After collision, momentum of A is 4^i - Incorrect (it is 2^i).
2. Statement B: After collision, momentum of A is parallel to momentum of B - Incorrect (they are perpendicular).
3. Statement C: After collision, momentum of A is 2^i - Correct.
4. Statement D: |ΔPA+ΔPB|=0 - Incorrect (the direction matters).
Conclusion
The only correct statement is C: After collision, momentum of A is 2^i.
---
- For ball A, the initial momentum PAi=2^i+4^j.
- For ball B, the initial momentum PBi=6^j.
- After the collision, the momentum of ball B is given as PBf=10^j.
- The change in momentum for ball B can be calculated as:
ΔPB=PBf−PBi=10^j−6^j=4^j
- The magnitude of this change is:
|ΔPB|=4
According to the law of conservation of momentum, the total momentum before the collision should equal the total momentum after the collision. Thus, we have:
PAi+PBi=PAf+PBf
Substituting the known values:
(2^i+4^j)+(6^j)=PAf+(10^j)
This simplifies to:
2^i+10^j=PAf+10^j
From the equation above, we can isolate PAf:
PAf=2^i+10^j−10^j=2^i
Now we can calculate the change in momentum for ball A:
ΔPA=PAf−PAi=(2^i)−(2^i+4^j)=−4^j
The magnitude of the change is:
|ΔPA|=4
Now, we evaluate the statements provided in the question:
1. Statement A: After collision, momentum of A is 4^i - Incorrect (it is 2^i).
2. Statement B: After collision, momentum of A is parallel to momentum of B - Incorrect (they are perpendicular).
3. Statement C: After collision, momentum of A is 2^i - Correct.
4. Statement D: |ΔPA+ΔPB|=0 - Incorrect (the direction matters).
The only correct statement is C: After collision, momentum of A is 2^i.
Topper's Solved these Questions
- ENERGY & MOMENTUMBOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - Level - 1 PARAGRAPH QUESTIONS
3 Videos
- ENERGY & MOMENTUMBOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - LEVEL - 2
48 Videos
- ENERGY & MOMENTUMBOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - LEVEL - 0 - LONG ANSWER TYPE
5 Videos
- ELECTROSTATICSBOOK - VMC MODULES ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - JEE Advanced (Archive)
89 Videos
- GASEOUS STATE & THERMODYNAMICSBOOK - VMC MODULES ENGLISHCHAPTER - GASEOUS STATE & THERMODYNAMICSEXERCISE - JEE ADVANCED (ARCHIVE )
111 Videos
Similar Questions
Given A=3ˆi+4ˆjandB=6ˆi+8ˆj which of the following statement is correct ?
View SolutionA ball of mass 1 kg moving with velocity →v=3ˆi+4ˆj collides with a wall and after collision velocity of the ball is −2ˆi+6ˆj . Which of the following unit vectors is perpendicular to wall?
View SolutionA ball with initial momentum P collides with rigid wall elastically. If P1 be it's momentum after collision then
View SolutionA ball of mass m moving with velocity v collides head on elastically with another identical ball moving with velocity - V. After collision
View SolutionA smooth ball 'A' moving with velocity 'V' collides with another smooth identical ball at rest. After collision both the balls move with same speed with angle between their velocities 60∘. No external force acts on the system of balls.

The speed of each ball after the collision is
View SolutionA smooth ball 'A' moving with velocity 'V' collides with another smooth identical ball at rest. After collision both the balls move with same speed with angle between their velocities 60∘. No external force acts on the system of balls.
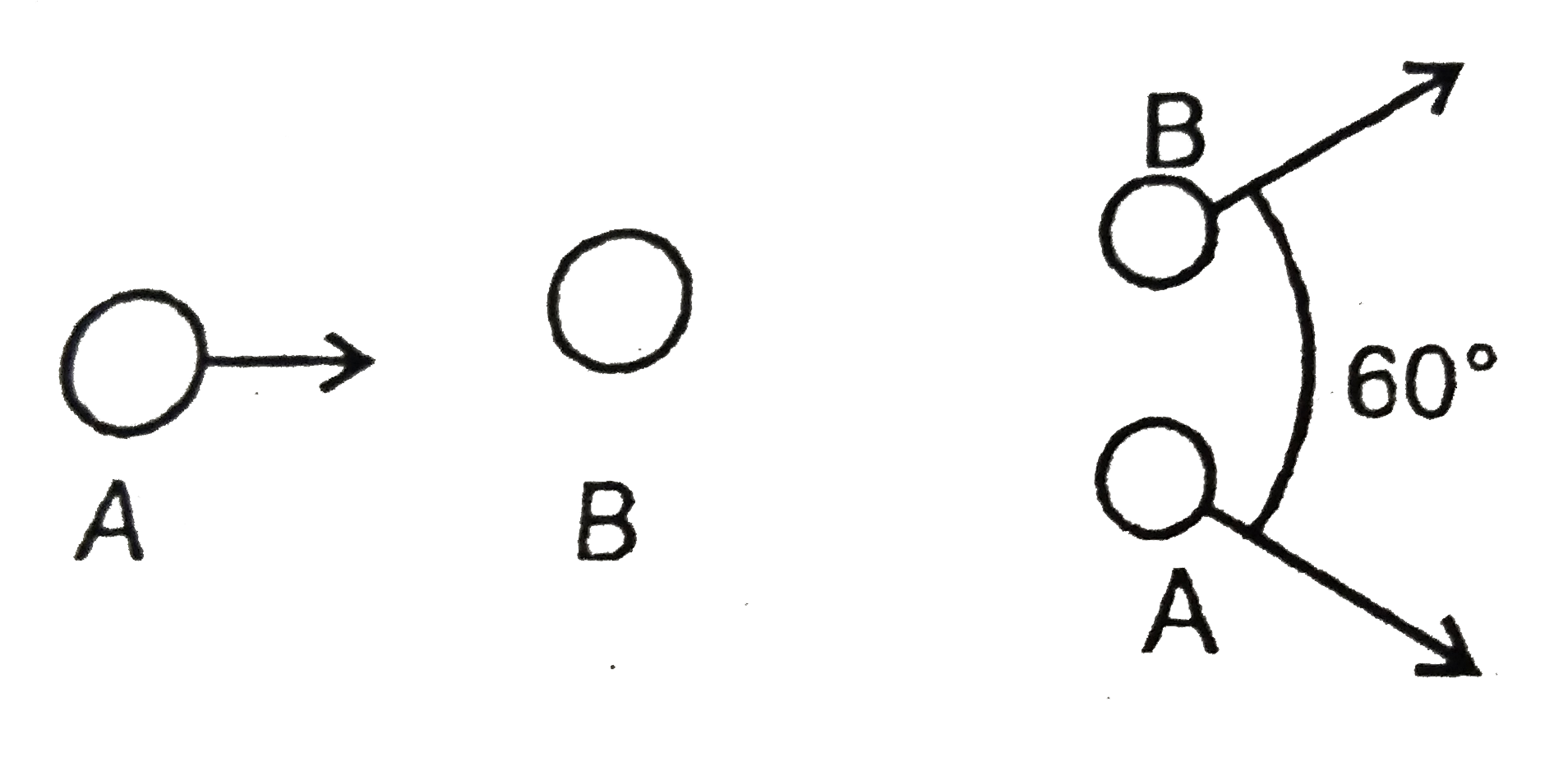
If the kinetic energy lost is fully converted to heat then heat produced is
View SolutionA smooth ball 'A' moving with velocity 'V' collides with another smooth identical ball at rest. After collision both the balls move with same speed with angle between their velocities 60∘. No external force acts on the system of balls.

The value of coefficient of resititution is
View SolutionA ball of mass m moving with velocity v, collide with the wall elastically as shown in the figure. After impact the change in angular momentum about P is :
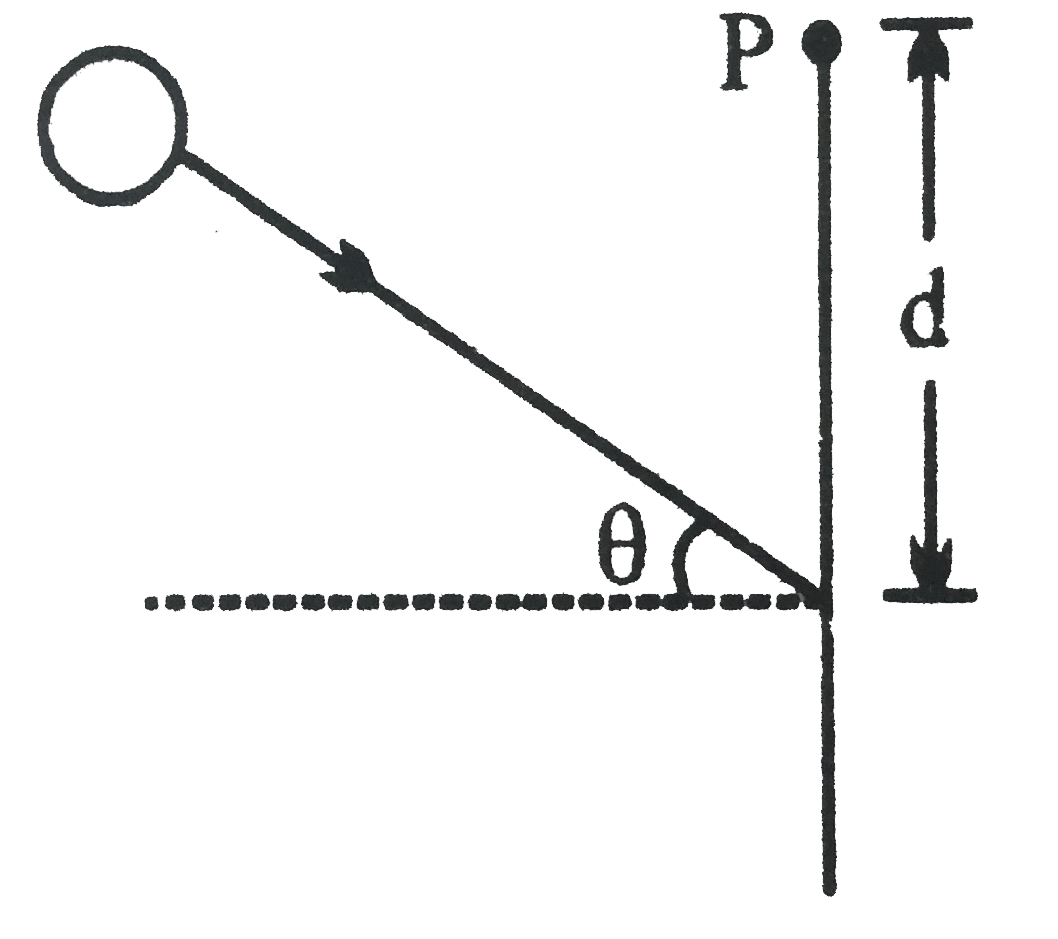 .
.
View SolutionTwo identical balls each moving with speed v at right angle to each other collide perfectly inelastically. Their speed after collision is
View SolutionTwo smooth balls A and B, each of mass m and radius R, have their centre at (0,0,R) and (5R,-R,R) respectively, in a coordinate system as shown. Ball A, moving along positive x-axis, collides with ball B. Just before the collision, speed of ball A is 4m/s and ball B is stationary. The collision between the balls is elastic. Velocity of the ball A just after the collsion is
A. (ˆi+√3ˆj)m/s
B. (ˆi−√3ˆj)m/s
C. (2ˆi+√3ˆj)m/s
D. (2ˆi+2ˆj)m/s
View Solution
