Define mechanical energy and law of conservation of mechanical energy. Prove conversion of gravitational potential energy in to kinetic energy by the help of law of conservation of mechanical energy. Also draw graph between energy and height.
Video Solution
Text Solution
Law of Conservation of Mechanical Energy : The total mechanical energy of a system (E) is defined as the sum of the kinetic energy (K) and the potential energy (U) of the system, i.e., E = K + U
According to the law of conservation of mechanical energy:
The total mechanical energy of a system remains constant if the only force that does work is a conservative force.
Let us consider a body of mass m placed at A as shown in
h = AB = height of the body above the ground
x = distance of any point C from A
g = acceleration due to gravity at the place
v1 = velocity of the body at C
v = velocity of the body at B, a point just above the ground. The velocity at the point A is zero.
At the point A

Potential energy at A, i.e., U1=mgh
Kinetic energy at A, i.e., K1=0
Total mechanical energy at A, i.e., E1=mgh+0=mgh…(1)
At the point C
As the body covers a distance x when it moves from A to C and v1 is the velocity at C, then from
v2−v20=2 we get : v21=0=2gx (As v=v1,v0=0,a=g,s=x) or v21=2gx
Kinetic energy at C, i.e., K2=12mv21=12m×2gx=mgx
Potential energy at C, i.e., U2=mg(h–x)
Total mechanical energy at C, i.e., E2=mgx+mg(h–x)=mgh…(2)
At the point B
From v2−v20=2as,v2−0=2gh(asv=v,v0=0,a=g,s=h)
or, v2=2gh
Kinetic energy at B, i.e., K3=12mv2=12m×2gh=mgh
Potential energy at B, i.e., U3=0
Total mechanical energy at B, i.e., E3=mgh+0=mgh…(3)
From eqns. (1), (2) and (3), E1=E2=E3
Clearly, total mechanical energy of the body at the points A, B and C (also at any other point) is the same. Thus, we find the total mechanical energy throughout the free fall is conserved. As the body falls down, the potential energy goes on decreasing whereas the kinetic energy goes on increasing. The variation of kinetic energy (K) and potential energy (U) and the constancy of the mechanical energy (E) have been represented graphically.
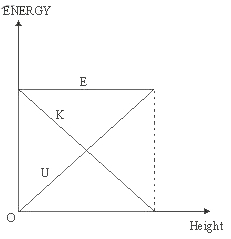 .
.
|
Topper's Solved these Questions
- ENERGY & MOMENTUMBOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - Level - 1
87 Videos
- ENERGY & MOMENTUMBOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - Level - 1 PARAGRAPH QUESTIONS
3 Videos
- ENERGY & MOMENTUMBOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - LEVEL - 0 -SHORT ANSWER TYPE
22 Videos
- ELECTROSTATICSBOOK - VMC MODULES ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - JEE Advanced (Archive)
89 Videos
- GASEOUS STATE & THERMODYNAMICSBOOK - VMC MODULES ENGLISHCHAPTER - GASEOUS STATE & THERMODYNAMICSEXERCISE - JEE ADVANCED (ARCHIVE )
111 Videos
Similar Questions
Knowledge Check
The usable form of mechanical energy is:
AElastic potential energyBKinetic energyCGravitational potential energyDNone of the given options.What are the two forms of mechanical energy?
APE and KEBSound and LightCHeat and tidalDSolar and wind
Define the law of conservation of energy.
View SolutionWhat is the law of conservation of energy?
View SolutionGive an example of mechanical energy
View SolutionFuels like coal and wood contain mechanical energy.
View SolutionState the principle of conservation of energy.
View SolutionDoes Lenz's law violate the law of conservation of energy?
View Solution
