Two small particles of equal masses start moving in opposite directions from a point A in a horizontal circular orbit. Their tangential velocities are v and 2, respectively. As shown in the figure. Between collisions, the particles moves with constant speeds. After making how many elastic collisions, other than that at A. these two particles will again reach the point A?

The correct Answer is:C
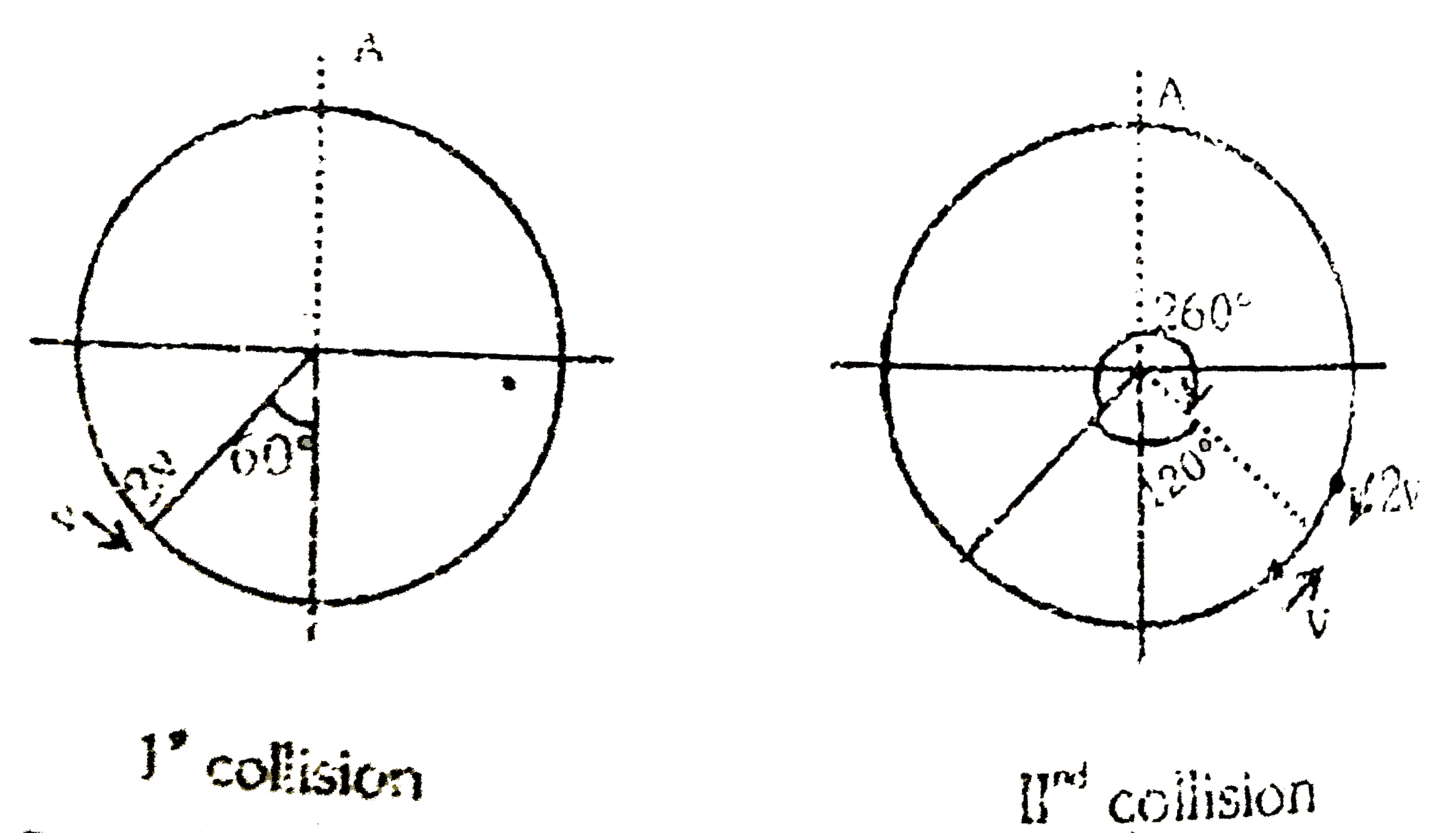
Particle with velocity 'v' covers and angle of 120∘ and after collision its velocity become '2v'.
It will cover angle of 240∘
Topper's Solved these Questions
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-V A
20 Videos
- BASIC MATHEMATICS USED IN PHYSICS &VECTORS BOOK - ALLENCHAPTER - BASIC MATHEMATICS USED IN PHYSICS &VECTORS EXERCISE - EXERCISE-IV ASSERTION & REASON
11 Videos
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLENCHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 5 B (Integer Type Questions)
3 Videos
Similar Questions
Two small particles of equal masses stant moving in opposite direction from a point A in a burtizonetal circule orbic their tangention velocity are V and 2V , respectively as shown in the figure between collsions , the particals move with constant speed After making how many elastic collition , other the then that at A these two partical will again reach the point A ?
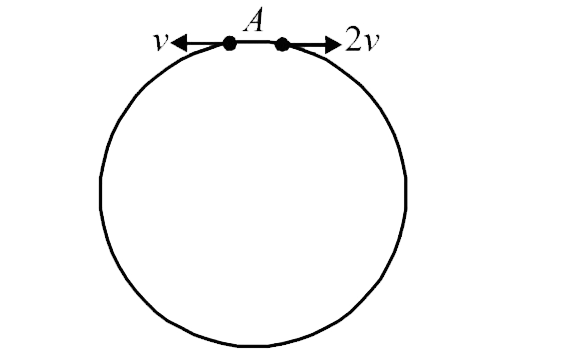
View SolutionTwo particles of masses m and 2m moving in opposite directions collide elastically with velocity 2v and v, respectiely. Find their velocities after collision.

View SolutionTwo balls of masses m and 2m moving in opposite directions collide head on elastically with velocities v and 2v. Find their velocities after collision.
View SolutionTwo balls of masses m and 2m moving in opposite directions collide head on elastically with velocities v and 2v. Find their velocities after collision.
View SolutionTwo particles move with constant velocities towards point O along straight lines in a plane as shown. The shortest distance A and B is

View SolutionTwo particles start moving simultaneously from points (0, 0) and (1, 0) respectively in the OXY plane with uniform velocities v1 and v2 as shown in the figure. It is found that they collide. Then

View SolutionTwo identical particles move towards each other with velocity 2v and v, respectively. The velocity of the centre of mass is:
View Solution(b) Two balls each of mass m moving in opposite direction with equal speed v collide head -on with each other . Predict the outcome of the collision assuming it perfectly elastic.
View SolutionA small particle of mass m is released from a height h on a large smooth sphere kept on a perfectly smooth surface as shown in the figure. Collision between particle and sphere is perfectly inelastic. Determine the velocities of particle and sphere after collision.
View SolutionA circle of radius unity is centered at thet origin. Two particles tart moving at the same time from the point (1,0) and move around the circle in opposite direction. One of the particle moves anticlockwise with constant speed v and the other moves clockwise with constant speed 3v. After leaving (1,0), the two particles meet first at a point P, and continue until they meet next at point Q. The coordinates of the point Q are
View Solution
