A one-dimensional force F varies with time according to the given graph. Calculate impulse of the force in following time intervals.
(a) From t=0s to t=10s.
From t=10s to t=15s.
(C) From t=0s to t=15s.
To solve the problem of calculating the impulse of the force over the specified time intervals based on the given graph, we will follow these steps:
1. Understanding Impulse:
Impulse is defined as the integral of force over time, which can also be interpreted as the area under the force vs. time graph. Mathematically, impulse J can be expressed as:
J=∫tftiFdt
where ti is the initial time and tf is the final time.
2. Calculate Impulse from t=0s to t=10s:
- Identify the area under the graph from t=0s to t=10s.
- The shape formed is a trapezoid with bases of lengths 10 N (at t=0s) and 5 N (at t=10s), and a height of 10s.
- The area (impulse) can be calculated as:
J1=Area=12×(b1+b2)×h=12×(10+5)×10=12×15×10=75N⋅s
3. Calculate Impulse from t=10s to t=15s:
- Identify the area under the graph from t=10s to t=15s.
- This area forms a triangle with a base of 5s and a height of 5 N.
- The area (impulse) can be calculated as:
J2=Area=12×base×height=12×5×5=12.5N⋅s
4. Calculate Impulse from t=0s to t=15s:
- For this interval, we sum the impulses calculated in the previous steps.
- The total impulse is:
Jtotal=J1+J2=75N⋅s−12.5N⋅s=62.5N⋅s
(Note: The area from t=10s to t=15s is negative since it is below the time axis.)
Final Answers:
- (a) Impulse from t=0s to t=10s: 75 N·s
- (b) Impulse from t=10s to t=15s: 12.5 N·s
- (c) Impulse from t=0s to t=15s: 62.5 N·s
Impulse is defined as the integral of force over time, which can also be interpreted as the area under the force vs. time graph. Mathematically, impulse J can be expressed as:
J=∫tftiFdt
where ti is the initial time and tf is the final time.
- Identify the area under the graph from t=0s to t=10s.
- The shape formed is a trapezoid with bases of lengths 10 N (at t=0s) and 5 N (at t=10s), and a height of 10s.
- The area (impulse) can be calculated as:
J1=Area=12×(b1+b2)×h=12×(10+5)×10=12×15×10=75N⋅s
- Identify the area under the graph from t=10s to t=15s.
- This area forms a triangle with a base of 5s and a height of 5 N.
- The area (impulse) can be calculated as:
J2=Area=12×base×height=12×5×5=12.5N⋅s
- For this interval, we sum the impulses calculated in the previous steps.
- The total impulse is:
Jtotal=J1+J2=75N⋅s−12.5N⋅s=62.5N⋅s
(Note: The area from t=10s to t=15s is negative since it is below the time axis.)
- (a) Impulse from t=0s to t=10s: 75 N·s
- (b) Impulse from t=10s to t=15s: 12.5 N·s
- (c) Impulse from t=0s to t=15s: 62.5 N·s
Topper's Solved these Questions
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-I
40 Videos
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-II
43 Videos
- BASIC MATHEMATICS USED IN PHYSICS &VECTORS BOOK - ALLENCHAPTER - BASIC MATHEMATICS USED IN PHYSICS &VECTORS EXERCISE - EXERCISE-IV ASSERTION & REASON
11 Videos
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLENCHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 5 B (Integer Type Questions)
3 Videos
Similar Questions
Calculate impulse of force →F=(3t2ˆi−(2t−1)ˆj+2ˆk)N over the time interval from t=1s to t=3s.
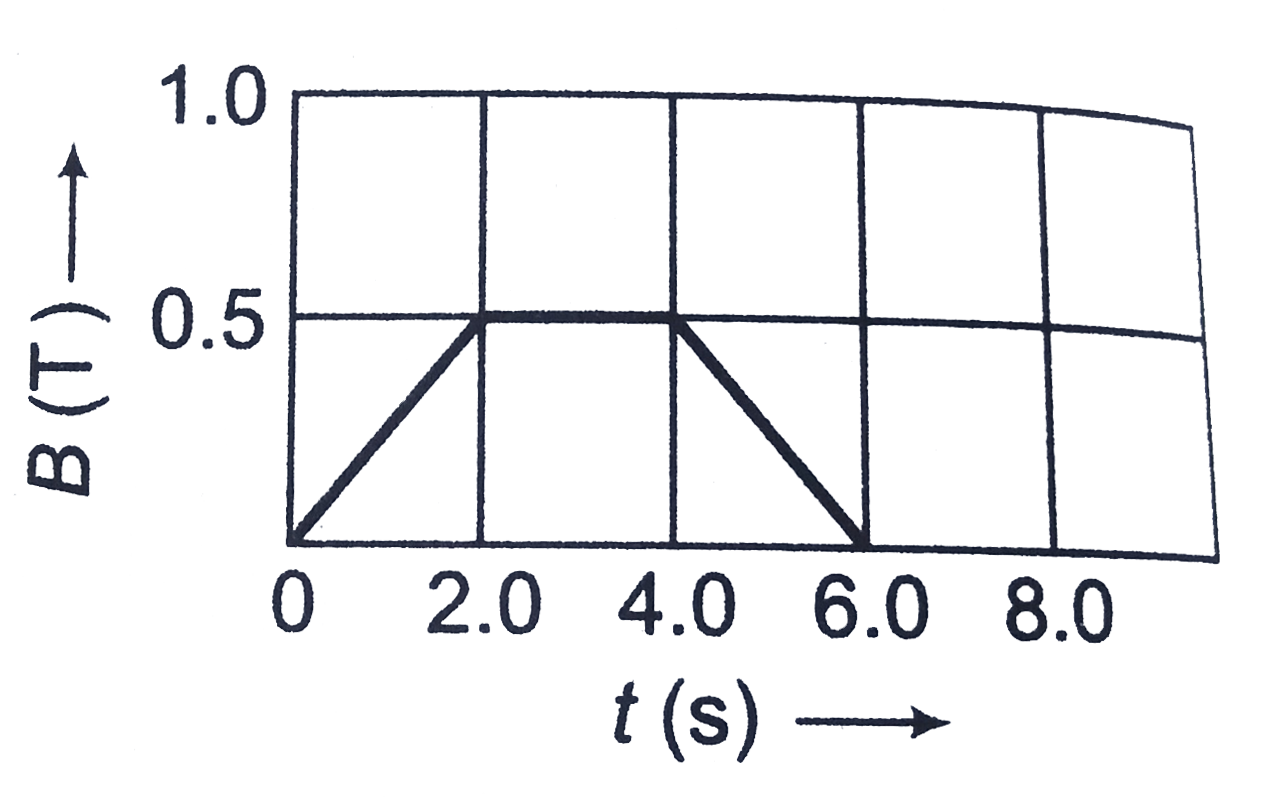
View SolutionThe magnetic field through a single loop of wire, 12cm in radius and of 8.5Ω resistance, changes with time as shown in figure. Calculate the emf in the loop as a function of time. Consider the time intervals
(a) t=0 to t=2.0s (b) t=2.0s to t=4.0s (c) t=4.0s to t=6.0s.
The magnetic field is perpendicular to the plane of the loop.
View SolutionDisplacement of a particle of mass 2 kg varies with time as s=(2t2−2t+10)m. Find total work done on the particle in a time interval from t=0 to t=2s.
View SolutionA particle moves according to the law x=rcos(pi)t/(2) The distance covered by it in the time interval between t=0 to t=3s is
View SolutionThe acceleration of particle varies with time as shown.

(a) Find an expression for velocity in terms of t.
(b) Calculate the displacement of the particle in the interval from t=2s to t=4s. Assume that v=0 at t=0.
View SolutionA particle is at x=+5 m at t=0,
x=-7m at t=6 s and x =+2 at t=10s.Find the averahe velocity of the particle during the intervals
(a)t=0 to t=6s
(b)t=6s to t=10s,
(c)t=0 to t=10 s.
View SolutionA particle moves rectilinearly possessing a parabolic s-t graph. Find the average velocity of the particle over a time interval from t=12s→t=1.5s.
View SolutionThe current in a wire varies with time according to the relation i = 2.0A+(0.6t2)A/s2. How many coulomb of charge pass a cross-section of the wire in the time interval between t = 0 and t = 10s?
View SolutionVelocity of particle of mass 2kg varies with time t accoridng to the equation →v=(2tˆi+4ˆj)ms−1. Here t is in seconds. Find the impulse imparted to the particle in the time interval from t=0 to t=2s.
View SolutionA force F=(2tˆi+3t2ˆj)N acts on an object moving in XY-plane. Find the magnitude of change in momentum of the object in the time interval t=0 to t=2s.
View Solution
