Question

Consider the inequality, $9^x-a{.3}^x-a+3\le 0$, where $\text{'}a\text{'}$ is a real parameter.
(a) Find the value of $\text{'}a\text{'}$ for which the inequality has at least one negative solution.
(b) Find the values of $\text{'}a\text{'}$ for which the inequality has at least one positive solution.
(c) Find the vlaues of $\text{'}a\text{'}$ for which the inequality has at least one real solution.

Explanation

`" "9^(x)-a. 3^(x)-a+3 le 0`
Let `" "t=3^(x)`
`rArr" "t^(2)-at-a+3 le 0`
or `" "t^(2)+3 le a(t+1)" "` (i)
where `" "t in R^(+) ` for `AA x in R`

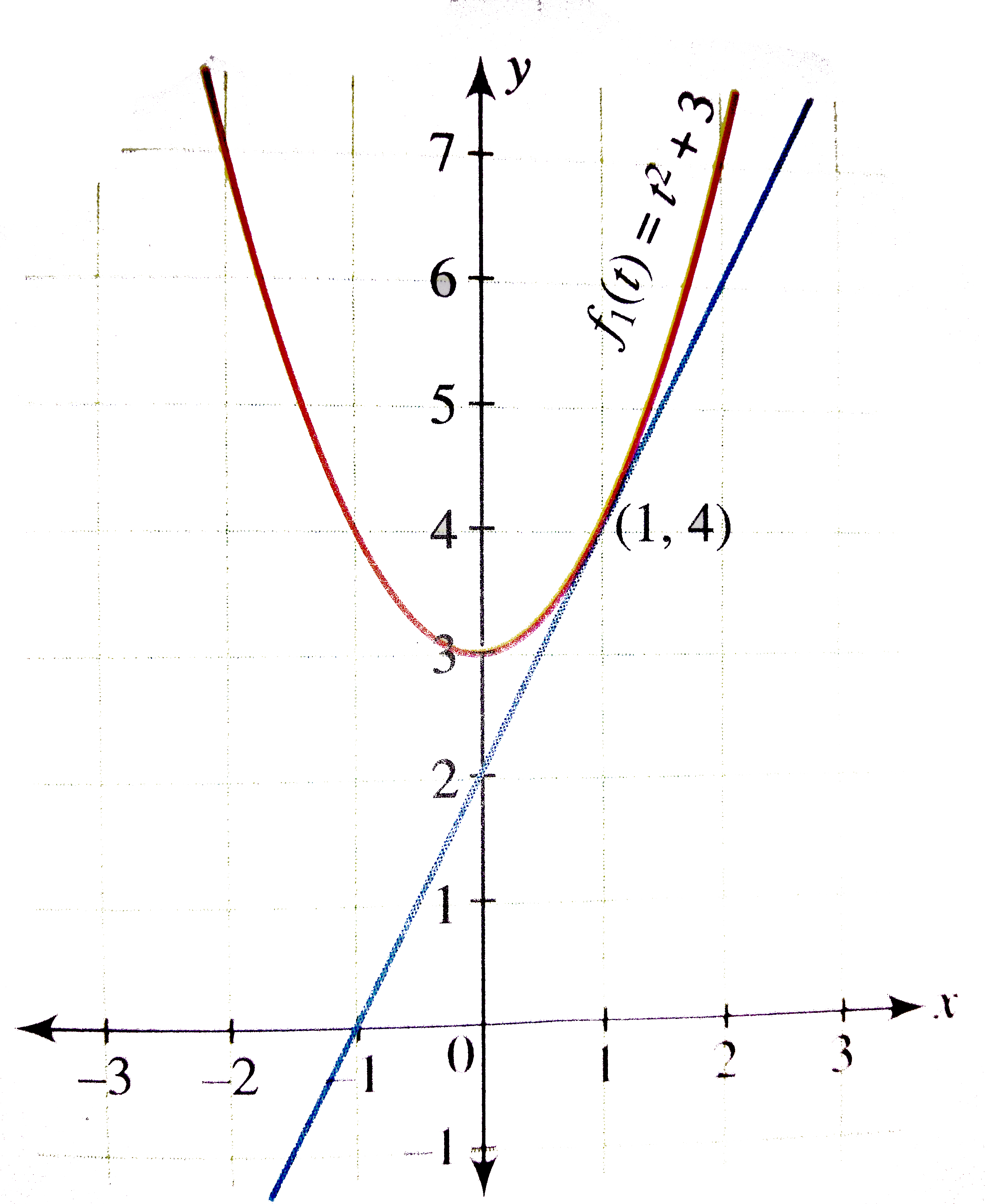
Let `f_(1)(t)=t^(2)+3 and f_(2)(t)= a (t+1)`
(a) For `x lt 0, t in (0, 1)`. That means (i) should have at least one solution in `t in (0, 1)`.
From (i), it is obvious that `a in R^(+)`.
Now `f_(2)(t)= a(t+1)` represents a straight line. It should meet the curve.
`f_(1)(t)=t^(2)+3`, at least once in `t in (0, 1)`.
`f_(1)(0)= 3, f_(1)(0) rArr a=3, "if" f_(1)(1)= f_(2)(1)=a =2`
Hence required `a in (2, 3)`.
(b) For at least one positive solution, `t in (1, oo)`. That means the graphs of `f_(1)(t)=t^(2)+3 and f_(2)(t)= a(t+1)` should meet at least once in `t in (1, oo)`.
If `a=2`, both curves touch each other at `(1, 4)`.
Hence required `a in (2, oo)`.
(c) In this case, both graphs should meet at least once in `t in (0, oo)`.
For `a=2`, both curves touch, hence required `a in [2, oo)`.