Consider the inequation $x^{2} + x + a - 9 < 0$
The values of the real parameter a so that the given inequaiton has at least one positive solution:

A

$(- \infty, 37 / 4)$

B

$(- \infty, \infty)$

C

$(3, \infty)$

D

$(- \infty, 9)$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:4

Let $f (x) = x^{2} + x + a - 9$
$x^{2} + x + a - 9 > 0$ has at least one positve solution, the either both the roots of equation $x^{2} + x + a - 9 = 0$ are non-negative or 0 lies betweens the roots.

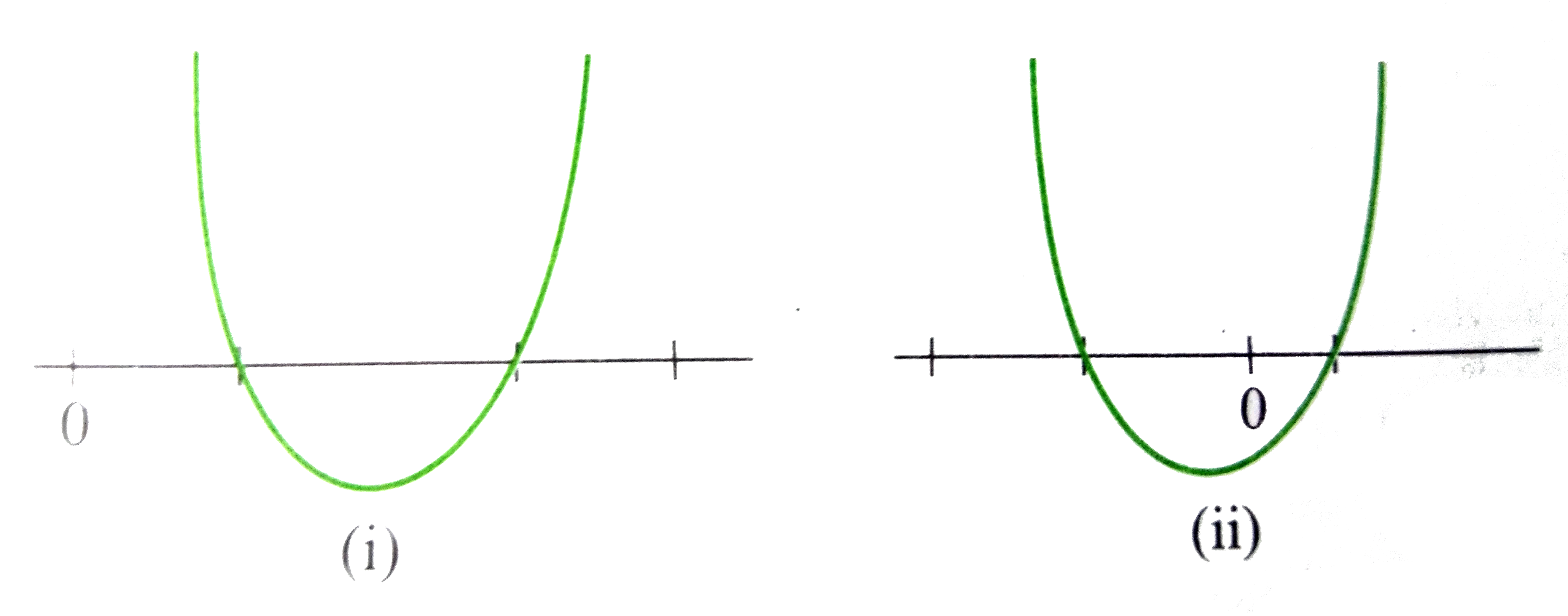
Now sum of roots $= - \frac{1}{2}$ , hence, case I is not possible . For Case II, $f (0) < 0 \Rightarrow a - 9 < 0 or a < 9$

|

Updated on:21/07/2023

Class 12 MATHS QUADRATIC EQUATIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Consider the inequation $x^{2} + x + a - 9 < 0$
The values of the real parameter a so that the given inequations has at least one negative solution.
A $(- \infty, 9)$
B $(\frac{37}{4}, \infty)$
C $(- \infty, \frac{37}{4})$
Dnone of these
Question 2 - Select One
Consider the inequation $x^{2} + x + a - 9 < 0$
The value of the parameter a so that the given inequaiton is ture $\forall x \in (- 1, 3)$
A $(- \infty, - 3]$
B $(- 3, \infty)$
C $[9, \infty)$
D $(- \infty, 34 / 4)$
Question 3 - Select One
Consider the inequation $9^{x} - a 3^{x} - a + 3 \leq 0$ , where a is real parameter.
The given inequality has at least one negative soluiton for $a \in$
A $(- \infty, 2)$
B $(3, \infty)$
C $(- 2, \infty)$
D $(2, 3)$

CENGAGE-THEORY OF EQUATIONS-Exercise (Comprehension)

Home
Ask Doubt
Profile